bài này hơi khó chút,vẫn lm đc bth nhaaaa
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NV
Nguyễn Việt Lâm
Giáo viên
1 tháng 7 2021
\(B=\left(x-1\right)\left(x+6\right)\left(x+2\right)\left(x+3\right)\)
\(=\left(x^2+5x-6\right)\left(x^2+5x+6\right)\)
\(=\left(x^2+5x\right)^2-36\ge-36\)
\(B_{min}=-36\) khi \(\left[{}\begin{matrix}x=0\\x=-5\end{matrix}\right.\)
\(C=\left(x^2-2x+1\right)+\left(y^2-4y+4\right)+2\)
\(=\left(x-1\right)^2+\left(y-2\right)^2+2\ge2\)
\(C_{min}=2\) khi \(\left(x;y\right)=\left(1;2\right)\)
1 tháng 7 2021
b) Ta có: \(B=\left(x-1\right)\left(x+2\right)\left(x+3\right)\left(x+6\right)\)
\(=\left(x^2+5x-6\right)\left(x^2+5x+6\right)\)
\(=\left(x^2+5x\right)^2-36\ge-36\forall x\)
Dấu '=' xảy ra khi x(x+5)=0
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-5\end{matrix}\right.\)
Vậy: \(B_{min}=-36\) khi \(x\in\left\{0;-5\right\}\)
c) Ta có: \(C=x^2-2x+y^2-4y+7\)
\(=x^2-2x+1+y^2-4y+4+2\)
\(=\left(x-1\right)^2+\left(y-2\right)^2+2\ge2\forall x,y\)
Dấu '=' xảy ra khi \(\left\{{}\begin{matrix}x=1\\y=2\end{matrix}\right.\)
Vậy: \(C_{min}=2\) khi (x,y)=(1;2)
MH
4
DT
Đỗ Thanh Hải
CTVVIP
14 tháng 4 2021
Tờ 1
41 It's very important to use body language in communication
42 Despite her age, she still leads an active life
43 My mother said that you had to decorate the room carefully
44 People recycle old cans to make new ones
45 Tim is always forgetting his homework
46 T
47 F
48 T
49 T
50 F
DT
Đỗ Thanh Hải
CTVVIP
14 tháng 4 2021
Tờ 2
17 C => hard
18 do => make
19 D => has
20 to go => going
21 A => At
22 B => to
23 C => beautifully
24 D => five-star
25 is => was
V
26 would travel
27 be
28 to buy
29 has spoken
30 Has - just been finished
VI
31 for
32 as
33 about
34 with
35 than
VII
36 development
37 exploration
38 behavior
39 deforestation
40 specialness
1 tháng 10 2017
\(2x^2+2y^2-4xy+2x-2y+4\)
\(=2\left(x-y\right)^2+2\left(x-y\right)+4\)
\(=2\left[\left(x-y\right)^2+2\left(x-y\right)\frac{1}{2}+\frac{1}{4}\right]+\frac{7}{2}\)
\(=2\left(x-y+\frac{1}{2}\right)^2+\frac{7}{2}\)
\(\Rightarrow A\ge\frac{7}{2}\)
Dấu = bn tự tính nhé
4 tháng 7 2016
mới giải đucợ 1 vế nè. xem tạm nhé
đặt cái biểu thức là S đi ^^
ta có:
\(\frac{1}{\left(n+1\right)\sqrt{n}}=\frac{\sqrt{n}}{\left(n+1\right)n}=\sqrt{n}.\frac{1}{n\left(n+1\right)}
=\sqrt{n}\left(\frac{1}{\sqrt{n}}+\frac{1}{\sqrt{n+1}}\right)
.\left(\frac{1}{\sqrt{n}}-\frac{1}{\sqrt{n+1}}\right)\)
< \(\sqrt{n}.\left(\frac{1}{\sqrt{n}}+\frac{1}{\sqrt{n}}\right).\left(\frac{1}{\sqrt{n}}-\frac{1}{\sqrt{n+1}}\right)\)
=\(\sqrt{n}.\frac{2}{\sqrt{n}}.\left(\frac{1}{\sqrt{n}}-\frac{1}{\sqrt{n+1}}\right)=2.\left(\frac{1}{\sqrt{n}}-\frac{1}{\sqrt{n+1}}\right)=\frac{2}{\sqrt{n}}-\frac{2}{\sqrt{n+1}}\)
áp dụng ta được: \(\frac{1}{2\sqrt{1}}< \frac{2}{\sqrt{1}}-\frac{2}{\sqrt{2}}\)
\(\frac{1}{3\sqrt{2}}< \frac{2}{\sqrt{2}}-\frac{2}{\sqrt{2}}\)
...................................................
\(\frac{1}{2011\sqrt{2010}}< \frac{2}{\sqrt{2010}}-\frac{2}{\sqrt{2011}}\)
=> \(S< 2-\frac{2}{\sqrt{2011}}< \frac{88}{45}\)
còn một vế nữa để mai nhé ^^ giờ mình bận :P hì
4 tháng 7 2016
mình bị ấn sai r :3 \(\frac{1}{3\sqrt{2}}< \frac{2}{\sqrt{2}}-\frac{2}{\sqrt{3}}\)đó nhá.sr nha ^^
VS
4
LM
7
29 tháng 5 2017
\(x\)x ( 2+3 ) - 45 = 2005
\(x\)x 5 = 2005 + 45
\(x\)x 5 = 2050
\(x\) = 2050 : 5
\(x=410\)
29 tháng 5 2017
2*x-45+3*x=2005
x2*x+3*x-45=2005
x(2+3) =2005+45
x.5 =2050
x =410
TM
2
GN
GV Nguyễn Trần Thành Đạt
Giáo viên
3 tháng 8 2023
Bài 9A:
\(a,\left(x+5\right)^2-\left(x-5\right)^2-2x+1=0\\ \Leftrightarrow\left(x^2+10x+25\right)-\left(x^2-10x+25\right)-2x+1=0\\ \Leftrightarrow x^2-x^2+10x+10x-2x=-1-25+25\\ \Leftrightarrow18x=-1\\ \Leftrightarrow x=-\dfrac{1}{18}\\ b,\left(2x-7\right)^2-\left(x+3\right)^2=3x^2+6\\ \Leftrightarrow4x^2-28x+49-x^2-6x-9-3x^2-6=0\\ \Leftrightarrow4x^2-x^2-3x^2-28x-6x=6+9-49\\ \Leftrightarrow22x=-34\\ \Leftrightarrow x=-\dfrac{17}{11}\\ c,\left(3x+2\right)^2-9\left(x-5\right)\left(x+5\right)=225-5x\\ \Leftrightarrow9x^2+12x+4-9\left(x^2-25\right)=225-5x\\ \Leftrightarrow9x^2-9x^2+12x+5x=225-4+9.25\\ \Leftrightarrow17x=446\\ \Leftrightarrow x=\dfrac{446}{17}\)
Sao bài này câu nào x cũng k nguyên ta, hơi xấu hi
GN
GV Nguyễn Trần Thành Đạt
Giáo viên
3 tháng 8 2023
9B
\(a,\left(4x-1\right)^2-4\left(2x-3\right)^2-x-4=0\\ \Leftrightarrow16x^2-8x+1-4\left(4x^2-12x+9\right)-x-4=0\\ \Leftrightarrow16x^2-16x^2-8x+48x-x=4+36-1\\ \Leftrightarrow39x=39\\ \Leftrightarrow x=1\\ b,x\left(x-5\right)-\left(4-x\right)^2=7x+1\\ \Leftrightarrow x^2-5x-\left(16-8x+x^2\right)-7x-1=0\\ \Leftrightarrow x^2-x^2-5x+8x-7x=1+16\\ \Leftrightarrow-4x=17\\ \Leftrightarrow x=\dfrac{-17}{4}\\ c,\left(2x-6\right)\left(x+3\right)=2\left(x-3\right)^2\\ \Leftrightarrow2x^2-6x+6x-18=2\left(x^2-6x+9\right)\\ \Leftrightarrow2x^2-2x^2-6x+6x+12x=18+18\\ \Leftrightarrow12x=36\\ \Leftrightarrow x=\dfrac{36}{12}=3\)
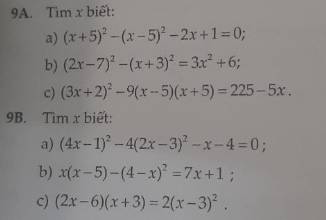
ừ