tìm n là số nguyên để phân thức sau nhận giá trị nguyên
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
KC
1
Những câu hỏi liên quan
HN
3
DT
Dang Tung
CTVHS
14 tháng 6 2023
a) Để A là phân số thì : \(n-2\ne0=>n\ne2\)
b) Để A nhận giá trị nguyên âm lớn nhất
\(=>A=-1\\ =>\dfrac{n-6}{n-2}=-1\\ =>n-6=-\left(n-2\right)\\ =>n-6=-n+2\\ =>n+n=6+2\\ =>2n=8\\ =>n=4\left(TMDK\right)\)
c) \(A=\dfrac{n-6}{n-2}=\dfrac{n-2-4}{n-2}=1-\dfrac{4}{n-2}\)
Để A nhận gt số nguyên thì : \(\dfrac{4}{n-2}\in Z=>4⋮\left(n-2\right)\\ =>n-2\inƯ\left(4\right)=\left\{\pm1;\pm2;\pm4\right\}\\ =>n\in\left\{3;1;4;0;6;-2\right\}\)
Đến đây bạn lập bảng giá trị rồi thay từng gt n vào bt A, giá trị nào cho A là STN thì bạn nhận gt đó ạ.
d) Mình nghĩ bạn thiếu đề ạ
C
2
16 tháng 11 2021
Để phân số này là số nguyên thì \(2x-5\in\left\{1;-1;19;-19\right\}\)
hay \(x\in\left\{3;2;12;-7\right\}\)
C
2
16 tháng 11 2021
\(\Rightarrow\dfrac{3x^2-6x+5x-10+14}{x-2}=3x+5+\dfrac{14}{x-2}\in Z\\ \Rightarrow x-2\inƯ\left(14\right)=\left\{-14;-7;-2;-1;1;2;7;14\right\}\\ \Rightarrow x\in\left\{-12;-5;0;1;3;4;9;16\right\}\)
16 tháng 11 2021
Để phân số này là số nguyên thì \(x-2\in\left\{1;-1;2;-2;7;-7;14;-14\right\}\)
hay \(x\in\left\{3;1;4;0;9;-5;16;-12\right\}\)
L
1
13 tháng 2 2019
Để 5n+1/3n+2 nhận giá trị nguyên
thì 5n+1 phải chia hết cho 3n+2
ta có:
5n+1 chia hết 3n+2
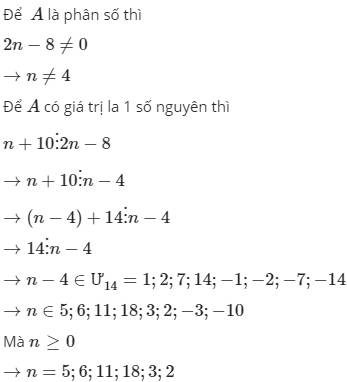
\(C\in Z\Leftrightarrow\left\{{}\begin{matrix}\dfrac{n-2}{2n+3}\in Z\left(1\right)\\\dfrac{n^2+2n+4}{2n+3}\in Z\left(2\right)\end{matrix}\right.\)
\(\left(1\right)\Leftrightarrow n-2⋮2n+3\\ \Leftrightarrow2n-4⋮2n+3\\ \Leftrightarrow2n+3\inƯ\left(7\right)=\left\{-7;-1;1;7\right\}\\ \Leftrightarrow n\in\left\{-5;-2;-1;2\right\}\left(3\right)\\ \left(2\right)\Leftrightarrow2n^2+4n+8⋮2n+3\\ \Leftrightarrow2n\left(n+3\right)-\left(2n+3\right)+11⋮2n+3\\ \Leftrightarrow2n+3\inƯ\left(11\right)=\left\{-11;-1;1;11\right\}\\ \Leftrightarrow n\in\left\{-7;-2;-1;4\right\}\left(4\right)\\ \left(3\right)\left(4\right)\Leftrightarrow n\in\left\{-2;-1\right\}\)
Vậy ...