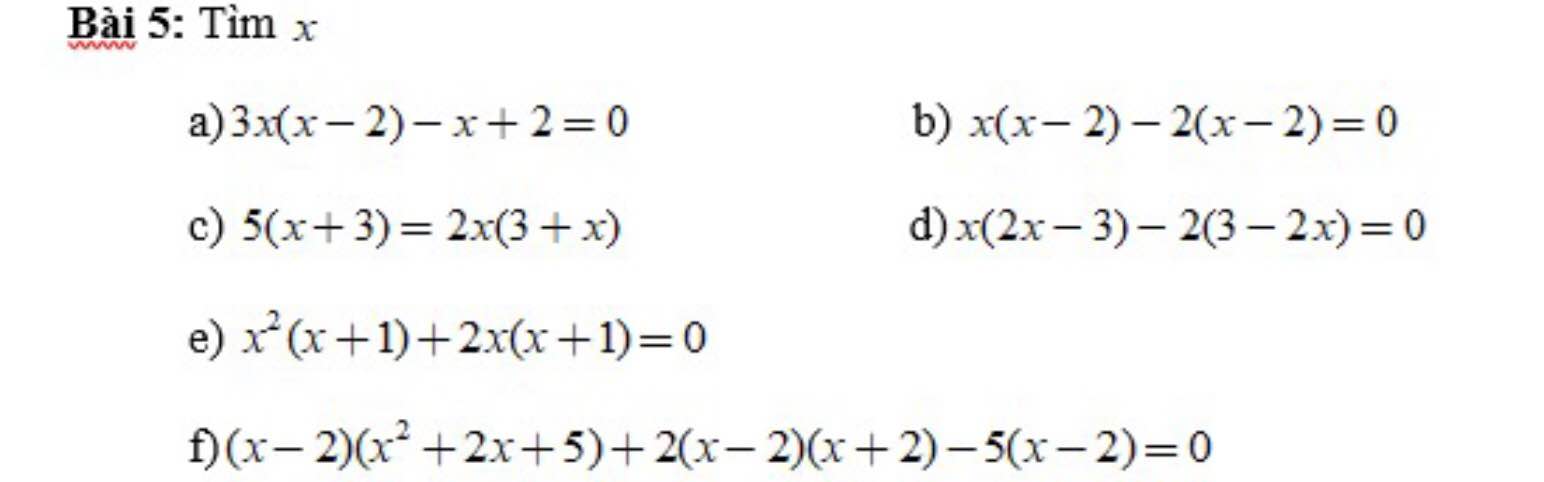 giải hết dùm em nha
giải hết dùm em nha
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



Diện tích xung quanh căn phòng là :
( 8 + 5 ) x 2 x 3 = 78 ( m2 )
Diện tích trần căn phòng là :
8 x 5 = 40 ( m2 )
a) Diện tích quét sơn là :
78 + 40 = 118 ( m2 )
Thể tích căn phòng đó là :
8 x 5 x 3 = 120 ( m3 )


c,\(\dfrac{5-x}{2}-\dfrac{3x+4}{3}=\dfrac{1}{4}\)
⇔\(\dfrac{5-x}{2}+\dfrac{-3x-4}{3}=\dfrac{1}{4}\)
⇔\(\dfrac{6\left(5-x\right)}{12}+\dfrac{4\left(-3x-4\right)}{12}=\dfrac{3}{12}\)
⇔6(5-x)+4(-3x-4)=3
⇔ 30-6x-12x-16=3
⇔ 30-16-3=12x+6x
⇔ 11=18x
⇔ x=\(\dfrac{11}{18}\)
Vậy S=\(\left\{\dfrac{11}{18}\right\}\)
d)x2-5x=9(x-5)
⇔x(x-5)=9(x-5)
⇔x(x-5)-9(x-5)=0
⇔(x-9)(x-5)=0
⇔\(\left\{{}\begin{matrix}x-9=0\Leftrightarrow x=9\\x-5=0\Leftrightarrow x=5\end{matrix}\right.\)
Vậy S=\(\left\{5;9\right\}\)

\(9,\\ =2-\sqrt{3}-\sqrt{3}-2=-2\sqrt{3}\left(B\right)\)

\(\Leftrightarrow n^5+n^2-n^2+1⋮n^3+1\)
\(\Leftrightarrow-n^3+n⋮n^3+1\)
\(\Leftrightarrow n=1\)



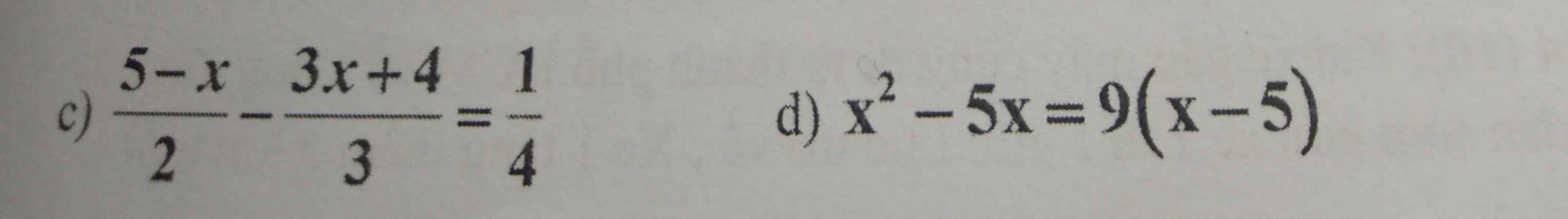
 giải dùm em câu 9 nha mng
giải dùm em câu 9 nha mng
a) \(3x\left(x-2\right)-x+2=0\)
\(3x\left(x-2\right)-\left(x-2\right)=0\)
\(\left(x-2\right)\left(3x-1\right)=0\)
⇔\(\left[{}\begin{matrix}x-2=0\\3x-1=0\end{matrix}\right.\)
⇔\(\left[{}\begin{matrix}x=2\\x=\dfrac{1}{3}\end{matrix}\right.\)
b) \(x\left(x-2\right)-2\left(x-2\right)=0\)
\(\left(x-2\right)\left(x-2\right)=0\)
\(\left(x-2\right)^2=0\)
⇔\(x-2=0\)
⇔\(x=2\)