cho a^2 + b^2 <= 2. CMR : a + b \(\le2\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
QD
1
18 tháng 6 2023
Ta có BDT luôn đúng \(\left(a-b\right)^2\ge0\) \(\Leftrightarrow a^2+b^2\ge2ab\) \(\Leftrightarrow2\left(a^2+b^2\right)\ge\left(a+b\right)^2\). Do \(a^2+b^2\le2\) nên \(2\left(a^2+b^2\right)\le4\).
Do đó \(\left(a+b\right)^2\le4\) \(\Leftrightarrow-2\le a+b\le2\), suy ra đpcm. ĐTXR \(\Leftrightarrow a=b=1\)
CD
1
NN
26 tháng 3 2017
vì a2 và b2 là 2 SCP nên chúng là STN
thử các trường hợp chỉ có 1 và 1 thỏa mãn => a và b đều = 1
=> a + b < 2(a + b)3 vì 2 < 16 (đpcm)
BU
1
9 tháng 8 2017
Giả sử (a+b)>2 thì (a+b)^2>4>>>>2(a^2+b^2)>=(a+b)^2>4>>>a^2+b^2>2(trái với gt đề bài)>>>Gt sai
>>>(a+b)<=2
3 tháng 4 2022
Bài 3:
\(\dfrac{1}{\left(x-y\right)^2}+\dfrac{1}{x^2}+\dfrac{1}{y^2}\ge\dfrac{4}{xy}\)
\(\Leftrightarrow x^2y^2\left(\dfrac{1}{\left(x-y\right)^2}+\dfrac{1}{x^2}+\dfrac{1}{y^2}\right)\ge\dfrac{4}{xy}.x^2y^2\)
\(\Leftrightarrow\dfrac{x^2y^2}{\left(x-y\right)^2}+x^2+y^2\ge4xy\)
\(\Leftrightarrow\dfrac{x^2y^2}{\left(x-y\right)^2}+x^2-2xy+y^2\ge2xy\)
\(\Leftrightarrow\left(\dfrac{xy}{x-y}\right)^2+\left(x-y\right)^2\ge2xy\)
\(\Leftrightarrow\left(\dfrac{xy}{x-y}\right)^2-2xy+\left(x-y\right)^2\ge0\)
\(\Leftrightarrow\left(\dfrac{xy}{x-y}-x+y\right)^2=0\) (luôn đúng)
29 tháng 8 2016
Áp dụng bất đẳng thức Cauchy ta có :
\(a^2+b^2\ge2\left|ab\right|\)
\(\Rightarrow\left|ab\right|\le1\)
\(\Leftrightarrow-1\le\left|ab\right|\le1\)
Ta có : \(a^2+b^2=\left(a+b\right)^2-2ab\)
\(\Rightarrow\left(a+b\right)^2\le2+2ab\le4\)
\(\Rightarrow a+b\le2\)
HP
2
LC
18 tháng 11 2016
Áp dụng bất đẳng thức Holder, ta có:
\(\left[\left(\sqrt[3]{a}\right)^3+\left(\sqrt[3]{b}\right)^3+1^3\right].\left(1^3+1^3+1^3\right).\left(1^3+1^3+1^3\right)\ge\left(\sqrt[3]{a}.1.1+\sqrt[3]{b}.1.1+1.1.1\right)^3\)
<=>\(\left(a+b+1\right).9\ge\left(\sqrt[3]{a}+\sqrt[3]{b}+1\right)^3\)
Vì a+b=3
=>\(\left(\sqrt[3]{a}+\sqrt[3]{b}+1\right)^3\le27\)
<=>\(\sqrt[3]{a}+\sqrt[3]{b}+1\le3\)
<=>\(\sqrt[3]{a}+\sqrt[3]{b}\le2\)
Dấu "=" xảy ra khi: a=b=1
=>ĐPCM
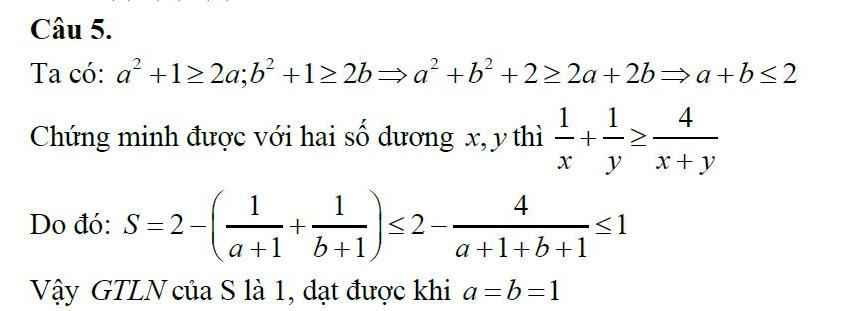
Ta có :
\(a^2+b^2\le2\) ( 1 )
Mặt khác \(2ab\le a^2+b^2\)nên
\(2ab\le a^2+b^2\le2\) ( 2 )
Cộng ( 1 ) với ( 2 ) , \(a^2+b^2+2ab\le4\)\(\Rightarrow\left(a+b\right)^2\le4\)\(\Rightarrow a+b\le2\)
a+b <=2 thế a+b=-3 thì sao???