Cho hàm số \(y=f\left(x\right)=-2010x\) với \(x_1;x_2\) là các giá trị của x.
a) Chứng minh nếu \(x_1>x_2\) thì \(f\left(x_1\right)>f\left(x_2\right)\)
b) Cho \(-1\le x\le10\) tìm GTNN, GTLN của \(f\left(x\right)\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NV
Nguyễn Việt Lâm
Giáo viên
13 tháng 1 2021
\(f\left(x_1\right)=ax_1\) ; \(f\left(x_2\right)=ax_2\) ; \(f\left(x_1x_2\right)=ax_1x_2\)
Để \(f\left(x_1\right)f\left(x_2\right)=f\left(x_1x_2\right)\)
\(\Leftrightarrow ax_1.ax_2=ax_1x_2\)
\(\Leftrightarrow a^2x_1x_2=ax_1x_2\)
\(\Leftrightarrow a^2=a\)
\(\Leftrightarrow\left[{}\begin{matrix}a=0\left(loại\right)\\a=1\end{matrix}\right.\)
Vậy \(a=1\)
6 tháng 11 2018
a) theo tính chất ta có: f(0+0)= f(0)+f(0)
=> f(0)=f(0)+f(0)
=> f(0)-f(0)=f(0)+f(0)-f(0)
=> 0=f(0)
hay f(0)=0
b) f(0)=f(-x+x)=f(-x)+f(x)
=>0=f(-x)+f(x)
=> f(-x)=0-f(x)=-f(x)
c) \(f\left(x_1-x_2\right)=f\left(x_1+\left(-x_2\right)\right)=f\left(x_1\right)+f\left(-x_2\right)=f\left(x_1\right)-f\left(x_2\right)\)
24 tháng 12 2018
Bài 1:
nếu x1<x2=>2018.x1-3<2018.x2
=>f(x1)<f(x2)
Bài 2:
nếu x dương=>100x2+2 dương
nếu x âm=>100x2+2 dương vì x2 luôn dương
=>f(x)=f(-x)
Bài 3:
nếu x1<x2=>-2019x1+1<2019x2+1
=>f(x1)<f(x2)
HQ
Hà Quang Minh
Giáo viên
23 tháng 9 2023
a) Thay \({x_1} = - 1;{x_2} = 1\) vào \(y = {x^2}\) ta được:
\({y_1} = f\left( { - 1} \right) = {\left( { - 1} \right)^2} = 1\)
\({y_2} = f\left( 1 \right) = {1^2} = 1\)
b) Ta có \({x_1} = - 1;{y_1} = 1 \Rightarrow {M_1}\left( { - 1;1} \right)\)
Ta có: \({x_2} = 1;{y_2} = 1 \Rightarrow {M_2}\left( {1;1} \right)\)
Biểu diễn trên mặt phẳng:
HP
27 tháng 12 2015
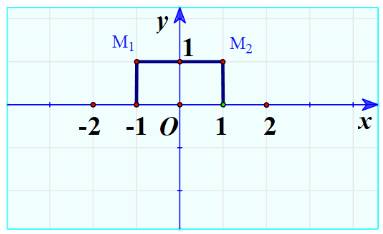
ta có:
\(f\left(x_1\right)=kx_1;f\left(x_2\right)=kx_2=>f\left(x_1-x_2\right)=k.\left(x_1-x_2\right)=kx_1-kx_2\)
vậy \(f\left(x_1-x_2\right)=f\left(x_1\right)-f\left(x_2\right)\)
tick mk nhé
20 tháng 11 2023
\(f\left(x_1\right)-f\left(x_2\right)=3x_1-3x_2=3\left(x_1-x_2\right)< 0\)
=>\(f\left(x_1\right)< f\left(x_2\right)\)
=>Hàm số đồng biến trên R
HQ
Hà Quang Minh
Giáo viên
23 tháng 9 2023
a) Ta có:
\(f\left( 1 \right) = 1 + 1 = 2\)
\(f\left( 2 \right) = 2 + 1 = 3\)
\( \Rightarrow f\left( 2 \right) > f\left( 1 \right)\)
b) Ta có:
\(f\left( {{x_1}} \right) = {x_1} + 1;f\left( {{x_2}} \right) = {x_2} + 1\)
\(\begin{array}{l}f\left( {{x_1}} \right) - f\left( {{x_2}} \right) = \left( {{x_1} + 1} \right) - \left( {{x_2} + 1} \right)\\ = {x_1} - {x_2} < 0\end{array}\)
Vậy \({x_1} < {x_2} \Rightarrow f\left( {{x_1}} \right) < f\left( {{x_2}} \right)\).