CM đa thức: g(x) = \(x^2-6x+10\) vô nghiệm
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NT
1
KT
23 tháng 1 2018
\(f\left(x\right)=9x^2+6x+2\)
\(=\left(9x^2+3x\right)+\left(3x+1\right)+1\)
\(=3x\left(3x+1\right)+\left(3x+1\right)+1\)
\(=\left(3x+1\right)\left(3x+1\right)+1\)
\(=\left(3x+1\right)^2+1\) \(>0\)
\(\Rightarrow\)đa thức vô nghiệm
b) \(g\left(x\right)=x^4-4x^2+2013\)
\(=\left(x^4-2x^2\right)-\left(2x^2-4\right)+2009\)
\(=x^2\left(x^2-2\right)-2\left(x^2-2\right)+2009\)
\(=\left(x^2-2\right)^2+2009\) \(>0\)
\(\Rightarrow\)đa thức vô nghiệm
28 tháng 6 2020
a) x2 + x + 1 = (x2 + x + 1/4) + 3/4 = (x + 1/2)2 + 3/4 > 0 => đa thức vô nghiệm
b) x2 - x + 1 = (x2 - x + 1/4) + 3/4 = (x - 1/2)2 + 3/4 > 0 => đa thức vô nghiệm
c) x2 - 6x + 10 = (x2 - 6x + 9) + 1 = (x - 3)2 + 1 > 0 => đa thức vô nghiệm
d) 9x2 + 6x + 2 = (9x2 + 6x + 1) + 1 = (3x + 1)2 + 1 > 0 => đa thức vô nghiệm
e) -2x2 + 8x - 11 = -2(x2 - 4x + 4) -3 = -2(x - 2)2 - 3 < 0 => đa thức vô nghiệm
g) -3x2 + 2x - 4 = -3(x2 - 2/3x + 1/9) - 11/3 < 0 => đa thức vô nghiệm
LP
1
NK
22 tháng 4 2017
f(x) = 2x2 + 6x +10 = 2(x2 + 3x + 5) = 2(x+1,5)2 + 5,5 >= 5,5 > 0
Vậy f(x) = 2x2 + 6x +10 vô nghiệm
NT
0
NN
0
NH
1
IC
1
H
2 tháng 3 2020
\(G\left(x\right)=x^2+2x+3\)
\(=x^2+x+x+1+2\)
\(=x.\left(x+1\right)+\left(x+1\right)+2\)
\(=\left(x+1\right).\left(x+1\right)+2\)
\(=\left(x+1\right)^2+2\ge2\)
Vậy G(x) vô nghiệm
\(A\left(x\right)=x^2-x+1\)
\(=x^2-\frac{1}{2}x-\frac{1}{2}x+\frac{1}{4}+\frac{3}{4}\)
\(=x.\left(x-\frac{1}{2}\right)-\frac{1}{2}.\left(x-\frac{1}{2}\right)+\frac{3}{4}\)
\(=\left(x-\frac{1}{2}\right).\left(x-\frac{1}{2}\right)+\frac{3}{4}\)
\(=\left(x-\frac{1}{2}\right)^2+\frac{3}{4}\ge\frac{3}{4}\)
Vậy A(x) vô nghiệm
ND
1
4 tháng 5 2021
$x^4-6x^2+15\\=x^4-3x^2-3x^2+9+6\\=x^2(x^2-3)-3(x^2-3)+6\\=(x^2-3)(x^2-3)+6\\=(x^2-3)^2+6\\(x^2-3)^2 \geq 0\\\to (x^2-3)^2+6 \geq 6>0\\\to x^4-6x^2+9$ vô nghiệm
2 tháng 7 2023
4:
a: f(x)=0
=>-x-4=0
=>x=-4
b: g(x)=0
=>x^2+x+4=0
Δ=1^2-4*1*4=1-16=-15<0
=>g(x) ko có nghiệm
c: m(x)=0
=>2x-2=0
=>x=1
d: n(x)=0
=>7x+2=0
=>x=-2/7
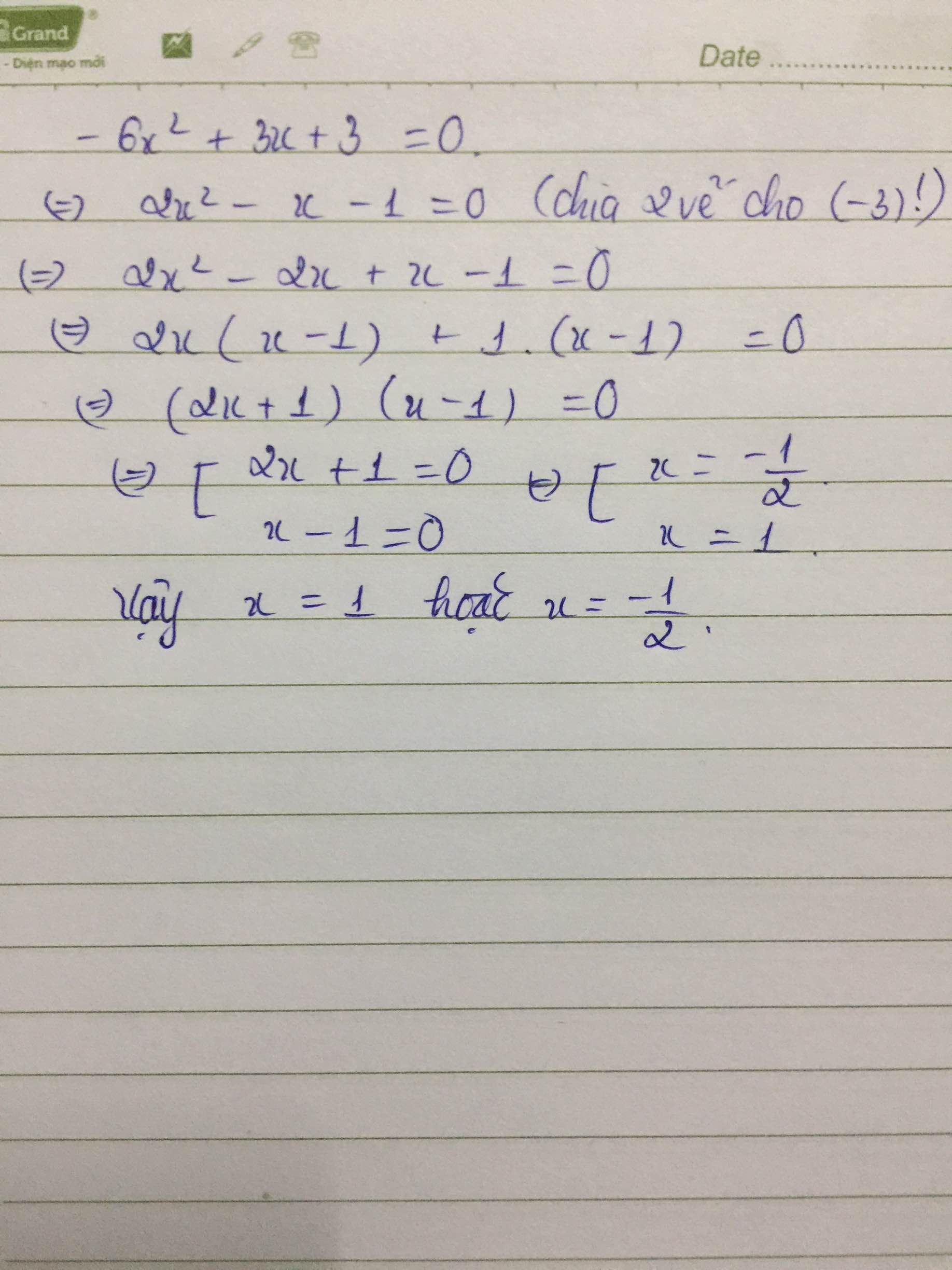
\(g\left(x\right)=x^2-6x+10\)
\(=x^2-6x+9+1\)
\(=\left(x-3\right)^2+1\ge1>0\)
Vậy ta có đpcm
ta có g(x) = x2-6x+10
= x2 - 2.x.3 + 32+ 1
= ( x-3)2+ 1
Ta thấy (x-3)2 \(\ge\) 0
=> (x-3)2+1\(\ge\) 1
Vậy đa thức vô nghiệm
Nếu hài lòng với câu trả lời này thì nhấn đúng ủng hộ mình nhé
Rất vui khi được giúp đỡ bạn