Tìm giá trị nhỏ nhât của 3x2 +1. Phân tích giống dạng hằng đẵng thức
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
PT
1
CM
3 tháng 9 2017
ĐKXĐ của phân thức x ≠ 1.
Ta có:
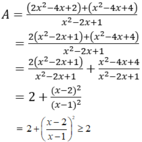
Vậy min A = 2 khi và chỉ khi x - 2 = 0 ⇔ x =2
CM
20 tháng 4 2019
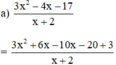
(Tách -4x = 6x – 10x để nhóm với 3x2 xuất hiện x + 2)
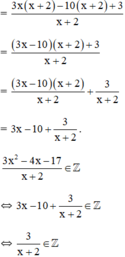
⇔ x + 2 ∈ Ư(3) = {±1; ±3}
+ x + 2 = 1 ⇔ x = -1
+ x + 2 = -1 ⇔ x = -3
+ x + 2 = 3 ⇔ x = 1
+ x + 2 = -3 ⇔ x = -5
Vậy với x = ±1 ; x = -3 hoặc x = -5 thì phân thức có giá trị nguyên.
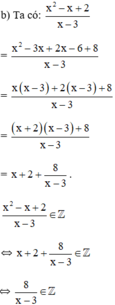
⇔ x – 3 ∈ Ư(8) = {±1; ±2; ±4; ±8}
+ x – 3 = 1 ⇔ x = 4
+ x – 3 = -1 ⇔ x = 2
+ x – 3 = 2 ⇔ x = 5
+ x – 3 = -2 ⇔ x = 1
+ x – 3 = 4 ⇔ x = 7
+ x – 3 = -4 ⇔ x = -1
+ x – 3 = 8 ⇔ x = 11
+ x – 3 = -8 ⇔ x = -5.
Vậy với x ∈ {-5; -1; 1; 2; 4; 5; 7; 11} thì giá trị phân thức là số nguyên.
PT
1
⇒ x
≠
±
2y
CM
8 tháng 12 2017
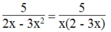xác định khi:
x(2 – 3x) ≠ 0 ⇔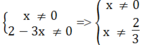 
Vậy phân thức 5 2 x - 3 x 2 xác định với x ≠ 0 và x ≠ 2/3
PT
0
AH
Akai Haruma
Giáo viên
12 tháng 9 2021
Đề bài bạn viết hơi khó hiểu, nhưng có thể tạm giải như sau:
Lời giải:
$A=\frac{4x^2}{x+1}=\frac{4(x^2-1)+4}{x+1}=\frac{4(x-1)(x+1)+4}{x+1}$
$=4(x-1)+\frac{4}{x+1}$
Với $x$ nguyên thì:
$A\in\mathbb{Z}\Leftrightarrow 4(x-1)+\frac{4}{x+1}\in\mathbb{Z}$
$\Leftrightarrow \frac{4}{x+1}\in\mathbb{Z}$
$\Leftrightarrow x+1$ là ước của $4$
$\Rightarrow x+1\in\left\{\pm 1;\pm 2;\pm 4\right\}$
$\Rightarrow x\in\left\{-2; 0; -3; 1; 3; -5\right\}$
19 tháng 12 2019
Bài 1:
a) x≠2x≠2
Bài 2:
a) x≠0;x≠5x≠0;x≠5
b) x2−10x+25x2−5x=(x−5)2x(x−5)=x−5xx2−10x+25x2−5x=(x−5)2x(x−5)=x−5x
c) Để phân thức có giá trị nguyên thì x−5xx−5x phải có giá trị nguyên.
=> x=−5x=−5
Bài 3:
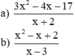
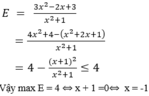
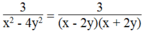
a) (x+12x−2+3x2−1−x+32x+2)⋅(4x2−45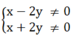)(x+12x−2+3x2−1−x+32x+2)⋅(4x2−45)
=(x+12(x−1)+3(x−1)(x+1)−x+32(x+1))⋅2(2x2−2)5=(x+12(x−1)+3(x−1)(x+1)−x+32(x+1))⋅2(2x2−2)5
=(x+1)2+6−(x−1)(x+3)2(x−1)(x+1)⋅2⋅2(x2−1)5=(x+1)2+6−(x−1)(x+3)2(x−1)(x+1)⋅2⋅2(x2−1)5
=(x+1)2+6−(x2+3x−x−3)(x−1)(x+1)⋅2(x−1)(x+1)5=(x+1)2+6−(x2+3x−x−3)(x−1)(x+1)⋅2(x−1)(x+1)5
=[(x+1)2+6−(x2+2x−3)]⋅25=[(x+1)2+6−(x2+2x−3)]⋅25
=[(x+1)2+6−x2−2x+3]⋅25=[(x+1)2+6−x2−2x+3]⋅25
=[(x+1)2+9−x2−2x]⋅25=[(x+1)2+9−x2−2x]⋅25
=2(x+1)25+185−25x2−45x=2(x+1)25+185−25x2−45x
=2(x2+2x+1)5+185−25x2−45x=2(x2+2x+1)5+185−25x2−45x
=2x2+4x+25+185−25x2−45x=2x2+4x+25+185−25x2−45x
=2x2+4x+2+185−25x2−45x=2x2+4x+2+185−25x2−45x
=2x2+4x+205−25x2−45x=2x2+4x+205−25x2−45x
c) tự làm, đkxđ: x≠1;x≠−1