Trong mặt phẳng với hệ tọa độ Oxy cho điểm C(2;-5) và đường thẳng d:3x -4y+4=0. Tìm trên d hai điểm A và B đối xứng nhau qua I(2;\(\frac{5}{2}\)) sao cho diện tích tam giác ABC bằng 15
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Giả sử tọa độ M(x;0). Khi đó \(\overrightarrow{MA}=\left(1-x;2\right);\overrightarrow{MB}=\left(4-x;3\right)\)
Theo giả thiết ta có \(\overrightarrow{MA}.\overrightarrow{MB}=MA.MB.\cos45^0\)
\(\Leftrightarrow\left(1-x\right)\left(4-x\right)+6=\sqrt{\left(1-x\right)^2+4}.\sqrt{\left(4-x\right)^2+9}.\frac{\sqrt{2}}{2}\)
\(\Leftrightarrow x^2-5x+10=\sqrt{x^2-2x+5}.\sqrt{x^2-8x+25}.\frac{\sqrt{2}}{2}\)
\(\Leftrightarrow2\left(x^2-5x+10\right)^2=\left(x^2-5x+10\right)\left(x^2-8x+25\right)\) (do \(x^2-5x+10>0\))
\(\Leftrightarrow x^4-10x^3+44x^2-110x+75=0\)
\(\Leftrightarrow\left(x-1\right)\left(x-5\right)\left(x^2-4x+15\right)=0\)
\(\Leftrightarrow x=1;x=5\)
Vậy ta có 2 điểm cần tìm là M(1;0) hoặc M(5;0)

\(\overrightarrow{AB}=\left(-4;4\right)=-4\left(1;-1\right)\)
\(\Rightarrow\) Phương trình CD song song AB đi qua D có dạng:
\(1\left(x+6\right)+1\left(y+8\right)=0\Leftrightarrow x+y+14=0\)
Gọi M là trung điểm AB \(\Rightarrow M\left(-6;4\right)\)
Phương trình đường thẳng d qua M và vuông góc AB có dạng:
\(1\left(x+6\right)-1\left(y-4\right)=0\Leftrightarrow x-y+10=0\)
Gọi N là giao điểm CD và d \(\Rightarrow\) N là trung điểm CD do ABCD là hình thang cân
Tọa độ N là nghiệm: \(\left\{{}\begin{matrix}x+y+14=0\\x-y+10=0\end{matrix}\right.\) \(\Rightarrow N\left(-12;-2\right)\)
\(\Rightarrow\left\{{}\begin{matrix}x_C=2x_N-x_D=...\\y_C=2y_N-y_D=...\end{matrix}\right.\)

\(\overrightarrow{AB}=\left(x_B-x_A;y_B-y_A\right)=\left(3;-9\right)\)

Đáp án C
Hình chiếu vuông góc của M(2;-1;4) lên mặt phẳng (Oxy) là điểm H(2;-1;0).


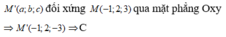
\(d\left(C;d\right)=\frac{\left|3.2-4\left(-5\right)+4\right|}{\sqrt{3^2+4^2}}=6\)
\(S_{ABC}=\frac{1}{2}AB.d\left(C;d\right)=\frac{1}{2}AB.6=15\Rightarrow AB=5\)
Gọi \(A\left(a;\frac{3a+4}{4}\right)\) \(\Rightarrow\overrightarrow{AI}=\left(a-2;\frac{3a-6}{4}\right)\Rightarrow AI=\sqrt{\left(a-2\right)^2+\left(\frac{3a-6}{4}\right)^2}=\frac{5}{2}\left|a-2\right|\)
\(AB=2IA\Rightarrow AI=\frac{5}{2}\Rightarrow\left|a-2\right|=1\Rightarrow\left[{}\begin{matrix}a=3\\a=1\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}A\left(3;\frac{13}{4}\right)\\B\left(1;\frac{7}{4}\right)\end{matrix}\right.\)