Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a)
Ta có:
∫π20cos2xsin2xdx=12∫π20cos2x(1−cos2x)dx=12∫π20[cos2x−1+cos4x2]dx=14∫π20(2cos2x−cos4x−1)dx=14[sin2x−sin4x4−x]π20=−14.π2=−π8∫0π2cos2xsin2xdx=12∫0π2cos2x(1−cos2x)dx=12∫0π2[cos2x−1+cos4x2]dx=14∫0π2(2cos2x−cos4x−1)dx=14[sin2x−sin4x4−x]0π2=−14.π2=−π8
b)
Ta có: Xét 2x – 2-x ≥ 0 ⇔ x ≥ 0.
Ta tách thành tổng của hai tích phân:
∫1−1|2x−2−x|dx=−∫0−1(2x−2−x)dx+∫10(2x−2−x)dx=−(2xln2+2−xln2)∣∣0−1+(2xln2+2−xln2)∣∣10=1ln2∫−11|2x−2−x|dx=−∫−10(2x−2−x)dx+∫01(2x−2−x)dx=−(2xln2+2−xln2)|−10+(2xln2+2−xln2)|01=1ln2
c)
∫21(x+1)(x+2)(x+3)x2dx=∫21x3+6x2+11x+6x2dx=∫21(x+6+11x+6x2)dx=[x22+6x+11ln|x|−6x]∣∣21=(2+12+11ln2−3)−(12+6−6)=212+11ln2∫12(x+1)(x+2)(x+3)x2dx=∫12x3+6x2+11x+6x2dx=∫12(x+6+11x+6x2)dx=[x22+6x+11ln|x|−6x]|12=(2+12+11ln2−3)−(12+6−6)=212+11ln2
d)
∫201x2−2x−3dx=∫201(x+1)(x−3)dx=14∫20(1x−3−1x+1)dx=14[ln|x−3|−ln|x+1|]∣∣20=14[1−ln2−ln3]=14(1−ln6)∫021x2−2x−3dx=∫021(x+1)(x−3)dx=14∫02(1x−3−1x+1)dx=14[ln|x−3|−ln|x+1|]|02=14[1−ln2−ln3]=14(1−ln6)
e)
∫π20(sinx+cosx)2dx=∫π20(1+sin2x)dx=[x−cos2x2]∣∣π20=π2+1∫0π2(sinx+cosx)2dx=∫0π2(1+sin2x)dx=[x−cos2x2]|0π2=π2+1
g)
I=∫π0(x+sinx)2dx∫π0(x2+2xsinx+sin2x)dx=[x33]∣∣π0+2∫π0xsinxdx+12∫π0(1−cos2x)dxI=∫0π(x+sinx)2dx∫0π(x2+2xsinx+sin2x)dx=[x33]|0π+2∫0πxsinxdx+12∫0π(1−cos2x)dx
Tính :J=∫π0xsinxdxJ=∫0πxsinxdx
Đặt u = x ⇒ u’ = 1 và v’ = sinx ⇒ v = -cos x
Suy ra:
J=[−xcosx]∣∣π0+∫π0cosxdx=π+[sinx]∣∣π0=πJ=[−xcosx]|0π+∫0πcosxdx=π+[sinx]|0π=π
Do đó:
I=π33+2π+12[x−sin2x2]∣∣π30=π33+2π+π2=2π3+15π6

Câu nào mình biết thì mình làm nha.
1) Đổi thành \(\dfrac{y^4}{4}+y^3-2y\) rồi thế số.KQ là \(\dfrac{-3}{4}\)
2) Biến đổi thành \(\dfrac{t^2}{2}+2\sqrt{t}+\dfrac{1}{t}\) và thế số.KQ là \(\dfrac{35}{4}\)
3) Biến đổi thành 2sinx + cos(2x)/2 và thế số.KQ là 1

a)
Ta có \(A=\int ^{\frac{\pi}{4}}_{0}\cos 2x\cos^2xdx=\frac{1}{4}\int ^{\frac{\pi}{4}}_{0}\cos 2x(\cos 2x+1)d(2x)\)
\(\Leftrightarrow A=\frac{1}{4}\int ^{\frac{\pi}{2}}_{0}\cos x(\cos x+1)dx=\frac{1}{4}\int ^{\frac{\pi}{2}}_{0}\cos xdx+\frac{1}{8}\int ^{\frac{\pi}{2}}_{0}(\cos 2x+1)dx\)
\(\Leftrightarrow A=\frac{1}{4}\left.\begin{matrix} \frac{\pi}{2}\\ 0\end{matrix}\right|\sin x+\frac{1}{16}\left.\begin{matrix} \frac{\pi}{2}\\ 0\end{matrix}\right|\sin 2x+\frac{1}{8}\left.\begin{matrix} \frac{\pi}{2}\\ 0\end{matrix}\right|x=\frac{1}{4}+\frac{\pi}{16}\)
b)
\(B=\int ^{1}_{\frac{1}{2}}\frac{e^x}{e^{2x}-1}dx=\frac{1}{2}\int ^{1}_{\frac{1}{2}}\left ( \frac{1}{e^x-1}-\frac{1}{e^x+1} \right )d(e^x)\)
\(\Leftrightarrow B=\frac{1}{2}\left.\begin{matrix} 1\\ \frac{1}{2}\end{matrix}\right|\left | \frac{e^x-1}{e^x+1} \right |\approx 0.317\)
c)
Có \(C=\int ^{1}_{0}\frac{(x+2)\ln(x+1)}{(x+1)^2}d(x+1)\).
Đặt \(x+1=t\)
\(\Rightarrow C=\int ^{2}_{1}\frac{(t+1)\ln t}{t^2}dt=\int ^{2}_{1}\frac{\ln t}{t}dt+\int ^{2}_{1}\frac{\ln t}{t^2}dt\)
\(=\int ^{2}_{1}\ln td(\ln t)+\int ^{2}_{1}\frac{\ln t}{t^2}dt=\frac{\ln ^22}{2}+\int ^{2}_{1}\frac{\ln t}{t^2}dt\)
Đặt \(\left\{\begin{matrix} u=\ln t\\ dv=\frac{dt}{t^2}\end{matrix}\right.\Rightarrow \left\{\begin{matrix} du=\frac{dt}{t}\\ v=\frac{-1}{t}\end{matrix}\right.\Rightarrow \int ^{2}_{1}\frac{\ln t}{t^2}dt=\left.\begin{matrix} 2\\ 1\end{matrix}\right|-\frac{\ln t+1}{t}=\frac{1}{2}-\frac{\ln 2 }{2}\)
\(\Rightarrow C=\frac{1}{2}-\frac{\ln 2}{2}+\frac{\ln ^22}{2}\)

1/ \(I=\int\limits^1_0\dfrac{2x+1}{x^2+x+1}dx=\int\limits^1_0\dfrac{d\left(x^2+x+1\right)}{x^2+x+1}=ln\left|x^2+x+1\right||^1_0=ln3\)
2/ \(\int\limits^{\dfrac{1}{2}}_0\dfrac{5x}{\left(1-x^2\right)^3}dx=-\dfrac{5}{2}\int\limits^{\dfrac{1}{2}}_0\dfrac{d\left(1-x^2\right)}{\left(1-x^2\right)^3}=\dfrac{5}{4}\dfrac{1}{\left(1-x^2\right)^2}|^{\dfrac{1}{2}}_0=\dfrac{35}{36}\)
3/ \(\int\limits^1_0\dfrac{2x}{\left(x+1\right)^3}dx\Rightarrow\) đặt \(x+1=t\Rightarrow x=t-1\Rightarrow dx=dt;\left\{{}\begin{matrix}x=0\Rightarrow t=1\\x=1\Rightarrow t=2\end{matrix}\right.\)
\(I=\int\limits^2_1\dfrac{2\left(t-1\right)dt}{t^3}=\int\limits^2_1\left(\dfrac{2}{t^2}-\dfrac{2}{t^3}\right)dt=\left(\dfrac{-2}{t}+\dfrac{1}{t^2}\right)|^2_1=\dfrac{1}{4}\)
4/ \(\int\limits^1_0\dfrac{4x-2}{\left(x^2+1\right)\left(x+2\right)}dx\)
Kĩ thuật chung là tách và sử dụng hệ số bất định như sau:
\(\dfrac{4x-2}{\left(x^2+1\right)\left(x+2\right)}=\dfrac{ax+b}{x^2+1}+\dfrac{c}{x+2}=\dfrac{\left(a+c\right)x^2+\left(2a+b\right)x+2b+c}{\left(x^2+1\right)\left(x+2\right)}\)
\(\Rightarrow\left\{{}\begin{matrix}a+c=0\\2a+b=4\\2b+c=-2\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}b=0\\a=-c=2\end{matrix}\right.\)
\(\Rightarrow I=\int\limits^1_0\left(\dfrac{2x}{x^2+1}-\dfrac{2}{x+2}\right)dx=\int\limits^1_0\dfrac{d\left(x^2+1\right)}{x^2+1}-2\int\limits^1_0\dfrac{d\left(x+2\right)}{x+2}=ln\dfrac{8}{9}\)
5/ \(\int\limits^1_0\dfrac{x^2dx}{x^6-9}\Rightarrow\) đặt \(x^3=t\Rightarrow3x^2dx=dt\Rightarrow x^2dx=\dfrac{1}{3}dt;\left\{{}\begin{matrix}x=0\Rightarrow t=0\\x=1\Rightarrow t=1\end{matrix}\right.\)
\(I=\dfrac{1}{3}\int\limits^1_0\dfrac{dt}{t^2-9}=\dfrac{1}{18}\int\limits^1_0\left(\dfrac{1}{t-3}-\dfrac{1}{t+3}\right)dt=\dfrac{1}{18}ln\left|\dfrac{t-3}{t+3}\right||^1_0=-\dfrac{1}{18}ln2\)
6/ Tương tự câu 4, sử dụng hệ số bất định ta tách được:
\(\int\limits^2_1\dfrac{2x-1}{x^2\left(x+1\right)}dx=\int\limits^2_1\left(\dfrac{3x-1}{x^2}-\dfrac{3}{x+1}\right)dx=\int\limits^2_1\left(\dfrac{3}{x}-\dfrac{1}{x^2}-\dfrac{3}{x+1}\right)dx\)
\(=\left(3ln\left|\dfrac{x}{x+1}\right|+\dfrac{1}{x}\right)|^2_1=3ln\dfrac{4}{3}-\dfrac{1}{2}\)
a) =
= 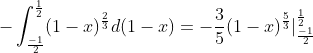
=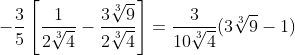
b) =
=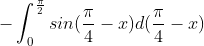 =
= 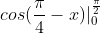
=
c)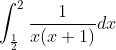 =
=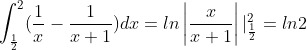
d)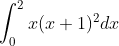 =
= 
=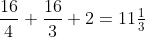
e)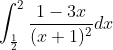 =
= 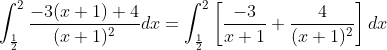
=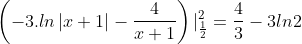
g)Ta có f(x) = sin3xcos5x là hàm số lẻ.
Vì f(-x) = sin(-3x)cos(-5x) = -sin3xcos5x = f(-x) nên: