Tìm các giới hạn sau: lim x → -...

K

Khách

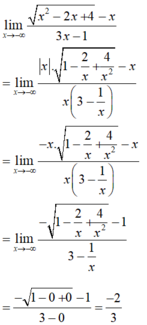
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Mua vip

PT

Pham Trong Bach

15 tháng 6 2017

Tìm các giới hạn sau: lim x → - ∞ x 2 - 2 x + 4 - x 3 x - 1

#Hỏi cộng đồng OLM #Toán lớp 11

1

CM

Cao Minh Tâm

15 tháng 6 2017

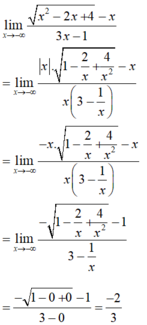
Giải bài tập Toán 11 | Giải Toán lớp 11

Các câu hỏi dưới đây có thể giống với câu hỏi trên

SG

Sách Giáo Khoa

11 tháng 4 2017

Tìm các giới hạn sau :

a) \(\lim\limits_{x\rightarrow-2}\dfrac{x+5}{x^2+x-3}\)

b) \(\lim\limits_{x\rightarrow3^-}\sqrt{x^2+8x+3}\)

c) \(\lim\limits_{x\rightarrow+\infty}\left(x^3+2x^2\sqrt{x}-1\right)\)

d) \(\lim\limits_{x\rightarrow-1}\dfrac{2x^3-5x-4}{\left(x+1\right)^2}\)

#Hỏi cộng đồng OLM #Toán lớp 11

0

SG

Sách Giáo Khoa

4 tháng 4 2017

Dùng định nghĩa, tìm các giới hạn sau :

a) \(\lim\limits_{x\rightarrow4}\dfrac{x+1}{3x-2}\)

b) \(\lim\limits_{x\rightarrow+\infty}\dfrac{2-5x^2}{x^2+3}\)

#Hỏi cộng đồng OLM #Toán lớp 11

1

DP

Đặng Phương Nam

4 tháng 4 2017

a) Hàm số f(x) = $\frac{x +1}{3x - 2}$ xác định trên R\{ $\frac{2}{3}$ } và ta có x = 4 ∈ ( $\frac{2}{3}$ ;+∞).

Giả sử (x_n) là dãy số bất kì và x_n ∈ ( $\frac{2}{3}$ ;+∞); x_n ≠ 4 và x_n→ 4 khi n→ +∞.

Ta có lim f(x_n) = lim $\frac{x_{n} +1}{3x_{n} - 2}$ = $\frac{4 + 1}{3. 4 - 2}$ = $\frac{1}{2}$ .

Vậy $\underset{x\rightarrow 4}{lim}$ $\frac{x +1}{3x - 2}$ = $\frac{1}{2}$ .

b) Hàm số f(x) = $\frac{2-5x^{2}}{x^{2}+3}$ xác định trên R.

Giả sử (x_n) là dãy số bất kì và x_n→ +∞ khi n→ +∞.

Ta có lim f(x_n) = lim $\frac{2-5x^{2}_{n}}{x^{2}_{n}+3}$ = lim $\frac{\frac{2}{x^{2}_{n}}-5}{1+\frac{3}{x^{2}_{n}}}$ = -5.

Vậy $\underset{x\rightarrow +\infty }{lim}$ $\frac{2-5x^{2}}{x^{2}+3}$ = -5.

SG

Sách Giáo Khoa

4 tháng 4 2017

Tìm các giới hạn sau...

#Hỏi cộng đồng OLM #Toán lớp 11

1

DP

Đặng Phương Nam

4 tháng 4 2017

Ôn tập chương IV

NT

Nguyễn Trọng Hiếu

4 tháng 5 2016

Tìm giới hạn sau :

\(\lim\limits_{x\rightarrow2}\frac{x-\sqrt{3x-2}}{x^2-4}\)

#Hỏi cộng đồng OLM #Toán lớp 11

1

NT

Nguyễn Thái Bình

4 tháng 5 2016

\(L=\lim\limits_{x\rightarrow2}\frac{x-\sqrt{3x-2}}{x^2-4}\)

\(=\lim\limits_{x\rightarrow2}\frac{x^2-3x+2}{\left(x-4\right)\left(x+\sqrt{3x-2}\right)}=\lim\limits_{x\rightarrow2}\frac{\left(x-2\right)\left(x-1\right)}{\left(x-2\right)\left(x+2\right)\left(x+\sqrt{3x-2}\right)}\)

\(=\lim\limits_{x\rightarrow2}\frac{x-1}{\left(x+2\right)\left(x+\sqrt{3x-2}\right)}=\frac{1}{16}\)

SG

Sách Giáo Khoa

4 tháng 4 2017

Tính các giới hạn sau...

#Hỏi cộng đồng OLM #Toán lớp 11

1

DP

Đặng Phương Nam

4 tháng 4 2017

a) $\underset{x\rightarrow -3}{lim}$ $\frac{x^{2 }-1}{x+1}$ = $\frac{(-3)^{2}-1}{-3 +1}$ = -4.

b) $\underset{x\rightarrow -2}{lim}$ $\frac{4-x^{2}}{x + 2}$ = $\underset{x\rightarrow -2}{lim}$ $\frac{ (2-x)(2+x)}{x + 2}$ = $\underset{x\rightarrow -2}{lim}$ (2-x) = 4.

c) $\underset{x\rightarrow 6}{lim}$ $\frac{\sqrt{x + 3}-3}{x-6}$ = $\underset{x\rightarrow 6}{lim}$ $\frac{(\sqrt{x + 3}-3)(\sqrt{x + 3}+3 )}{(x-6) (\sqrt{x + 3}+3 )}$
= $\underset{x\rightarrow 6}{lim}$ $\frac{x +3-9}{(x-6) (\sqrt{x + 3}+3 )}$ = $\underset{x\rightarrow 6}{lim}$ $\frac{1}{\sqrt{x+3}+3}$ = $\frac{1}{6}$ .

d) $\underset{x\rightarrow +\infty }{lim}$ $\frac{2x-6}{4-x}$ = $\underset{x\rightarrow +\infty }{lim}$ $\frac{2-\frac{6}{x}}{\frac{4}{x}-1}$ = -2.

e) $\underset{x\rightarrow +\infty }{lim}$ $\frac{17}{x^{2}+1}$ = 0 vì $\underset{x\rightarrow +\infty }{lim}$ (x² + 1) = $\underset{x\rightarrow +\infty }{lim}$ x²( 1 + $\frac{1}{x^{2}}$ ) = +∞.

f) $\underset{x\rightarrow +\infty }{lim}$ $\frac{-2x^{2}+x -1}{3 +x}$ = $\underset{x\rightarrow +\infty }{lim}$ $\frac{-2+\frac{1}{x} -\frac{1}{x^{2}}}{\frac{3}{x^{2}} +\frac{1}{x}}$ = -∞, vì $\frac{3}{x^{2}}+\frac{1}{x}$ > 0 với ∀x>0.

SG

Sách Giáo Khoa

10 tháng 4 2017

Dùng định nghĩa tìm các giới hạn :

a) \(\lim\limits_{x\rightarrow5}\dfrac{x+3}{3-x}\)\(\)

b) \(\lim\limits_{x\rightarrow+\infty}\dfrac{x^3+1}{x^2+1}\)

#Hỏi cộng đồng OLM #Toán lớp 11

0

SG

Sách Giáo Khoa

4 tháng 4 2017

Tìm các giới hạn sau :

a) \(\lim\limits_{x\rightarrow2}\dfrac{3x-5}{\left(x-2\right)^2}\)

b) \(\lim\limits_{x\rightarrow1^-}\dfrac{2x-7}{x-1}\)

c) \(\lim\limits_{x\rightarrow1^+}\dfrac{2x-7}{x-1}\)

#Hỏi cộng đồng OLM #Toán lớp 11

1

DP

Đặng Phương Nam

4 tháng 4 2017

a) Ta có $\underset{x\rightarrow 2}{lim}$ (x - 2)²= 0 và (x - 2)²> 0 với ∀x ≠ 2 và $\underset{x\rightarrow 2}{lim}$ (3x - 5) = 3.2 - 5 = 1 > 0.

Do đó $\underset{x\rightarrow 2}{lim}$ $\frac{3x -5}{(x-2)^{2}}$ = +∞.

b) Ta có $\underset{x\rightarrow 1^{-}}{lim}$ (x - 1) và x - 1 < 0 với ∀x < 1 và $\underset{x\rightarrow 1^{-}}{lim}$ (2x - 7) = 2.1 - 7 = -5 <0.

Do đó $\underset{x\rightarrow 1^{-}}{lim}$ $\frac{2x -7}{x-1}$ = +∞.

c) Ta có $\underset{x\rightarrow 1^{+}}{lim}$ (x - 1) = 0 và x - 1 > 0 với ∀x > 1 và $\underset{x\rightarrow 1^{+}}{lim}$ (2x - 7) = 2.1 - 7 = -5 < 0.

Do đó $\underset{x\rightarrow 1^{+}}{lim}$ $\frac{2x -7}{x-1}$ = -∞.

NT

Nguyen Thi Mai

4 tháng 4 2017

Giỏi quá ta, chắc là hs cao tuổi nhất ...

SG

Sách Giáo Khoa

11 tháng 4 2017

Tìm các giới hạn sau...

#Hỏi cộng đồng OLM #Toán lớp 11

0

LN

lu nguyễn

10 tháng 3 2020

tìm các giới hạn sau:

a; \(\lim\limits_{x\rightarrow1}\frac{2x^4-5x^3+3x^2+1}{3x^4-8x^3+6x^2-1}\)

b; \(\lim\limits_{x\rightarrow1}\frac{x^3-3x^2+2}{x^4-4x+3}\)

c; \(\lim\limits_{x\rightarrow1}\frac{x^3-2x-1}{x^5-2x-1}\)

d; \(\lim\limits_{x\rightarrow-1}\frac{\left(x+2\right)^2-1}{x^2-1}\)

#Hỏi cộng đồng OLM #Toán lớp 11

3

AH

Akai Haruma

Giáo viên

12 tháng 3 2020

a.

\(\lim\limits_{x\to 1+}\frac{2x^4-5x^3+3x^2+1}{3x^4-8x^3+6x^2-1}=\lim_{x\to 1+}\frac{2x^4-5x^3+3x^2+1}{(x-1)^3(3x+1)}=\lim\limits _{x\to 1+}\frac{2x^4-5x^3+3x^2+1}{3x+1}.\lim\limits_{x\to 1+}\frac{1}{(x-1)^3}\)

\(=\frac{1}{4}.(+\infty)=+\infty \)

Hoàn toàn tương tự:

\(\lim\limits_{x\to 1-}\frac{2x^4-5x^3+3x^2+1}{3x^4-8x^3+6x^2-1}=-\infty \)

Do đó: \(\lim\limits_{x\to 1+}\frac{2x^4-5x^3+3x^2+1}{3x^4-8x^3+6x^2-1}\neq \lim\limits_{x\to 1-}\frac{2x^4-5x^3+3x^2+1}{3x^4-8x^3+6x^2-1}\) nên không tồn tại \(\lim\limits_{x\to 1}\frac{2x^4-5x^3+3x^2+1}{3x^4-8x^3+6x^2-1}\)

AH

Akai Haruma

Giáo viên

12 tháng 3 2020

b.

\(\lim\limits_{x\to 1+}\frac{x^3-3x^2+2}{x^4-4x+3}=\lim\limits_{x\to 1+}\frac{(x-1)(x^2-2x-2)}{(x-1)^2(x^2+2x+3)}=\lim\limits_{x\to 1+}\frac{x^2-2x-2}{(x-1)(x^2+2x+3)}\)

\(=\lim\limits_{x\to 1+}\frac{x^2-2x-2}{x^2+2x+3}.\lim\limits_{x\to 1+}\frac{1}{x-1}=\frac{-1}{2}.(+\infty)=-\infty \)

Tương tự \(\lim\limits_{x\to 1-}\frac{x^3-3x^2+2}{x^4-4x+3}=+\infty \)

Do đó không tồn tại \(\lim\limits_{x\to 1}\frac{x^3-3x^2+2}{x^4-4x+3}\)

c.

\(\lim\limits_{x\to 1}\frac{x^3-2x-1}{x^5-2x-1}=\frac{1^3-2.1-1}{1^5-2.1-1}=1\)

d.

\(\lim\limits_{x\to -1}\frac{(x+2)^2-1}{x^2-1}=\lim\limits_{x\to -1}\frac{(x+2-1)(x+2+1)}{(x-1)(x+1)}=\lim\limits_{x\to -1}\frac{x+3}{x-1}=-1\)

LN

lu nguyễn

26 tháng 2 2020

tìm các giới hạn sau:

a; \(\lim\limits_{x\rightarrow\frac{\pi}{2}}\frac{sin\left(x-\frac{\pi}{4}\right)}{x}\)

b, \(\lim\limits_{x\rightarrow2}\frac{\sqrt[3]{3x^2-4}-\sqrt{3x-2}}{x+1}\)

c,\(\lim\limits_{x\rightarrow0}x^2sin\frac{1}{2}\)

#Hỏi cộng đồng OLM #Toán lớp 11

1

NV

Nguyễn Việt Lâm

Giáo viên

27 tháng 2 2020

Tất cả đều ko phải dạng vô định, bạn cứ thay số vào tính thôi:

\(a=\frac{sin\left(\frac{\pi}{4}\right)}{\frac{\pi}{2}}=\frac{\sqrt{2}}{\pi}\)

\(b=\frac{\sqrt[3]{3.4-4}-\sqrt{6-2}}{3}=\frac{0}{3}=0\)

\(c=0.sin\frac{1}{2}=0\)

LN

lu nguyễn

27 tháng 2 2020

câu b: là gh dạng 0/0 chứa căn không đồng bậc thì phải thêm bớt mà đâu phải thay số đâu mình tính rồi nhưng số xấu bằng \(\frac{38-2\sqrt{6}}{15}\)