Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

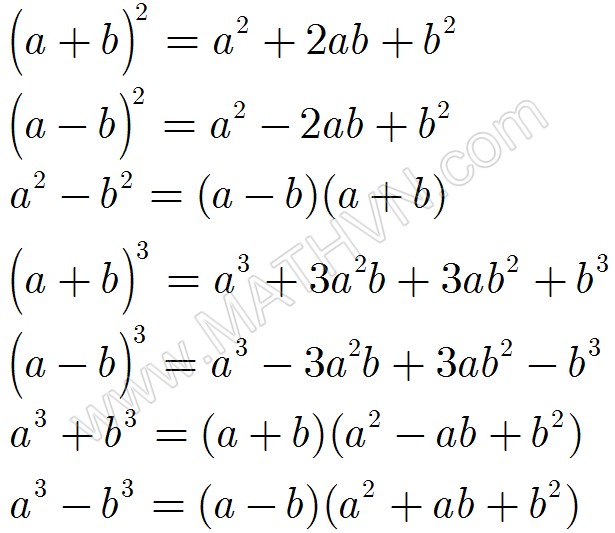
a) \(\left(2x-1\right)^2=49\)
\(\Leftrightarrow\orbr{\begin{cases}2x-1=7\\2x-1=-7\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}2x=8\\2x=-6\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}x=4\\x=-3\end{cases}}\)

a)<=>(x^2+x-3)(x^2+x-2)-12=(x-2)(x+3)(x^2+x+1)
TH1:=>x-2=0
=>x=2
TH2:x+3=0
=>x=-3
dựa vô bệt thức ta thấy
D<0=> phương trình ko có nghiệm thực
=>x=-3 hoặc 2
nhớ tick nhé


1) \(x^4-6x^3-x^2+54x-72=0\)
\(\Leftrightarrow x^3\left(x-2\right)-4x^2\left(x-2\right)-9x\left(x-2\right)+36\left(x-2\right)=0\)
\(\Leftrightarrow\left(x-2\right)\left(x^3-4x^2-9x+36\right)=0\)
\(\Leftrightarrow\left(x-2\right)\left[x^2\left(x-4\right)-9\left(x-4\right)\right]=0\)
\(\Leftrightarrow\left(x-2\right)\left(x-4\right)\left(x^2-9\right)=0\)
\(\Leftrightarrow\left(x-2\right)\left(x-4\right)\left(x-3\right)\left(x+3\right)=0\)
Tự làm nốt...
2) \(x^4-5x^2+4=0\)
\(\Leftrightarrow x^2\left(x^2-1\right)-4\left(x^2-1\right)=0\)
\(\Leftrightarrow\left(x^2-1\right)\left(x^2-4\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(x+1\right)\left(x-2\right)\left(x+2\right)=0\)
Tự làm nốt...
\(x^4-2x^3-6x^2+8x+8=0\)
\(\Leftrightarrow x^3\left(x-2\right)-6x\left(x-2\right)-4\left(x-2\right)=0\)
\(\Leftrightarrow\left(x-2\right)\left(x^3-6x-4\right)=0\)
\(\Leftrightarrow\left(x-2\right)\left[x^2\left(x+2\right)-2x\left(x+2\right)-2\left(x+2\right)\right]=0\)
\(\Leftrightarrow\left(x-2\right)\left(x+2\right)\left(x^2-2x-2\right)=0\)
\(\Leftrightarrow\left(x-2\right)\left(x+2\right)\left[\left(x-1\right)^2-\left(\sqrt{3}\right)^2\right]=0\)
\(\Leftrightarrow\left(x-2\right)\left(x+2\right)\left(x-1-\sqrt{3}\right)\left(x-1+\sqrt{3}\right)=0\)
...
\(2x^4-13x^3+20x^2-3x-2=0\)
\(\Leftrightarrow2x^3\left(x-2\right)-9x^2\left(x-2\right)+2x\left(x-2\right)+\left(x-2\right)=0\)
\(\Leftrightarrow\left(x-2\right)\left(2x^3-9x^2+2x+1\right)=0\)
Bí

a)x2+(x-3)(3x-5)=9
<=>x2+3x2-5x-9x+15=9
,<=>4x2-14x+15=9
<=>4x2-14x+6=0
<=>4x2-12x-2x+6=0
<=>4x(x-3)-2(x-3)=0
<=>(x-3)(4x-2)=0
=> x-3=0 hoặc 4x-2=0 =>x=3 hoặc x=1/2
b)(3x+2)2=(x-4)2
<=>(3x+2)2-(x-4)2=0
<=>(3x+2-x+4)(3x+2+x-4)=0 (HẰNG ĐẲNG THỨC SỐ 3)
<=>(2x+6)(4x-2)=0
=>2x+6=0 hoặc 4x-2 => x=-3 hoặc x=1/2
c)Chưa ra thông cảm ahihi
c, x4+2x3-2x2+2x-3 = 0
<=> (x4-x3)+(3x3-3x2)+(x2-x)+(3x-3) = 0
<=> x3(x-1)+3x2(x-1)+x(x-1)+3(x-1) = 0
<=> (x-1)(x3+3x2+x+3) = 0
<=> (x-1)[x2(x+3)+(x+3)] = 0
<=> (x-1)(x+3)(x2+1) = 0
<=> x-1 =0 hoặc x+3=0 ( vì x2+1 khác 0 )
<=> x =1 hoặc x= -3


1) \(x^4-2x^2-144x+1295=0\)
\(\Rightarrow\)Cậu xem lại đề thử xem nhé !
2) \(x\left(x-1\right)\left(x+1\right)\left(x+2\right)=24\)
\(\Leftrightarrow\left(x^2+2x\right)\left(x^2-1\right)-24=0\)
\(\Leftrightarrow x^4+2x^3-x^2-2x-24=0\)
\(\Leftrightarrow x^4+x^3+4x^2+x^3+x^2+4x-6x^2-6x-24=0\)
\(\Leftrightarrow x^2\left(x^2+x+4\right)+x\left(x^2+x+4\right)-6\left(x^2+x+4\right)=0\)
\(\Leftrightarrow\left(x^2+x-6\right)\left(x^2+x+4\right)=0\)
\(\Leftrightarrow\left(x^2+3x-2x-6\right)\left(x^2+x+4\right)=0\)
\(\Leftrightarrow\left[x\left(x+3\right)-2\left(x+3\right)\right]\left(x^2+x+4\right)=0\)
\(\Leftrightarrow\left(x+3\right)\left(x-2\right)\left(x^2+x+4\right)=0\)
\(\Leftrightarrow\)\(x+3=0\)
hoặc \(x-2=0\)
hoặc \(x^2+x+4=0\)
\(\Leftrightarrow\)\(x=-3\left(tm\right)\)
hoặc \(x=2\left(tm\right)\)
hoặc \(\left(x+\frac{1}{2}\right)^2+\frac{15}{4}=0\left(ktm\right)\)
Vậy tập nghiệm của phương trình là : \(S=\left\{-3;2\right\}\)
3) \(x^4-2x^3+4x^2-3x-10=0\)
\(\Leftrightarrow x^4+x^3-3x^3-3x^2+7x^2+7x-10x-10=0\)
\(\Leftrightarrow x^3\left(x+1\right)-3x^2\left(x+1\right)+7x\left(x+1\right)-10\left(x+1\right)=0\)
\(\Leftrightarrow\left(x+1\right)\left(x^3-3x^2+7x-10\right)=0\)
\(\Leftrightarrow\left(x+1\right)\left(x^3-2x^2-x^2+2x+5x-10\right)=0\)
\(\Leftrightarrow\left(x+1\right)\left[x^2\left(x-2\right)-x\left(x-2\right)+5\left(x-2\right)\right]=0\)
\(\Leftrightarrow\left(x+1\right)\left(x-2\right)\left(x^2-x+5\right)=0\)
\(\Leftrightarrow\)\(x+1=0\)
hoặc \(x-2=0\)
hoặc \(x^2-x+5=0\)
\(\Leftrightarrow x=-1\left(tm\right)\)
hoặc \(x=2\left(tm\right)\)
hoặc \(\left(x-\frac{1}{2}\right)^2+\frac{19}{4}=0\left(ktm\right)\)
Vậy tập nghiệm của phương trình là :\(S=\left\{-1;2\right\}\)
Đặt \(\left\{{}\begin{matrix}x-9=a\\x-10=b\end{matrix}\right.\)
\(a^4+b^4=\left(a+b\right)^4\)
\(\Leftrightarrow a^4+b^4=a^4+4a^3b+6a^2b^2+4ab^3+b^4\)
\(\Leftrightarrow2ab\left(2a^2+3ab+2b^2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}a=0\\b=0\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=9\\x=10\end{matrix}\right.\)