
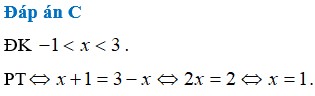
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

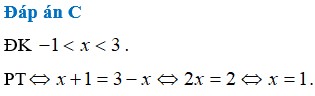

ta có
\(\)\(y=\frac{1}{3}\log^3_{\frac{1}{2}}x+\log^2_{\frac{1}{2}}x-3\log_{\frac{1}{2}}x+1\)
Đặt =\(t=\log_{\frac{1}{2}}x\) ta có
\(y=\frac{1}{3}t^3+t^2-3t+1\)
với \(\frac{1}{4}\le x\le4\Leftrightarrow\frac{1}{4}\le\left(\frac{1}{2}\right)^t\le4\Leftrightarrow-2\le t\le2\)
thay vì tính GTLN,GTNN của hàm số y trên [1/4;4] ta tính GTLN,GTNN của hàm số trên [-2;2]
ta tính \(y'=t^2+2t-3\)
ta tính y'=0 suy ra t=1(loại);t=-3(loại)
ta tính y(2)=\(\frac{5}{3}\);y(-2)=\(\frac{-25}{3}\)
vậy GTNN của y=\(\frac{-25}{3}khi\log_{\frac{1}{2}}x=-2\Rightarrow x=4\)
hàm số đạt GTLN y=\(\frac{5}{3}\) khi \(\log_{\frac{1}{2}}x=2\Leftrightarrow x=\left(\frac{1}{2}\right)^2=\frac{1}{4}\)

a) ĐK: x-1 khác 0 và x+1 khác 0
<=> x khác 1 và x khác -1
b) ĐK: x-2 khác 0
<=> x khác 2

a) -2x+14=0
<=>-2x= - 14
<=>x = 7
Vậy phương trình có tập nghiệm x={7}
b)(4x-10) (x+5)=0
<=>4x-10=0 <=>4x=10 <=>x=5/2
<=>x+5=0 <=>x=-5
Vậy phương trình có tập nghiệm x={5/2;- 5}
c)\(\frac{1-x}{x+1}\) + 3=\(\frac{2x+3}{x+1}\)
ĐKXD: x+1 #0<=>x#-1(# là khác)
\(\frac{1-x}{x+1}\)+3=\(\frac{2x+3}{x+1}\)
<=>\(\frac{1-x}{x+1}\)+\(\frac{3.\left(x+1\right)}{x+1}\)=\(\frac{2x+3}{x+1}\)
<=>\(\frac{1-x}{x+1}\)+\(\frac{3x+3}{x+1}\)=\(\frac{2x+3}{x+1}\)
=>1-x+3x+3=2x+3
<=>-x+3x-2x=-1-3+3
<=>0x = -1 (vô nghiệm)
Vâyj phương trình vô nghiệm
d) 1,2-(x-0,8)=-2(0,9+x)
<=> 1,2-x+0,8=-1,8-2x
<=>-x+2x=-1,2-0,8-1,8
<=>x=-4
Vậy phương trình có tập nghiệm x={-4}

Lời giải:
Điều kiện \(x\geq 0\)
\(\text{PT}\Leftrightarrow 2(x^2+2x+4)=3\sqrt{4x(x^2+4)}\)
Áp dụng bất đẳng thức AM-GM:
\(2(x^2+2x+4)=3\sqrt{4x(x^2+4)}\leq 3\left (\frac{4x+x^2+4}{2}\right)\)
\(\Rightarrow 4(x^2+2x+4)\leq 3(x^2+4x+4)\Leftrightarrow (x-2)^2\leq 0\)
Ta biết rằng \((x-2)^2\geq 0\forall x\in\mathbb{R}\) nên dấu bằng xảy ra khi \(x=2\)
Vậy \(x=2\) là nghiệm của phương trình.


điều kiện : \(\begin{cases}x\ne1\\x\ne2\end{cases}\)
phương trình: \(\Leftrightarrow\left(x+m\right)\left(x-2\right)=\left(x+3\right)\left(x-1\right)\)
\(\Leftrightarrow x^2+\left(m-2\right)x-2m=x^2+2x-3\)
\(\Leftrightarrow\left(m-4\right)x=2m-3\)
+ m = 4 phương trình vô nghiệm
+ m\(\ne\) 4 phương trình \(\Leftrightarrow x=\frac{2m-3}{m-4}\)
do điều kiện : \(\begin{cases}x\ne1\\x\ne2\end{cases}\)nên \(\begin{cases}\frac{2m-3}{m+1}\ne1\\\frac{2m-3}{m-4}\ne2\end{cases}\) \(\Leftrightarrow\) \(\begin{cases}2m-3\ne m-4\\2m-3\ne2m-8\end{cases}\)
\(\Leftrightarrow m\ne-1\)
vậy: + \(m\in\left\{4;-1\right\}\): phương trình vô nghiệm
+ \(m\in R\text{ /}\left\{4;-1\right\}\) :phương trình có nghiệm duy nhất \(x=\frac{2m-3}{m-4}\)