Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Do \(1\le x\le2\Rightarrow\left(x-1\right)\left(x-2\right)\le0\)
\(\Leftrightarrow x^2+2\le3x\)
Hoàn toàn tương tự ta có \(y^2+2\le3y\)
Do đó: \(P\ge\dfrac{x+2y}{3x+3y+3}+\dfrac{2x+y}{3x+3y+3}+\dfrac{1}{4\left(x+y-1\right)}\)
\(P\ge\dfrac{x+y}{x+y+1}+\dfrac{1}{4\left(x+y-1\right)}\)
Đặt \(a=x+y-1\Rightarrow1\le a\le3\)
\(\Rightarrow P\ge f\left(a\right)=\dfrac{a+1}{a+2}+\dfrac{1}{4a}\)
\(f'\left(a\right)=\dfrac{3a^2-4a-4}{4a^2\left(a+2\right)^2}=\dfrac{\left(a-2\right)\left(3a+2\right)}{4a^2\left(a+2\right)^2}=0\Rightarrow a=2\)
\(f\left(1\right)=\dfrac{11}{12}\) ; \(f\left(2\right)=\dfrac{7}{8}\) ; \(f\left(3\right)=\dfrac{53}{60}\)
\(\Rightarrow f\left(a\right)\ge\dfrac{7}{8}\Rightarrow P_{min}=\dfrac{7}{8}\) khi \(\left(x;y\right)=\left(1;2\right);\left(2;1\right)\)

Ta có : \(P=\frac{\left(\frac{x}{y}\right)^3}{\frac{x}{y}+\frac{y}{z}}+\frac{\left(\frac{y}{z}\right)^3}{\frac{x}{y}+\frac{y}{z}}+\left(\frac{z}{x}\right)^2+\frac{15}{\frac{z}{x}}\)
Đặt \(a=\frac{x}{y};b=\frac{y}{z};c=\frac{z}{x}\Rightarrow a,b,c=1,c>1\)
Biểu thức viết lại : \(P=\frac{a^3}{a+b}+\frac{b^3}{a+b}+c^2+\frac{15}{c}\)
Ta có : \(a^3+b^3\ge ab\left(a+b\right)\Rightarrow\frac{a^3}{a+b}+\frac{b^3}{a+b}\ge ab=\frac{1}{c}\) vì a,b>0
Vậy \(P\ge\frac{1}{c}+c^2+\frac{15}{c}=c^2+\frac{16}{c}=f\left(c\right)\) với mọi \(c\in\left(1;+\infty\right)\)
Ta có \(f'\left(c\right)=2c-\frac{16}{c}\Rightarrow f'\left(c\right)=0\Leftrightarrow c=2\)
Lập bảng biến thiên ta có \(f'\left(c\right)\ge f\left(2\right)=12\) khi và chỉ khi \(c=2\Rightarrow a=b=\frac{1}{\sqrt{2}}\Rightarrow z=\sqrt{2}y=2x\)
Vậy giá trị nhỏ nhất P=12 khi và chỉ khi \(z=\sqrt{2}y=2x\)

Đk: \(x\ge2;y\ge-1;0< x+y\le9\)
Ta có: \(\sqrt{2x-4}+\frac{1}{\sqrt{2}}\sqrt{2(y+1)}\leq\sqrt{3(x+y-1)}\)
Từ giả thiết suy ra
\(x+y-1=\sqrt{2x-4}+\sqrt{y+1}\Rightarrow x+y-1\leq\sqrt{3(x+y-1)}\)
Vậy \(1\leq(x+y)\leq4\). Đặt \(\left\{\begin{matrix}t=x+y\\t\in\left[1;4\right]\end{matrix}\right.\) ta có:
\(P=t^2-\sqrt{9-t}+\frac{1}{\sqrt{t}}\)
\(P'\left(t\right)=2t+\frac{1}{2\sqrt{9-t}}-\frac{1}{2t\sqrt{t}}>0\forall t\in\left[1;4\right]\)
Vậy \(P\left(t\right)\) đồng biến trên \([1;4]\)
Suy ra \(P_{max}=P\left(4\right)=4^2-\sqrt{9-4}+\frac{1}{\sqrt{4}}=\frac{33-2\sqrt{5}}{2}\) khi \(\left\{\begin{matrix}x=4\\y=0\end{matrix}\right.\)
\(P_{min}=P\left(1\right)=2-2\sqrt{2}\) khi \(\left\{\begin{matrix}x=2\\y=-1\end{matrix}\right.\)

\(4=2^x+2^y\ge2\sqrt{2^{x+y}}\Rightarrow2^{x+y}\le4\Rightarrow x+y\le2\)
\(\Rightarrow xy\le1\)
\(P=4x^2y^2+2x^3+2y^3+10xy\)
\(P=4x^2y^2+10xy+2\left(x+y\right)\left[\left(x+y\right)^2-3xy\right]\)
\(P\le4x^2y^2+10xy+4\left(4-3xy\right)=4x^2y^2-2xy+16\)
Đặt \(xy=t\Rightarrow0< t\le1\)
Xét hàm \(f\left(t\right)=4t^2-2t+16\) trên \((0;1]\)
\(\Rightarrow...\)

Đặt \(x+y=t,t\in\left[-2;2\right]\)
Biến đổi được \(P=-2t^3+6t\)
Xét \(f\left(t\right)=-2t^3+6t\) trên \(\left[-2;2\right]\)
Lập bảng biến thiên
Ta có \(P_{Max}=4\) khi t=1
\(P_{Min}=-4\) khi t= -1
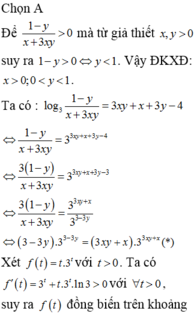
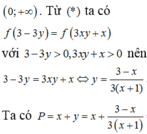
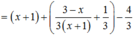
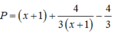
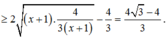
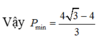
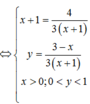
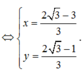

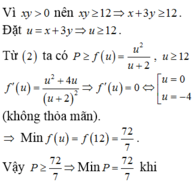
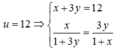
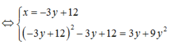
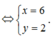
\(log_{\frac{1}{2}}x+log_{\frac{1}{2}}y\le log_{\frac{1}{2}}\left(x+y^2\right)\Leftrightarrow log_{\frac{1}{2}}\left(xy\right)\le log_{\frac{1}{2}}\left(x+y^2\right)\Leftrightarrow xy\ge x+y^2\)
\(\Leftrightarrow x\left(y-1\right)\ge y^2\Leftrightarrow\left\{{}\begin{matrix}y>1\\x\ge\frac{y^2}{y-1}\end{matrix}\right.\)
\(\Rightarrow P=x+3y\ge\frac{y^2}{y-1}+3y=4y+1+\frac{1}{y-1}=4\left(y-1\right)+\frac{1}{y-1}+5\)
\(\Rightarrow P\ge2\sqrt{4\left(y-1\right).\frac{1}{\left(y-1\right)}}+5=9\)
\(\Rightarrow P_{min}=9\) khi \(\left\{{}\begin{matrix}x=\frac{9}{2}\\y=\frac{3}{2}\end{matrix}\right.\)