Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
a: \(\overrightarrow{AI}=\dfrac{1}{2}\left(\overrightarrow{AM}+\overrightarrow{AN}\right)=\dfrac{1}{4}\overrightarrow{AB}+\dfrac{1}{4}\overrightarrow{AC}\)
Lời giải:
Ta có:
\(\overrightarrow{AB}=\overrightarrow{AM}+\overrightarrow{MB}=\overrightarrow{AM}+\overrightarrow{MN}+\overrightarrow{NB}=\overrightarrow{AM}-\overrightarrow{BN}+\overrightarrow{MN}\)
Vì $AM,BN$ là trung tuyến nên $M,N$ lần lượt là trung điểm của $BC, AC$
$\Rightarrow MN$ là đường trung bình của tam giác $ABC$ ứng với $AB$
\(\Rightarrow \overrightarrow{MN}=\frac{1}{2}\overrightarrow{BA}=-\frac{1}{2}\overrightarrow{AB}\). Do đó:
\(\overrightarrow{AB}=\overrightarrow{AM}-\overrightarrow{BN}-\frac{1}{2}\overrightarrow{AB}\Leftrightarrow \frac{3}{2}\overrightarrow{AB}=\overrightarrow{AM}-\overrightarrow{BN}\)
\(\Leftrightarrow \overrightarrow{AB}=\frac{2}{3}\overrightarrow{AM}-\frac{2}{3}\overrightarrow{BN}\)
Cho tam giác ABC có trung tuyến BM và trọng tâm G . Phân tích vecto BG theo hai vecto BA và vecto BC
\(\overrightarrow{BG}=\dfrac{2}{3}\overrightarrow{BM}=\dfrac{2}{3}\overrightarrow{BA}+\dfrac{2}{3}\overrightarrow{BC}\)
Đặt \(\overrightarrow{b}=x\cdot\overrightarrow{a}+y\cdot\overrightarrow{c}\)
mà \(\overrightarrow{b}=\left(-1;-1\right);\overrightarrow{a}=\left(4;-2\right);\overrightarrow{c}=\left(2;5\right)\)
nên \(\left\{{}\begin{matrix}4x+2y=-1\\-2x+5y=-1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}4x+2y=-1\\-4x+10y=-2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}12y=-3\\4x+2y=-1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}y=-\dfrac{1}{4}\\4x=-1-2y=-1-2\cdot\dfrac{-1}{4}=-1+\dfrac{1}{2}=-\dfrac{1}{2}\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=-\dfrac{1}{8}\\y=-\dfrac{1}{4}\end{matrix}\right.\)
Vậy: \(\overrightarrow{b}=\dfrac{-1}{8}\cdot\overrightarrow{a}+\dfrac{-1}{4}\cdot\overrightarrow{c}\)
\(\overrightarrow{AN}=\overrightarrow{AB}+\overrightarrow{BN}=-\overrightarrow{BA}+\dfrac{2}{3}\overrightarrow{BA}+\dfrac{2}{3}\overrightarrow{AC}\)
\(=\dfrac{1}{3}\overrightarrow{AB}+\dfrac{2}{3}\overrightarrow{AC}\)
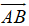
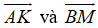
Ta có M là trung điểm của AC nên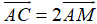
K là trung điểm của BC nên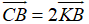
Bạn tự vẽ hình minh họa nha :>
Gọi G là giao điểm của AK, BM thì G là trọng tâm của tam giác.
Ta có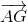
= 
=> 
=
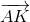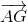Theo quy tắc 3 điểm đối với tổng vec-tơ:
AK là trung tuyến thuộc cạnh BC nên
Từ đây ta có
= 
+
=> 
= -
- 
.
BM là trung tuyến thuộc đỉnh B nên:
=>
= 
+
.