giup mink voi
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NV
1
4 tháng 1 2024
Nhân vật chính trong chuyện "Hai kiểu áo" là: viên quan và thợ may.
Viên quan: luôn tìm cách xu nịnh luồn lách để thăng tiến nhưng lại có thái độ khinh thường, bắt nạt những người dân đen nghèo khổ.
Thợ may: người nhìn thấu bộ mặt thối nát của quan lại
TH
1
NN
Nguyễn Ngọc Anh Minh
CTVHS
VIP
19 tháng 5 2022
a/
\(\left(104,5-14,1+9,6\right)xx=25\)
\(\Rightarrow100xx=25\Rightarrow x=\dfrac{25}{100}=\dfrac{1}{4}\)
b/
\(T=\dfrac{\left(2011-2\right)x2010+2000}{2011x2010-2020}=\)
\(=\dfrac{2011x2010-4020+2000}{2011x2010-2020}=\dfrac{2011x2010-2020}{2011x2010-2020}=1\)
29 tháng 11 2023
a: \(\left(\overrightarrow{AB},\overrightarrow{AC}\right)=\widehat{BAC}=90^0\)
ΔABC vuông tại A
=>\(\widehat{ABC}+\widehat{ACB}=90^0\)
=>\(\widehat{ACB}=30^0\)
\(\left(\overrightarrow{CA};\overrightarrow{CB}\right)=\widehat{ACB}=30^0\)
Lấy M sao cho \(\overrightarrow{AB}=\overrightarrow{BM}\)
=>AB=BM và B nằm giữa A và M
=>B là trung điểm của AM
Ta có: \(\widehat{ABC}+\widehat{MBC}=180^0\)(hai góc kề bù)
=>\(\widehat{MBC}+60^0=180^0\)
=>\(\widehat{MBC}=120^0\)
\(\left(\overrightarrow{AB},\overrightarrow{BC}\right)=\left(\overrightarrow{BM},\overrightarrow{BC}\right)=\widehat{MBC}=120^0\)
b: Vì ΔABC vuông tại A nên \(\overrightarrow{AB}\cdot\overrightarrow{AC}=0\)
Xét ΔABC vuông tại A có \(sinABC=\dfrac{AC}{BC}\)
=>\(\dfrac{4}{BC}=sin60=\dfrac{\sqrt{3}}{2}\)
=>\(BC=\dfrac{8\sqrt{3}}{3}\)
ΔABC vuông tại A
=>\(AB^2+AC^2=BC^2\)
=>\(AB^2=BC^2-AC^2=\left(\dfrac{8}{\sqrt{3}}\right)^2-4^2=\dfrac{16}{3}\)
=>\(AB=\dfrac{4\sqrt{3}}{3}\)
MB=BA
mà \(AB=\dfrac{4\sqrt{3}}{3}\)
nên \(MB=\dfrac{4\sqrt{3}}{3}\)
\(\overrightarrow{AB}\cdot\overrightarrow{BC}=\overrightarrow{BM}\cdot\overrightarrow{BC}\)
\(=BM\cdot BC\cdot cos\left(\overrightarrow{BM},\overrightarrow{BC}\right)\)
\(=\dfrac{4\sqrt{3}}{3}\cdot\dfrac{8\sqrt{3}}{3}\cdot cos120=-\dfrac{16}{3}\)
c: \(\overrightarrow{AB}\left(\overrightarrow{BC}+\overrightarrow{BA}\right)\)
\(=\overrightarrow{AB}\cdot\overrightarrow{BC}+\overrightarrow{AB}\cdot\overrightarrow{BA}\)
\(=-\dfrac{16}{3}-AB^2=-\dfrac{16}{3}-\left(\dfrac{4}{\sqrt{3}}\right)^2=-\dfrac{32}{3}\)
29 tháng 11 2023
a: \(\overrightarrow{a}=\left(2;-3\right);\overrightarrow{b}=\left(4;8\right);\overrightarrow{c}=\left(-7;3\right)\)
Tọa độ của \(\overrightarrow{a}+\overrightarrow{b}\) là:
\(\left\{{}\begin{matrix}x=2+4=6\\y=-3+8=5\end{matrix}\right.\)
Tọa độ của \(3\overrightarrow{a}-2\overrightarrow{b}+5\overrightarrow{c}\) là:
\(\left\{{}\begin{matrix}x=3\cdot2-2\cdot4+5\left(-7\right)=-37\\y=3\cdot\left(-3\right)-2\cdot8+5\cdot3=-10\end{matrix}\right.\)
b: \(\overrightarrow{2a}+\overrightarrow{u}-\overrightarrow{c}=\overrightarrow{0}\)
=>\(\overrightarrow{u}=-2\overrightarrow{a}+\overrightarrow{c}\)
Tọa độ của vecto u là:
\(\left\{{}\begin{matrix}x=-2\cdot2+\left(-7\right)=-11\\y=-2\cdot\left(-3\right)+3=6+3=9\end{matrix}\right.\)
c: Đặt \(\overrightarrow{c}=x\cdot\overrightarrow{a}+y\cdot\overrightarrow{b}\)
=>\(\left\{{}\begin{matrix}-7=2x+4y\\3=-3x+8y\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}4x+8y=-14\\-3x+8y=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}7x=-17\\-3x+8y=3\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=-\dfrac{17}{7}\\8y=3x+3=\dfrac{-51}{7}+3=-\dfrac{30}{7}\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=-\dfrac{17}{7}\\y=-\dfrac{30}{7\cdot8}=-\dfrac{30}{56}=-\dfrac{15}{28}\end{matrix}\right.\)
Vậy: \(\overrightarrow{c}=-\dfrac{17}{7}\cdot\overrightarrow{a}+\dfrac{-15}{28}\cdot\overrightarrow{b}\)
d: \(\left|\overrightarrow{a}\right|=\sqrt{2^2+\left(-3\right)^2}=\sqrt{13}\)
\(\overrightarrow{b}-\overrightarrow{c}=\left(11;5\right)\)
=>\(\left|\overrightarrow{b}-\overrightarrow{c}\right|=\sqrt{11^2+5^2}=\sqrt{146}\)
e: \(\overrightarrow{a}\cdot\overrightarrow{b}=2\cdot4+\left(-3\right)\cdot8=-24+8=-16\)
\(\overrightarrow{a}-\overrightarrow{b}=\left(-2;-11\right);\overrightarrow{b}+\overrightarrow{c}=\left(-3;11\right)\)
\(\left(\overrightarrow{a}-\overrightarrow{b}\right)\left(\overrightarrow{b}+\overrightarrow{c}\right)=\left(-2\right)\cdot\left(-3\right)+\left(-11\right)\cdot11=-121+6=-115\)
f: \(cos\left(\overrightarrow{a},\overrightarrow{b}\right)=\dfrac{\overrightarrow{a}\cdot\overrightarrow{b}}{\left|\overrightarrow{a}\right|\cdot\left|\overrightarrow{b}\right|}=\dfrac{-16}{\sqrt{2^2+\left(-3\right)^2}\cdot\sqrt{4^2+8^2}}\)
\(=\dfrac{-16}{\sqrt{13}\cdot4\sqrt{5}}=-\dfrac{4}{\sqrt{65}}\)
15 tháng 6 2023
4:
=145(69+22+8+1)
=145*100=14500
3:
Xe 1 chở: (168+26):2=194:2=97 tạ
Xe 2 chở 97-26=71 tạ
M
2
Y
12 tháng 2 2023
\(\left(-\dfrac{7}{23}\right).\left(\dfrac{13}{28}\right)+\left(-\dfrac{7}{23}\right).\left(\dfrac{25}{28}\right)\)
\(=\left(-\dfrac{7}{23}\right).\left(\dfrac{13}{28}+\dfrac{25}{28}\right)\)
\(=\left(-\dfrac{7}{23}\right).\left(\dfrac{38}{28}\right)\)
\(=\left(-\dfrac{7}{23}\right).\left(\dfrac{19}{14}\right)\)
\(=-\dfrac{19}{46}\)
PL
12 tháng 2 2023
\(\left(-\dfrac{7}{23}\right)\cdot\left(\dfrac{13}{28}\right)+\left(-\dfrac{7}{13}\right)\cdot\left(\dfrac{25}{28}\right)\)
\(=\left(-\dfrac{7}{23}\right)\cdot\left(\dfrac{13}{28}+\dfrac{25}{28}\right)\)
\(=\left(-\dfrac{7}{13}\right)\cdot\dfrac{38}{28}\)
\(=\left(-\dfrac{7}{13}\right)\cdot\dfrac{19}{14}\)
\(=-\dfrac{19}{26}\)
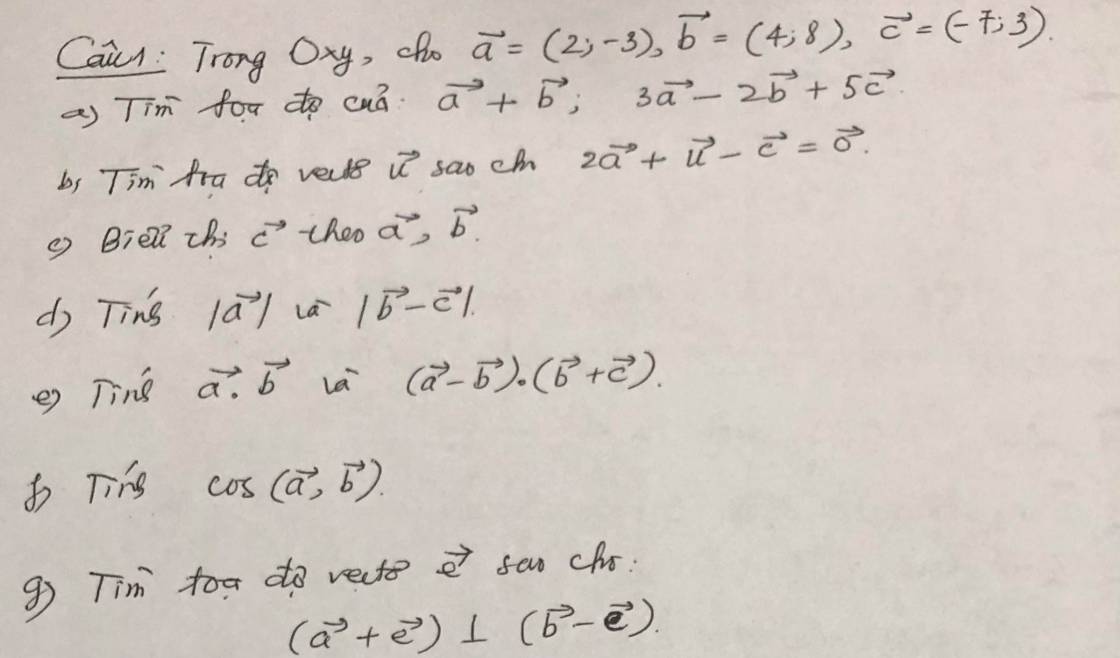
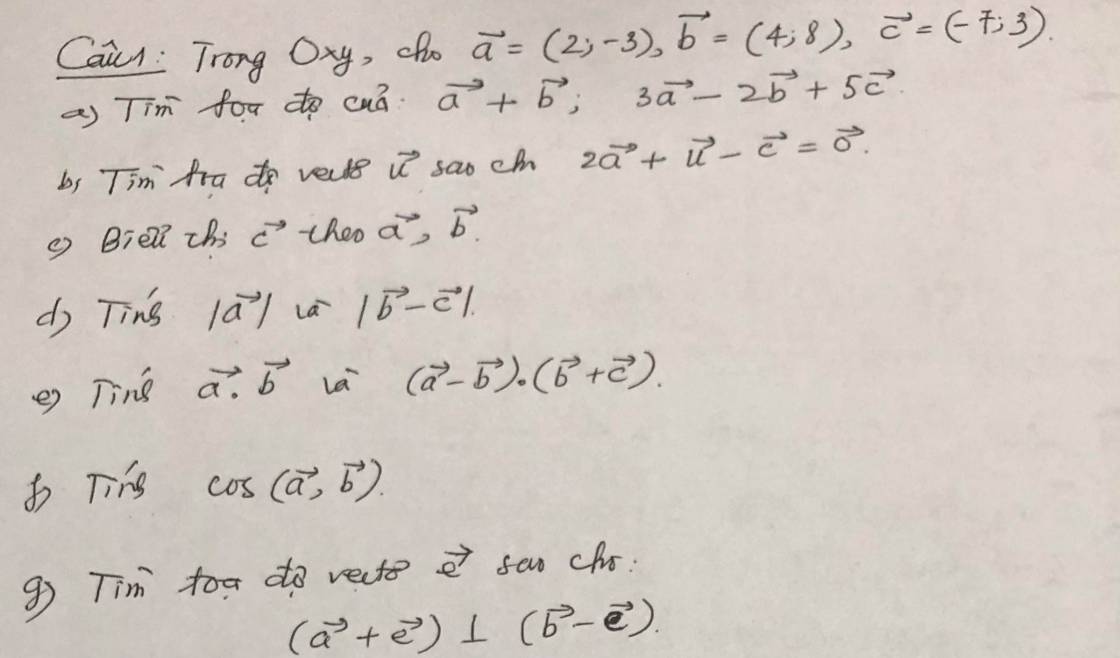
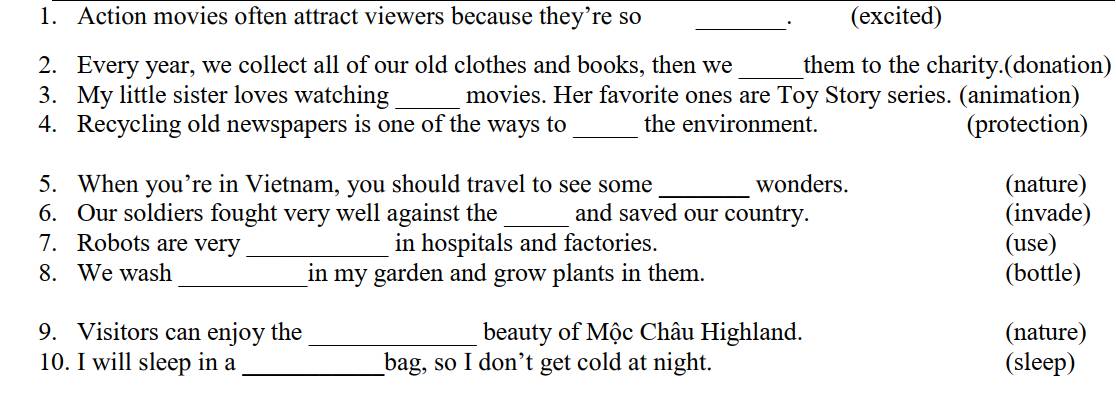
1 exciting
2 donate
3 animated
4 protect
5 natural
6 invaders
7 useful
8 bottles
9 natural
10 sleeping