mọi người giúp mình bài 1 với ạ. Mình cảm ơn nhiều ạ
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
TT
1
Những câu hỏi liên quan
NP
0
TV
Mọi người giúp mình bài này với, mình cảm ơn nhiều ạ.
2
14 tháng 12 2021
\(1,ĐK:x\ge2\\ PT\Leftrightarrow\sqrt{3x-6}+x-2-\left(\sqrt{2x-3}-1\right)=0\\ \Leftrightarrow\dfrac{3\left(x-2\right)}{\sqrt{3x-6}}+\left(x-2\right)-\dfrac{2\left(x-2\right)}{\sqrt{2x-3}+1}=0\\ \Leftrightarrow\left(x-2\right)\left(\dfrac{3}{\sqrt{3x-6}}-\dfrac{2}{\sqrt{2x-3}+1}+1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=2\left(tm\right)\\\dfrac{3}{\sqrt{3x-6}}-\dfrac{2}{\sqrt{2x-3}+1}+1=0\left(1\right)\end{matrix}\right.\)
Với \(x>2\Leftrightarrow-\dfrac{2}{\sqrt{2x-3}+1}>-\dfrac{2}{1+1}=-1\left(3x-6\ne0\right)\)
\(\Leftrightarrow\left(1\right)>0-1+1=0\left(vn\right)\)
Vậy \(x=2\)
14 tháng 12 2021
\(2,ĐK:x\ge-1\)
Đặt \(\left\{{}\begin{matrix}\sqrt{x+1}=a\\\sqrt{x^2-x+1}=b\end{matrix}\right.\left(a,b\ge0\right)\Leftrightarrow a^2+b^2=x^2+2\)
\(PT\Leftrightarrow2a^2+2b^2-5ab=0\\ \Leftrightarrow\left(a-2b\right)\left(2a-b\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}a=2b\\b=2a\end{matrix}\right.\)
Với \(a=2b\Leftrightarrow x+1=4x^2-4x+4\left(vn\right)\)
Với \(b=2a\Leftrightarrow4x+4=x^2-x+1\Leftrightarrow x^2-5x-3=0\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5+\sqrt{37}}{2}\left(tm\right)\\x=\dfrac{5-\sqrt{37}}{2}\left(tm\right)\end{matrix}\right.\)
Vậy ...
13 tháng 1 2022
a) Điện trở tương đương của đoạn mạch :
\(R_{tđ}=\dfrac{R_1.R_2}{R_1+R_2}=\dfrac{60.40}{60+40}=24\left(\Omega\right)\)
b) Hiệu điện thế giữa hai đầu đoạn mạch :
\(U=I.R_{tđ}=2.24=48\left(V\right)\)
⇒ \(U=U_1=U_2=48\left(V\right)\) (vì R1 // R2)
Cường độ dòng điện chạy qua mỗi điện trở :
\(I_1=\dfrac{U_1}{R_1}=\dfrac{48}{60}=0,8\left(A\right)\)
\(I_2=\dfrac{U_2}{R_2}=\dfrac{48}{40}=1,2\left(A\right)\)
Chúc bạn học tốt
WC
0
17 tháng 7 2021
1) Áp dụng định lí Pytago vào ΔABC vuông tại A, ta được:
\(BC^2=AB^2+AC^2\)
\(\Leftrightarrow BC^2=6^2+8^2=100\)
hay BC=10(cm)
Áp dụng hệ thức lượng trong tam giác vuông vào ΔABC vuông tại A có AH là đường cao ứng với cạnh huyền BC, ta được:
\(AH\cdot BC=AB\cdot AC\)
\(\Leftrightarrow AH\cdot10=6\cdot8=48\)
hay AH=4,8(cm)
MN
4
NK
1
NV
Nguyễn Việt Lâm
Giáo viên
7 tháng 8 2021
\(y'=3mx^2-4mx-\left(m+1\right)\)
- Với \(m=0\Rightarrow y'=-1< 0\) hàm nghịch biến trên R (thỏa)
- Với \(m\ne0\) hàm nghịch biến trên R khi:
\(\left\{{}\begin{matrix}3m< 0\\\Delta'=4m^2+3m\left(m+1\right)\le0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m< 0\\7m^2+3m\le0\\\end{matrix}\right.\)
\(\Leftrightarrow-\dfrac{3}{7}\le m< 0\)
Vậy \(-\dfrac{3}{7}\le m\le0\Rightarrow m=0\)
S có 1 phần tử
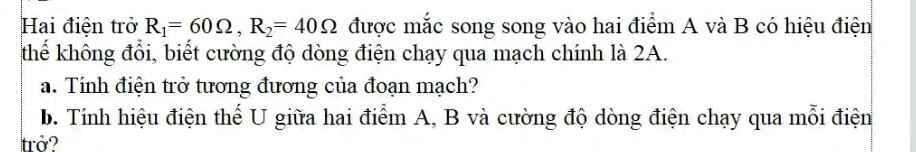
Bài 1:
a) \(\dfrac{3}{4}+\dfrac{-1}{3}\)
\(=\dfrac{9}{12}+\dfrac{-4}{12}\)
\(=\dfrac{5}{12}\)
b) \(\dfrac{4}{5}+\dfrac{9}{15}:\dfrac{1}{15}\)
\(=\dfrac{4}{5}+\dfrac{9}{15}\cdot\dfrac{15}{1}\)
\(=\dfrac{4}{5}+\dfrac{9}{1}\)
\(=\dfrac{4}{5}+\dfrac{45}{5}\)
\(=\dfrac{49}{5}\)
c) \(\dfrac{9}{2}\cdot\dfrac{4}{13}+\dfrac{4}{13}\cdot\dfrac{-5}{2}+\dfrac{4}{13}\)
\(=\dfrac{4}{13}\cdot\left(\dfrac{9}{2}-\dfrac{5}{2}+1\right)\)
\(=\dfrac{4}{13}\cdot\left(\dfrac{4}{2}+1\right)\)
\(=\dfrac{4}{13}\cdot3\)
\(=\dfrac{12}{13}\)
d) \(\dfrac{-5}{7}+\dfrac{3}{4}+\dfrac{-1}{5}+\dfrac{-2}{7}+\dfrac{1}{4}\)
\(=\left[\left(\dfrac{-5}{7}+\dfrac{-2}{7}\right)+\left(\dfrac{3}{4}+\dfrac{1}{4}\right)\right]+\dfrac{-1}{5}\)
\(=\left(\dfrac{-7}{7}+\dfrac{4}{4}\right)+\dfrac{-1}{5}\)
\(=\left(-1+1\right)-\dfrac{1}{5}\)
\(=-\dfrac{1}{5}\)