\(\dfrac{72X202X28}{303X63X14}\) mọi người chỉ chi tiết giúp em nhé
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
12 tháng 12 2020
Ta có: \(\dfrac{6^5\cdot\left(-12\right)^6}{\left(-4\right)^9\cdot\left(-3\right)^{10}}\)
\(=-\dfrac{3^5\cdot2^5\cdot12^6}{4^9\cdot3^{10}}\)
\(=-\dfrac{2^5\cdot3^6\cdot4^6}{4^9\cdot3^5}\)
\(=-\dfrac{2^5\cdot3}{4^3}\)
\(=-\dfrac{2^5}{2^6}\cdot3=-\dfrac{3}{2}\)
12 tháng 12 2020
ta được \(\dfrac{6^5.12^6}{4^8.\left(-4\right).3^{10}}\) \(=\dfrac{2^5.3^5.2^{12}.3^6}{2^{16}.\left(-4\right).3^{10}}\) \(=\dfrac{2^{17}.3^{11}}{2^{16}.\left(-4\right).3^{10}}=\dfrac{-6}{4}=\dfrac{-3}{2}\)
9 tháng 3 2022
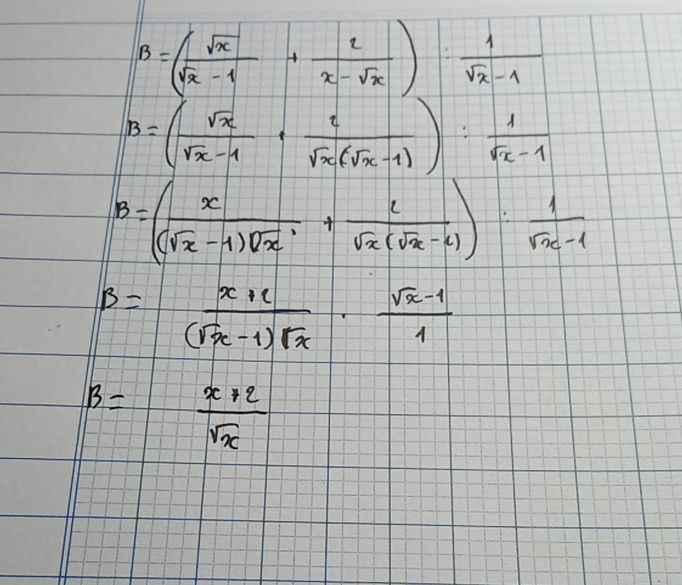
\(=\dfrac{\sqrt{x}+1}{\sqrt{x}\left(\sqrt{x}-1\right)}\cdot\dfrac{\left(\sqrt{x}-1\right)^2}{\sqrt{x}+1}=\dfrac{\sqrt{x}-1}{\sqrt{x}}\)
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
13 tháng 7 2023
2\(\sqrt{\dfrac{16}{3}}\) - 3\(\sqrt{\dfrac{1}{27}}\) - \(\dfrac{3}{2\sqrt{3}}\)
= \(\dfrac{8}{\sqrt{3}}\) - \(\dfrac{3}{3\sqrt{3}}\) - \(\dfrac{3}{2\sqrt{3}}\)
= \(\dfrac{8}{\sqrt{3}}\) - \(\dfrac{1}{\sqrt{3}}\) - \(\dfrac{3}{2\sqrt{3}}\)
= \(\dfrac{16}{2\sqrt{3}}\) - \(\dfrac{2}{2\sqrt{3}}\) - \(\dfrac{3}{2\sqrt{3}}\)
= \(\dfrac{11}{2\sqrt{3}}\)
= \(\dfrac{11\sqrt{3}}{6}\)
f, 2\(\sqrt{\dfrac{1}{2}}\)- \(\dfrac{2}{\sqrt{2}}\) + \(\dfrac{5}{2\sqrt{2}}\)
= \(\dfrac{2}{\sqrt{2}}\) - \(\dfrac{2}{\sqrt{2}}\) + \(\dfrac{5}{2\sqrt{2}}\)
= \(\dfrac{5}{2\sqrt{2}}\)
= \(\dfrac{5\sqrt{2}}{4}\)
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
13 tháng 7 2023
(1 + \(\dfrac{3-\sqrt{3}}{\sqrt{3}-1}\)).(1- \(\dfrac{3+\sqrt{3}}{\sqrt{3}+1}\))
= \(\dfrac{\sqrt{3}-1+3-\sqrt{3}}{\sqrt{3}-1}\).\(\dfrac{\sqrt{3}+1-3+\sqrt{3}}{\sqrt{3}+1}\)
= \(\dfrac{2}{\sqrt{3}-1}\).\(\dfrac{-2}{\sqrt{3}+1}\)
= \(\dfrac{-4}{3-1}\)
= \(\dfrac{-4}{2}\)
= -2
27 tháng 11 2024
đặt x^2+ax+b= (x-1)(x-m)
x^2+ax+b/x^2-1 = x-m/x+1
lim x-m/x+1=-1/2 suy ra 1-m/2=-1/2 nên m = 3
x^2+ax+b= (x-1)(x-3)=x^2-4x+3 suy ra a=-4, b=3
T
17 tháng 11 2023
\(\dfrac{4x+2}{4x-2}+\dfrac{3-6x}{6x-6}\left(dkxd:x\ne\dfrac{1}{2};x\ne1\right)\)
\(=\dfrac{2\left(2x+1\right)}{2\left(2x-1\right)}+\dfrac{3\left(1-2x\right)}{6\left(x-1\right)}\)
\(=\dfrac{2x+1}{2x-1}+\dfrac{1-2x}{2\left(x-1\right)}\)
\(=\dfrac{2x+1}{2x-1}+\dfrac{1-2x}{2x-2}\)
\(=\dfrac{\left(2x+1\right)\left(2x-2\right)}{\left(2x-1\right)\left(2x-2\right)}+\dfrac{\left(1-2x\right)\left(2x-1\right)}{\left(2x-1\right)\left(2x-2\right)}\)
\(=\dfrac{4x^2-2x-2}{\left(2x-1\right)\left(2x-2\right)}+\dfrac{-4x^2+4x-1}{\left(2x-1\right)\left(2x-2\right)}\)
\(=\dfrac{4x^2-2x-2-4x^2+4x-1}{\left(2x-1\right)\left(2x-2\right)}\)
\(=\dfrac{2x-3}{\left(2x-1\right)\left(2x-2\right)}\)
\(=\dfrac{2x-3}{4x^2-6x+2}\)
2
22 tháng 5 2023
Ptr có `2` nghiệm phân biệt `<=>\Delta' > 0`
`=>(m+1)^2-m^2+2m-3 > 0`
`<=>m^2+2m+1-m^2+2m-3 > 0`
`<=>m > 1/2`
`=>` Áp dụng Viét có: `{(x_1+x_2=-b/a=2m+2),(x_1.x_2=c/a=m^2-2m+3):}`
Ta có: `1/[x_1 ^2]-[4x_2]/[x_1]+3x_2 ^2=0`
`=>1-4x_1.x_2+3(x_1.x_2)^2=0`
`<=>1-4(m^2-2m+3)+3(m^2-2m+3)^2=0`
`<=>[(m^2-2m+3=1),(m^2-2m+3=1/3):}`
`<=>[(m^2-2m+2=0(VN)),(m^2-2m+8/3=0(VN)):}`
`=>` Không có `m` thỏa mãn.
NH
19 tháng 2 2023
\(\dfrac{x-2}{5}=\dfrac{1-x}{6}\\ =>\left(x-2\right)\cdot6=\left(1-x\right)\cdot5\\ =>6x-12=5-5x\\ =>6x+5x=5+12\\ =>11x=17\\ x=\dfrac{17}{11}\)
XO
10 tháng 8 2020
Ta có : \(\frac{3}{x-1}=\frac{4}{y-2}=\frac{5}{z-3}\Rightarrow1:\frac{3}{x-1}=1:\frac{4}{y-2}=1:\frac{5}{z-3}\)
\(\Rightarrow\frac{x-1}{3}=\frac{y-2}{4}=\frac{z-3}{5}\)
Đặt \(\frac{x-1}{3}=\frac{y-2}{4}=\frac{z-3}{5}=k\Rightarrow\hept{\begin{cases}x=3k+1\\y=4k+2\\z=5k+3\end{cases}}\)
Khi đó x + y + z = 18
<=> 3k + 1 + 4k + 2 + 5k + 3 = 18
=> 12k + 6 = 18
=> 12k = 12
=> k = 1
=> x = 4 ; y = 6 ; z = 8
F
11 tháng 8 2020
Bài giải
Áp dụng tính chất của dãy tỉ số bằng nhau, ta có :
\(\frac{3}{x-1}=\frac{4}{y-2}=\frac{5}{z-3}=\frac{3+4+5}{x-1+y-2+z-3}=\frac{12}{12}=1\)
\(\Rightarrow\text{ }\hept{\begin{cases}x=3\text{ : }1+1=4\\y=4\text{ : }1+2=6\\z=5\text{ : }1+3=8\end{cases}}\)
\(\Rightarrow\text{ }x=4\text{ ; }y=6\text{ ; }z=8\)
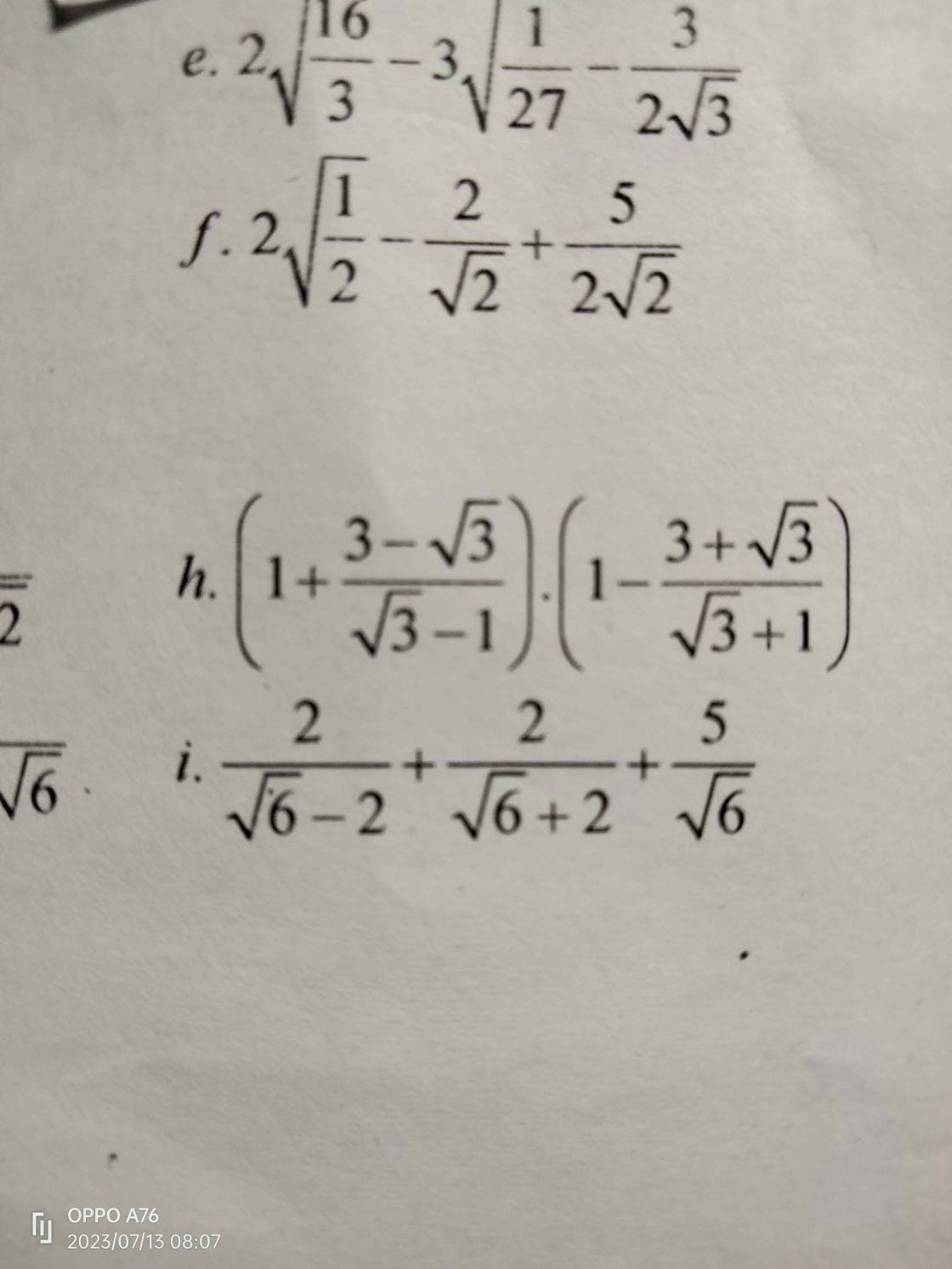
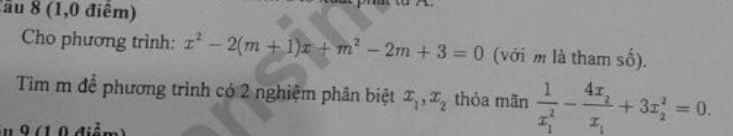
\(\dfrac{72\times202\times28}{303\times63\times14}=\dfrac{9\times8\times101\times2\times14\times2}{101\times3\times9\times7\times14}=\dfrac{8\times2\times2}{3\times7}=\dfrac{32}{21}\)
\(\dfrac{72\times202\times28}{303\times63\times14}\)
\(=\dfrac{9\times8\times2\times101\times7\times4}{101\times3\times9\times7\times2\times7}\)
\(=\dfrac{8\times4}{3\times7}=\dfrac{32}{21}\)