Làm tính chia: (2x^4-5x^3+6x-15) : (2x-5)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
12 tháng 10 2017
a)\(x^4-6x^2+2x+28\)
\(=\left(x^4-x^3\right)+\left(x^3-x^2\right)-\left(5x^2-5x\right)-\left(3x-3\right)+25\)
\(=\left(x-1\right)\left(x^3+x^2-5x-3\right)+25\)
=> số dư là 25
b) Cách làm tương tự câu a nhé
ZH
1
15 tháng 10 2021
\(\dfrac{x^4+2x^3+10x-25}{x^2+5}\)
\(=\dfrac{\left(x^2-5\right)\left(x^2+5\right)+2x\left(x^2+5\right)}{x^2+5}\)
\(=x^2+2x-5\)
J
2
29 tháng 12 2017
Ta có:
\(2x^3-5x^2+6x-15\)
\(=\left(2x^3-5x^2\right)+\left(6x-15\right)\)
\(=x^2\left(2x-5\right)+3\left(2x-5\right)\)
\(=\left(x^2+3\right)\left(2x-5\right)\)
\(\Rightarrow\left(2x^3-5x^2+6x-15\right):\left(2x-5\right)=x^2+3\)
17 tháng 11 2021
\(=\left[x^2\left(2x-5\right)+3\left(2x-5\right)\right]:\left(2x-5\right)\\ =x^2+3\)
25 tháng 5 2022
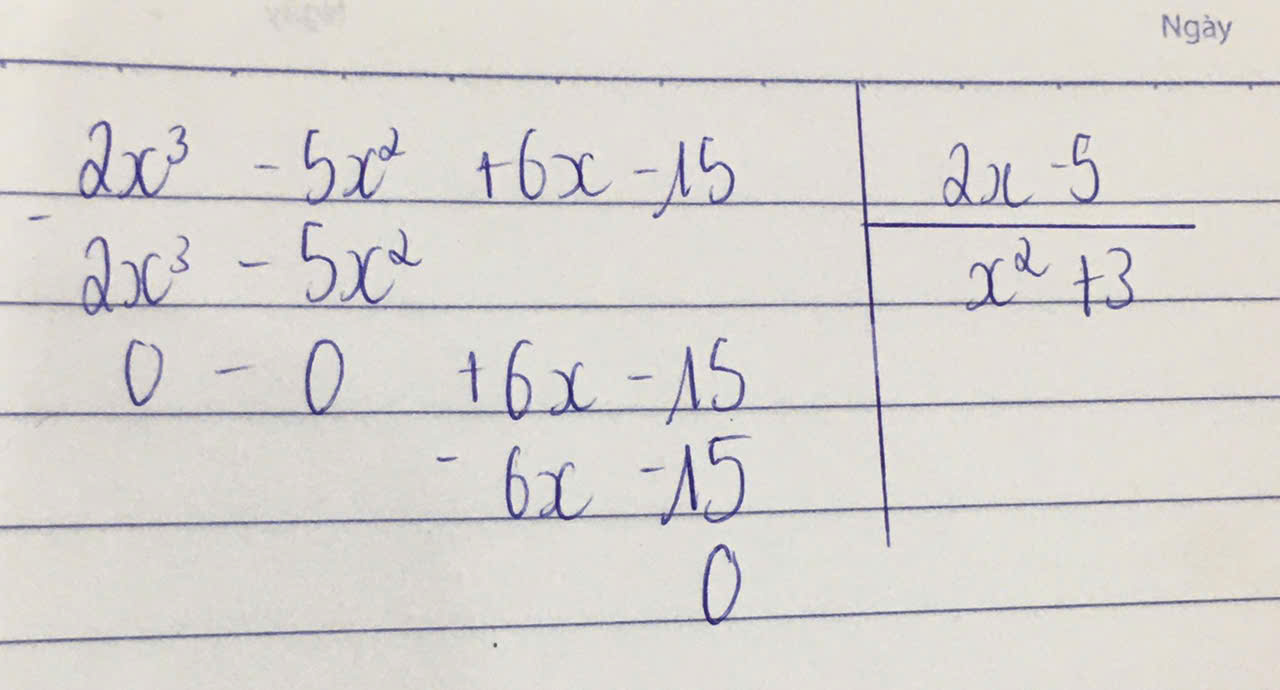
a: \(\Leftrightarrow12x^2-10x-12x^2-28x=7\)
=>-38x=7
hay x=-7/38
b: \(\Leftrightarrow-10x^2-5x+9x^2+6x+x^2-\dfrac{1}{2}x=0\)
=>1/2x=0
hay x=0
c: \(\Leftrightarrow18x^2-15x-18x^2-14x=15\)
=>-29x=15
hay x=-15/29
d: \(\Leftrightarrow x^2+2x-x-3=5\)
\(\Leftrightarrow x^2+x-8=0\)
\(\text{Δ}=1^2-4\cdot1\cdot\left(-8\right)=33>0\)
Do đó: Phương trình có hai nghiệm phân biệt là:
\(\left\{{}\begin{matrix}x_1=\dfrac{-1-\sqrt{33}}{2}\\x_2=\dfrac{-1+\sqrt{33}}{2}\end{matrix}\right.\)
e: \(\Leftrightarrow-15x^2+10x-10x^2-5x-5x=4\)
\(\Leftrightarrow-25x^2=4\)
\(\Leftrightarrow x^2=-\dfrac{4}{25}\left(loại\right)\)