Chứng minh giá trị của biểu thức ko phụ thuộc vào biến x;y (x-5)×(x+7)+(x+4)×(2-x
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
20 tháng 9 2021
2) \(P=\left(2x+1\right)\left(4x^2-2x+1\right)=8x^3+1=8.\left(\dfrac{1}{2}\right)^3+1=8.\dfrac{1}{8}+1=2\)
\(Q=\left(x+3y\right)\left(x^2-3xy+9y^2\right)=x^3+27y^3=1^3+27.\left(\dfrac{1}{3}\right)^3=1+27.\dfrac{1}{27}=2\)
3) \(\left(8x+2\right)\left(1-3x\right)+\left(6x-1\right)\left(4x-10\right)=-50\)
\(\Leftrightarrow-24x^2+2x+2+24x^2-64x+10=-50\)
\(\Leftrightarrow-62x=-62\Leftrightarrow x=1\)
TV
2
29 tháng 7 2018
(x-1)(x2+x+1)-(x+1)(x2-x+1)
=x3+x2+x-x2-x-1-(x3-x2+x+x2-x+1)
=x3+x2+x-x2-x-1-x3+x2-x-x2+x-1
=...( còn lại bn tự lm nha)
TA
2
N
14 tháng 10 2021
\(2x^2+3x-10x-15-2x^2+6x+x+7=-8\)
=> BT trên ko phụ thuộc vào biến x
14 tháng 10 2021
\(\left(x-5\right)\left(2x+3\right)-2x\left(x-3\right)+x+7\)
\(=2x^2-7x-15-2x^2+6x+x+7=-8\)
PA
19 tháng 9 2021
B=-x(x-y)-y(x+y)+(x+y)(x-y)+2y^(2)
B=-x^2+xy-yx-y^2+x^2-xy+xy-y^2+2y^2
B=0
vậu B ko phọ thuộc vào gt của biến
19 tháng 9 2021
\(B=-x\left(x-y\right)-y\left(x+y\right)+\left(x+y\right)\left(x-y\right)+2y^2\)
\(=-x^2+xy-xy-y^2+x^2-y^2+2y^2\)
=0
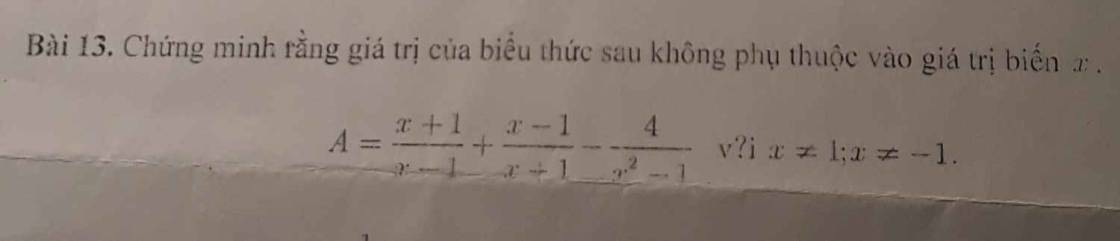
`(x -5).(x+7) + (x+4).(2-x)`
` = x^2 +2x-35 -x^2 -2x + 8`
`= (x^2 - x^2) + (2x - 2x) + (-35 +8)`
`=0 + 0 - 27`
`=-27`
`=>` Biểu thức trên không phụ thuộc vào biến `x`
\(\left(x-5\right)\left(x+7\right)+\left(x+4\right)\left(2-x\right)=x^2+2x-35+2x-x^2+8-4x=-27\)
Vậy bth ko phụ thuộc biến x