Tìm GTLN của biểu thức: x+1/2 - | x-2/3 |
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
T
3
13 tháng 4 2022
\(A=\dfrac{x^2+3}{x^2+1}=1+\dfrac{2}{x^2+1}\le1+\dfrac{2}{1}=3\)
" = " \(\Leftrightarrow x=0\)
W
2
NN
7 tháng 4 2020
\(ĐKXĐ:x\ne-1\)
\(B=\frac{3\left(x+1\right)}{x^3+x^2+x+1}\)\(\Leftrightarrow B=\frac{3\left(x+1\right)}{\left(x^3+x^2\right)+\left(x+1\right)}\)\(\Leftrightarrow B=\frac{3\left(x+1\right)}{x^2\left(x+1\right)+\left(x+1\right)}\)\(\Leftrightarrow B=\frac{3\left(x+1\right)}{\left(x+1\right)\left(x^2+1\right)}\)\(\Leftrightarrow B=\frac{3}{x^2+1}\)
Vì \(x^2\ge0\)\(\Rightarrow x^2+1\ge1\)\(\Rightarrow\frac{3}{x^2+1}\le3\)\(\Rightarrow B\le3\)
Dấu " = " xảy ra \(\Leftrightarrow x^2=0\)\(\Leftrightarrow x=0\)( thoả mãn ĐKXĐ )
Vậy \(maxB=3\)\(\Leftrightarrow x=0\)
19 tháng 4 2020
\(B=\frac{3\left(x+1\right)}{x^3+x^2+x+1}\)
\(=\frac{3\left(x+1\right)}{x^2\left(x+1\right)+1\left(x+1\right)}=\frac{3\left(x+1\right)}{\left(x^2+1\right)\left(x+1\right)}=\frac{3}{x^2+1}\)
Vì \(x^2\ge0\Rightarrow x^2+1\ge1\)
Mà \(\frac{3}{x^2+1}\le3\)Nên \(\Rightarrow B\le3\)
Dấu ''='' xảy ra <=> x = 0
Vậy \(Max_B=3\Leftrightarrow x=0\)
W
1
NN
12 tháng 4 2020
\(B=\frac{3\left(x+1\right)}{x^3+x^2+x+1}=\frac{3\left(x+1\right)}{\left(x^3+x^2\right)+\left(x+1\right)}=\frac{3\left(x+1\right)}{x^2\left(x+1\right)+\left(x+1\right)}\)
\(=\frac{3\left(x+1\right)}{\left(x^2+1\right)\left(x+1\right)}=\frac{3}{x^2+1}\)
Vì \(x^2\ge0\forall x\)\(\Rightarrow x^2+1\ge1\forall x\)
\(\Rightarrow\frac{1}{x^2+1}\le1\forall x\)\(\Rightarrow\frac{3}{x^2+1}\le3\forall x\)
hay \(B\le3\)
Dấu " = " xảy ra \(\Leftrightarrow x^2=0\)\(\Leftrightarrow x=0\)
Vậy \(maxB=3\)\(\Leftrightarrow x=0\)
18 tháng 11 2018
\(A=x^2-6x+10\)
\(\Leftrightarrow A=x^2-2\cdot x\cdot3+3^2-9+10\)
\(\Leftrightarrow A=\left(x-3\right)^2+1\ge1\) \(\forall x\in z\)
\(\Leftrightarrow A_{min}=1khix=3\)
\(B=3x^2-12x+1\)
\(\Leftrightarrow B=\left(\sqrt{3}x\right)^2-2\cdot\sqrt{3}x\cdot2\sqrt{3}+\left(2\sqrt{3}\right)^2-12+1\)
\(\Leftrightarrow B=\left(\sqrt{3}x-2\sqrt{3}\right)^2-11\ge-11\) \(\forall x\in z\)
\(\Leftrightarrow B_{min}=-11khix=2\)
DD
Đoàn Đức Hà
Giáo viên
26 tháng 6 2021
\(A=\frac{5x^2+4x-1}{x^2}=\frac{9x^2-\left(4x^2-4x+1\right)}{x^2}=9-\frac{\left(2x-1\right)^2}{x^2}\le9\)
Dấu \(=\)khi \(2x-1=0\Leftrightarrow x=\frac{1}{2}\).
\(B=\frac{x^2}{x^2+x+1}=\frac{3x^2}{3x^2+3x+3}=\frac{4x^2+4x+4-\left(x^2+4x+4\right)}{3x^2+3x+3}=\frac{4}{3}-\frac{\left(x+2\right)^2}{3\left(x^2+x+1\right)}\le\frac{4}{3}\)
Dấu \(=\)khi \(x+2=0\Leftrightarrow x=-2\).
14 tháng 3 2022
a. \(A=\left(\dfrac{2-3x}{x^2+2x-3}-\dfrac{x+3}{1-x}-\dfrac{x+1}{x+3}\right):\dfrac{3x+12}{x^3-1}\left(ĐKXĐ:x\ne1;x\ne-3\right)\)
\(=\left(\dfrac{2-3x}{\left(x-1\right)\left(x+3\right)}+\dfrac{x+3}{x-1}-\dfrac{x+1}{x+3}\right):\dfrac{3x+12}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\left(\dfrac{2-3x}{\left(x-1\right)\left(x+3\right)}+\dfrac{\left(x+3\right)^2}{\left(x-1\right)\left(x+3\right)}-\dfrac{\left(x-1\right)\left(x+1\right)}{\left(x-1\right)\left(x+3\right)}\right):\dfrac{3x+12}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\dfrac{2-3x+x^2+6x+9-x^2+1}{\left(x-1\right)\left(x+3\right)}:\dfrac{3x+12}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\dfrac{3x+12}{\left(x-1\right)\left(x+3\right)}:\dfrac{3x+12}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\dfrac{3x+12}{\left(x-1\right)\left(x+3\right)}.\dfrac{\left(x-1\right)\left(x^2+x+1\right)}{3x+12}=\dfrac{x^2+x+1}{x+3}\)
\(M=A.B=\dfrac{x^2+x+1}{x+3}.\dfrac{x^2+x-2}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{x^2+x-2}{x+3}\)
b. -Để M thuộc Z thì:
\(\left(x^2+x-2\right)⋮\left(x+3\right)\)
\(\Rightarrow\left(x^2+3x-2x-6+4\right)⋮\left(x+3\right)\)
\(\Rightarrow\left[x\left(x+3\right)-2\left(x+3\right)+4\right]⋮\left(x+3\right)\)
\(\Rightarrow4⋮\left(x+3\right)\)
\(\Rightarrow x+3\in\left\{1;2;4;-1;-2;-4\right\}\)
\(\Rightarrow x\in\left\{-2;-1;1;-4;-5;-7\right\}\)
c. \(A^{-1}-B=\dfrac{x+3}{x^2+x+1}-\dfrac{x^2+x-2}{x^3-1}\)
\(=\dfrac{x+3}{x^2+x+1}-\dfrac{x^2+x-2}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\dfrac{\left(x+3\right)\left(x-1\right)}{\left(x-1\right)\left(x^2+x+1\right)}-\dfrac{x^2+x-2}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\dfrac{x^2-x+3x-3-x^2-x+2}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\dfrac{x-1}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{1}{x^2+x+1}\)
\(=\dfrac{1}{x^2+2.\dfrac{1}{2}x+\dfrac{1}{4}+\dfrac{3}{4}}=\dfrac{1}{\left(x+\dfrac{1}{2}\right)^2+\dfrac{3}{4}}\le\dfrac{1}{\dfrac{3}{4}}=\dfrac{4}{3}\)
\(Max=\dfrac{4}{3}\Leftrightarrow x=\dfrac{-1}{2}\)
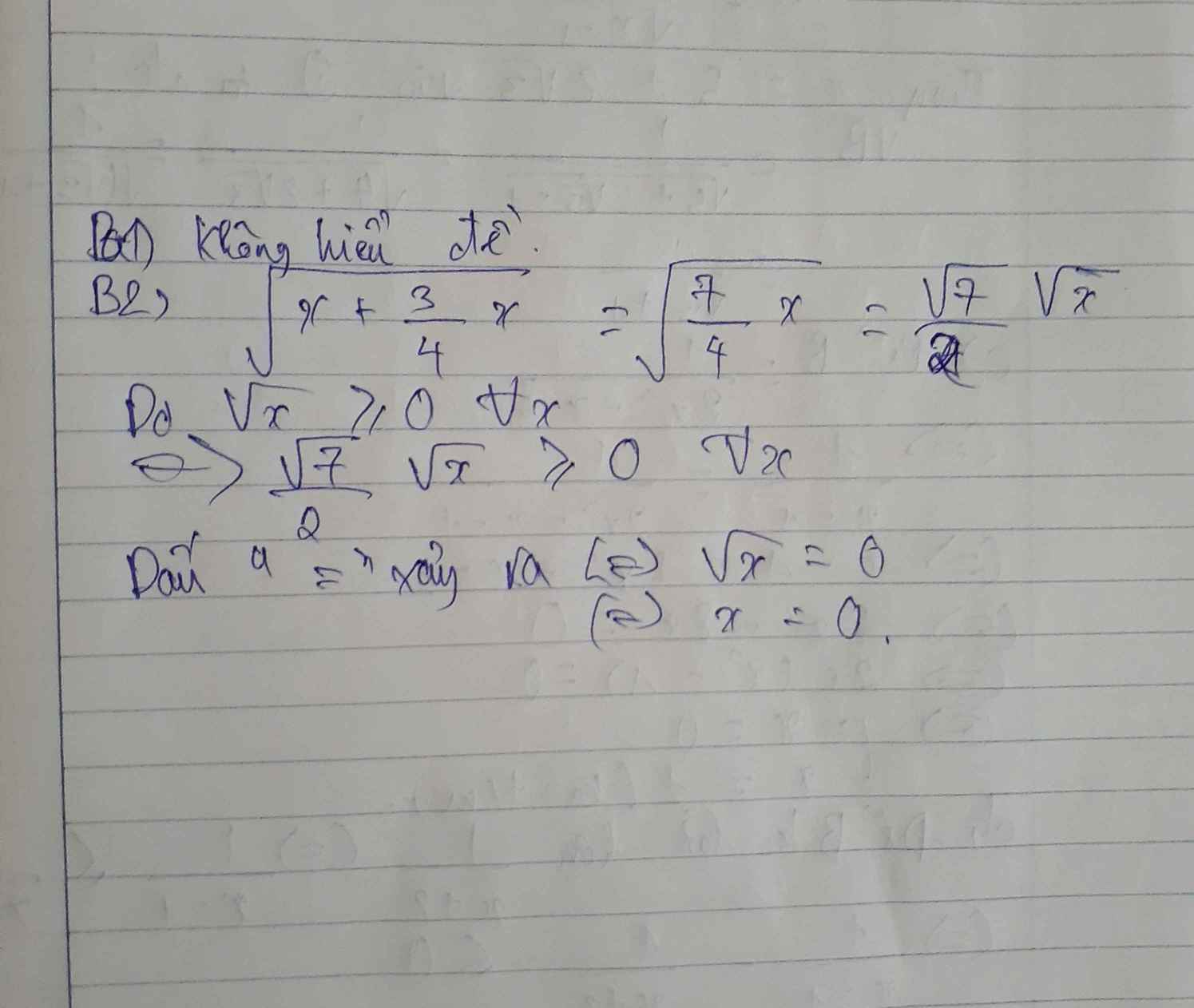
minh ko biet nha
Nho k cho minh nha
Chuc cac ban hok gioi
Bạn có tâm weeeeeee :vvvvvvvv