GTNN của: 9x2-6x+25
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
VT
1
LT
2
KV
14 tháng 5 2023
Sửa đề: Tìm GTLN của P
P = 27 - 6x - 9x²
= -(9x² + 6x + 1 - 28)
= -[(3x + 1)² - 28]
= -(3x + 1)² + 28
Do (3x + 1)² ≥ 0 với mọi x
⇒ -(3x + 1)² ≤ 0 với mọi x
⇒ -(3x + 1)² + 28 ≤ 28 với mọi x
Vậy GTLN của P là 28 khi x = -1/3
5 tháng 10 2021
Với \(x\ge\dfrac{1}{6}\Leftrightarrow A=5x^2-6x+1-1=5x^2-6x\)
\(A=5\left(x^2-2\cdot\dfrac{3}{5}x+\dfrac{9}{25}\right)-\dfrac{9}{5}=5\left(x-\dfrac{3}{5}\right)^2-\dfrac{9}{5}\ge-\dfrac{9}{5}\\ A_{min}=-\dfrac{9}{5}\Leftrightarrow x=\dfrac{3}{5}\left(1\right)\)
Với \(x< \dfrac{1}{6}\Leftrightarrow A=5x^2+6x-1-1=5x^2+6x-2\)
\(A=5\left(x^2+2\cdot\dfrac{3}{5}x+\dfrac{9}{25}\right)-\dfrac{19}{5}=5\left(x+\dfrac{3}{5}\right)^2-\dfrac{19}{5}\ge-\dfrac{19}{5}\\ A_{min}=-\dfrac{19}{5}\Leftrightarrow x=-\dfrac{3}{5}\left(2\right)\\ \left(1\right)\left(2\right)\Leftrightarrow A_{min}=-\dfrac{19}{5}\Leftrightarrow x=-\dfrac{3}{5}\)
5 tháng 10 2021
Với \(x\ge\dfrac{1}{3}\Leftrightarrow B=9x^2-6x-4\left(3x-1\right)+6=9x^2-18x+10\)
\(B=9\left(x^2-2x+1\right)+1=9\left(x-1\right)^2+1\ge1\\ B_{min}=1\Leftrightarrow x=1\left(1\right)\)
Với \(x< \dfrac{1}{3}\Leftrightarrow B=9x^2-6x+4\left(3x-1\right)+6=9x^2+6x+2\)
\(B=\left(9x^2+6x+1\right)+1=\left(3x+1\right)^2+1\ge1\\ B_{min}=1\Leftrightarrow x=-\dfrac{1}{3}\left(2\right)\)
\(\left(1\right)\left(2\right)\Leftrightarrow B_{min}=1\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-\dfrac{1}{3}\end{matrix}\right.\)
NG
a,x3+3x2+3x+1
b,x2+6x+9
c,-x3+9x2-27x+27
d,x2+4x+4
k,10x-25-x2
f,(x+y)2-9x2
g,8x3+42x2y+16xy2+6xy+y3
2
3 tháng 7 2023
a) \(x^3+3x^2+3x+1=x^2+3\cdot x^2\cdot1+3\cdot x\cdot1^2+1^3=\left(x-1\right)^3\)
b) \(x^2+6x+9=x^2+2\cdot3\cdot x+3^2=\left(x+3\right)^2\)
c) \(-x^3+9x^2-27x+27\)
\(=-\left(x^3-9x^2+27x-27\right)\)
\(=-\left(x^3-3\cdot3\cdot x^2+3\cdot3^2\cdot x-3^3\right)=-\left(x-3\right)^3\)
d) \(x^2+4x+4=x^2+2\cdot2\cdot x+2^2=\left(x+2\right)^2\)
k) \(10x-25-x^2=-x^2+10x-25=-\left(x^2-10x+25\right)\)
\(=-\left(x^2-2\cdot5\cdot x+5^2\right)=-\left(x-5\right)^2\)
f) \(\left(x+y\right)^2-9x^2=\left(x-y\right)^2-\left(3x\right)^2=\left[\left(x-y\right)-3x\right]\left[\left(x-y\right)+3x\right]\)
\(=\left(x-y-3x\right)\left(x-y+3x\right)=\left(-2x-y\right)\left(4x-y\right)\)
18 tháng 8 2021
a) \(7x\left(2x-3\right)-\left(4x^2-9\right)=0\Rightarrow7x\left(2x-3\right)-\left(2x-3\right)\left(2x+3\right)=0\Rightarrow\left(2x-3\right)\left(7x-2x+3\right)=0\Rightarrow\left[{}\begin{matrix}2x-3=0\\5x+3=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=\dfrac{3}{2}\\x=-\dfrac{3}{5}\end{matrix}\right.\)
b) \(\left(2x-7\right).\left(x-2\right)\left(x^2-4\right)=0\Rightarrow\left(2x-7\right)\left(x-2\right)^2\left(x+2\right)=0\Rightarrow\left[{}\begin{matrix}2x-7=0\\\left(x-2\right)^2=0\\x+2=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=\dfrac{7}{2}\\x=2\\x=-2\end{matrix}\right.\)
c)\(\left(9x^2-25\right)-\left(6x-10\right)=0\Rightarrow\left(3x-5\right)\left(3x+5\right)-2\left(3x-5\right)=0\Rightarrow\left(3x-5\right)\left(3x+5-2\right)=0\Rightarrow\left[{}\begin{matrix}3x-5=0\\3x+3=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=\dfrac{5}{3}\\x=1\end{matrix}\right.\)
18 tháng 8 2021
a: Ta có: \(7x\left(2x-3\right)-\left(4x^2-9\right)=0\)
\(\Leftrightarrow7x\left(2x-3\right)-\left(2x-3\right)\left(2x+3\right)=0\)
\(\Leftrightarrow\left(2x-3\right)\left(5x-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{3}{2}\\x=\dfrac{3}{5}\end{matrix}\right.\)
b: Ta có: \(\left(2x-7\right)\left(x-2\right)\left(x^2-4\right)=0\)
\(\Leftrightarrow\left(2x-7\right)\left(x-2\right)^2\cdot\left(x+2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{7}{2}\\x=2\\x=-2\end{matrix}\right.\)
c: Ta có: \(\left(9x^2-25\right)-\left(6x-10\right)=0\)
\(\Leftrightarrow\left(3x-5\right)\left(3x+5-2\right)=0\)
\(\Leftrightarrow\left(3x-5\right)\left(3x+3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5}{3}\\x=-1\end{matrix}\right.\)
13 tháng 7 2023
Ta có: \(D=9x^2+12x-1=9x^2+12x+4-3=\left(3x+2\right)^2-3\)
Mà: \(D=\left(3x+2\right)^2-3\le-3\forall x\)
Dấu "=" xảy ra
\(\Leftrightarrow\left(3x+2\right)^2=0\Leftrightarrow3x+2=0\Leftrightarrow3x=-2\Leftrightarrow x=-\dfrac{2}{3}\)
Vậy \(D_{min}=-3\Leftrightarrow x=-\dfrac{2}{3}\)
M
2
26 tháng 8 2023
\(P=\sqrt[]{9x^2-6x+1}+\sqrt[]{25-30x+9x^2}\)
\(\Leftrightarrow P=\sqrt[]{\left(3x-1\right)^2}+\sqrt[]{\left(5-3x\right)^2}\)
\(\Leftrightarrow P=\left|3x-1\right|+\left|5-3x\right|\)
\(\Leftrightarrow P=\left|3x-1\right|+\left|5-3x\right|\ge\left|3x-1+5-3x\right|=4\)
Vậy \(GTNN\left(P\right)=4\)
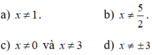
9x^2 - 6x + 25 = (3x)^2 - 6x + 1 + 24 = [ (3x)^2 - 2.3x + 1 ] + 24 = ( 3x - 1 )^2 + 24
Vì: ( 3x - 1 )^2 >= 0 với mọi x
Nên: ( 3x - 1 )^2 + 24 > 0 với mọi x
Vậy: GTNN của ( 3x - 1 )^2 + 24 là 24 <=> 3x - 1 = 0 => x = 1/3
Chúc bạn học tốt
9x^2 - 6x + 25 = (3x)^2 - 6x + 1 + 24 = [ (3x)^2 - 2.3x + 1 ] + 24 = ( 3x - 1 )^2 + 24
Vì: ( 3x - 1 )^2 >= 0 với mọi x
Nên: ( 3x - 1 )^2 + 24 > 0 với mọi x
Vậy: GTNN của ( 3x - 1 )^2 + 24 là 24 <=> 3x - 1 = 0 => x = 1/3