x3-16x=0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NB
1
TA
26 tháng 4 2023
a) \(x^3-16x=0\)
⇔\(x\left(x^2-16\right)=0\)
⇒\(x=0\) hoặc \(x^2-16=0\)
\(TH_1:x=0\)
\(TH_2:x^2-16=0\) ⇔ \(x^2=16\) ⇔ \(x=\pm4\)
Vậy \(x\in\left\{0;\pm4\right\}\)
b) \(\left(2x+1\right)^2-\left(x-1\right)^2=0\)
⇒ \(2x+1=x-1\)
⇒ \(2x+2=x\)
⇒ \(2\left(x+1\right)=x\) ⇒ x = -2
Vậy x = -2
HN
2
27 tháng 10 2021
\(a,\Leftrightarrow x\left(x+9\right)=0\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-9\end{matrix}\right.\\ b,\Leftrightarrow\left(x+4-4\right)\left(x+4+4\right)=0\\ \Leftrightarrow x\left(x+8\right)=0\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-8\end{matrix}\right.\\ c,\Leftrightarrow x\left(x-4\right)\left(x+4\right)=0\Leftrightarrow\left[{}\begin{matrix}x=0\\x=4\\x=-4\end{matrix}\right.\\ d,\Leftrightarrow\left(x-5\right)^2=0\Leftrightarrow x=5\)
27 tháng 10 2021
a) \(\Leftrightarrow x\left(x+9\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x=-9\end{matrix}\right.\)
b) \(\Leftrightarrow x\left(x+8\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x=-8\end{matrix}\right.\)
c) \(\Leftrightarrow x\left(x-4\right)\left(x+4\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x=4\\x=-4\end{matrix}\right.\)
d) \(\Leftrightarrow\left(x-5\right)^2=0\\ \Leftrightarrow x=5\)
LH
2
28 tháng 7 2019
1) \(x^3-x=0\)
\(\Leftrightarrow x.\left(x^2-1\right)=0\)
\(\)\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x^2-1=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=1\\x=-1\end{matrix}\right.\)
Vậy :.....
2) \(x^3-16x=0\)
\(\Leftrightarrow x.\left(x^2-16\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x^2-16=0\Rightarrow x^2=16\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=8\\x=-8\end{matrix}\right.\)
Vậy :....
22 tháng 10 2021
3: \(x^3+3x^2-16x-48\)
\(=x^2\left(x+3\right)-16\left(x+3\right)\)
\(=\left(x+3\right)\left(x-4\right)\left(x+4\right)\)
ST
3
5 tháng 9 2021
\(x^2y-x^3-16y+16x=\left(x^2y-x^3\right)-\left(16y-16x\right)=x^2\left(y-x\right)-16\left(y-x\right)=\left(x^2-16\right)\left(y-x\right)=\left(x-4\right)\left(x+4\right)\left(y-x\right)\)
5 tháng 9 2021
\(x^2y-x^3-16y+16x=-x^2\left(x-y\right)+16\left(x-y\right)=\left(x-y\right)\left(16-x^2\right)=\left(x-y\right)\left(4-x\right)\left(4+x\right)\)
15 tháng 10 2021
c: \(x^4+x^3-4x^2+x+1\)
\(=x^4-x^3+2x^3-2x^2-2x^2+2x-x+1\)
\(=\left(x-1\right)\left(x^3+2x^2-2x-1\right)\)
\(=\left(x-1\right)\left[\left(x-1\right)\left(x^2+x+1\right)+2x\left(x-1\right)\right]\)
\(=\left(x-1\right)^2\cdot\left(x^2+3x+1\right)\)
CM
10 tháng 7 2017
a) TXĐ: R
y′ = 6x − 24 x 2 = 6x(1 − 4x)
y' = 0 ⇔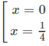 
y' > 0 trên khoảng (0; 1/4) , suy ra y đồng biến trên khoảng (0; 1/4)
y' < 0 trên các khoảng ( - ∞ ; 0 ); (14; + ∞ ), suy ra y nghịch biến trên các khoảng ( - ∞ ;0 ); (14; + ∞ )
b) TXĐ: R
y′ = 16 + 4x − 16 x 2 − 4 x 3 = −4(x + 4)( x 2 − 1)
y' = 0 ⇔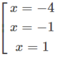 
Bảng biến thiên:
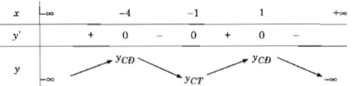
Vậy hàm số y đã cho đồng biến trên các khoảng ( - ∞ ; -4) và (-1; 1), nghịch biến trên các khoảng (-4; -1) và (1; + ∞ )
c) TXĐ: R
y′ = 3 x 2 − 12x + 9
y' = 0
y' > 0 trên các khoảng ( - ∞ ; 1), (3; + ∞ ) nên y đồng biến trên các khoảng ( - ∞ ; 1), (3; + ∞ )
y'< 0 trên khoảng (1; 3) nên y nghịch biến trên khoảng (1; 3)
d) TXĐ: R
y′ = 4 x 3 + 16 = 4x( x 2 + 4)
y' = 0 ⇔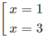 
y' > 0 trên khoảng (0; + ∞ ) ⇒ y đồng biến trên khoảng (0; + ∞ )
y' < 0 trên khoảng ( - ∞ ; 0) ⇒ y nghịch biến trên khoảng ( - ∞ ; 0)
\(x^3-16x=0\)
\(\left(x^2-16\right)x=0\)
Th1: \(x=0\)
Th2: \(x^2-16=0\)
\(x^2=16\)
\(x=+-4\)
Vậy x=-4; 0; 4
\(x^3-16x=0\)
\(=>x\left(x^2-16\right)=0\)
\(=>\orbr{\begin{cases}x=0\\x^2-16=0\end{cases}}\)
\(=>\orbr{\begin{cases}x=0\\x=+-4\end{cases}}\)