Giúp mk vs Đag gấp
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NV
Nguyễn Việt Lâm
Giáo viên
19 tháng 4 2022
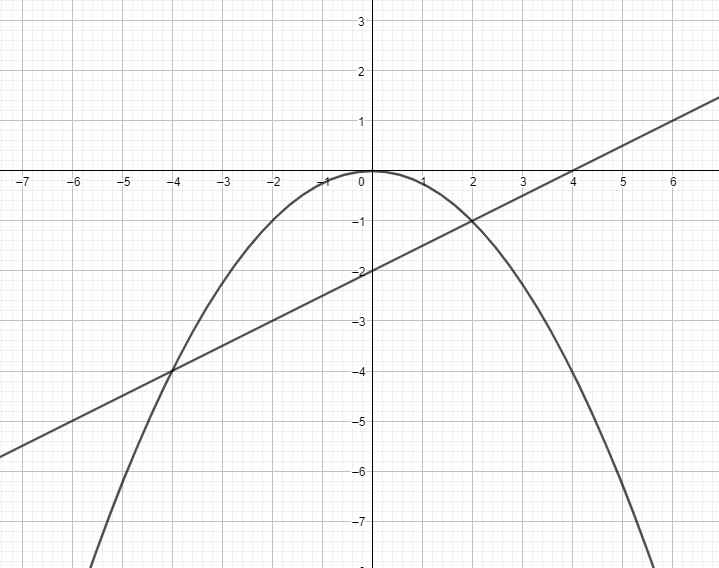
b.
Phương trình hoành độ giao điểm (P) và (d):
\(-\dfrac{1}{4}x^2=\dfrac{1}{2}x-2\Leftrightarrow x^2+2x-8=0\)
\(\Leftrightarrow\left(x-2\right)\left(x+4\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=2\Rightarrow1=-1\\x=-4\Rightarrow y=-4\end{matrix}\right.\)
Vậy (P) và (d) cắt nhau tại 2 điểm có tọa độ là (2;-1) là (-4;-4)
NH
0
TL
1
19 tháng 5 2021
Part 1
1 into
2 which
3 happily
4 aspect
5 if
6 compulsory
Part 2
1c 2f 3b 4g 5a 6e
Part 3
1 opened
2 to be finished
3 repairing
4 driving
5 harmful
6 modernize
7 environmentalists
8 effectively
Part 4
1 disappointing -> disappointed
2 come -> came
Part 5
1 succeed although he tried
2 my father could speak
3 seen such an interesting
4 being made to protect
Part 6
1 forest
2 climate
3 in
4 fortunatelt
Part 7
1T 2F 3F 4F
TN
0
NT
1
3 tháng 7 2021
a) Ta có: \(P=\dfrac{a\sqrt{a}-1}{a-\sqrt{a}}-\dfrac{a\sqrt{a}+1}{a+\sqrt{a}}+\left(\sqrt{a}-\dfrac{1}{\sqrt{a}}\right)\left(\dfrac{3\sqrt{a}}{\sqrt{a}-1}-\dfrac{\sqrt{a}+2}{\sqrt{a}+1}\right)\)
\(=\dfrac{\left(\sqrt{a}-1\right)\left(a+\sqrt{a}+1\right)}{\sqrt{a}\left(\sqrt{a}-1\right)}-\dfrac{\left(\sqrt{a}+1\right)\left(a-\sqrt{a}+1\right)}{\sqrt{a}\left(\sqrt{a}+1\right)}+\dfrac{a-1}{\sqrt{a}}\cdot\dfrac{3\sqrt{a}\left(\sqrt{a}+1\right)-\left(\sqrt{a}+2\right)\left(\sqrt{a}-1\right)}{\left(\sqrt{a}-1\right)\left(\sqrt{a}+1\right)}\)
\(=\dfrac{a+\sqrt{a}+1-a+\sqrt{a}-1}{\sqrt{a}}+\dfrac{3a+3\sqrt{a}-\left(a-\sqrt{a}+2\sqrt{a}-2\right)}{\sqrt{a}}\)
\(=2+\dfrac{3a+3\sqrt{a}-a+\sqrt{a}-2\sqrt{a}+2}{\sqrt{a}}\)
\(=\dfrac{2\sqrt{a}+2a+2\sqrt{a}+2}{\sqrt{a}}\)
\(=\dfrac{2\left(a+2\sqrt{a}+1\right)}{\sqrt{a}}\)
\(=\dfrac{2\left(\sqrt{a}+1\right)^2}{\sqrt{a}}\)
b) Ta có: \(P-6=\dfrac{2\left(\sqrt{a}+1\right)^2-6\sqrt{a}}{\sqrt{a}}\)
\(=\dfrac{2a+4\sqrt{a}+2-6\sqrt{a}}{\sqrt{a}}\)
\(=\dfrac{2\left(a-\sqrt{a}+1\right)}{\sqrt{a}}>0\forall a\) thỏa mãn ĐKXĐ
hay P>6
N
d
1
NV
Nguyễn Việt Lâm
Giáo viên
28 tháng 3 2021
Đặt \(log_2x=t\Rightarrow t\ge4\)
Phương trình trở thành: \(\sqrt{t^2-2t-3}=m\left(t-3\right)\)
\(\Leftrightarrow\sqrt{\left(t+1\right)\left(t-3\right)}=m\left(t-3\right)\)
\(\Leftrightarrow\sqrt{t+1}=m\sqrt{t-3}\)
\(\Leftrightarrow m=\sqrt{\dfrac{t+1}{t-3}}\)
Hàm \(f\left(t\right)=\sqrt{\dfrac{t+1}{t-3}}\) nghịch biến khi \(t\ge4\)
\(\lim\limits_{t\rightarrow+\infty}\sqrt{\dfrac{t+1}{t-3}}=1\) ; \(f\left(4\right)=\sqrt{5}\)
\(\Rightarrow1< f\left(t\right)\le\sqrt{5}\Rightarrow1< m\le\sqrt{5}\)
Đáp án D
NH
0
HP
2
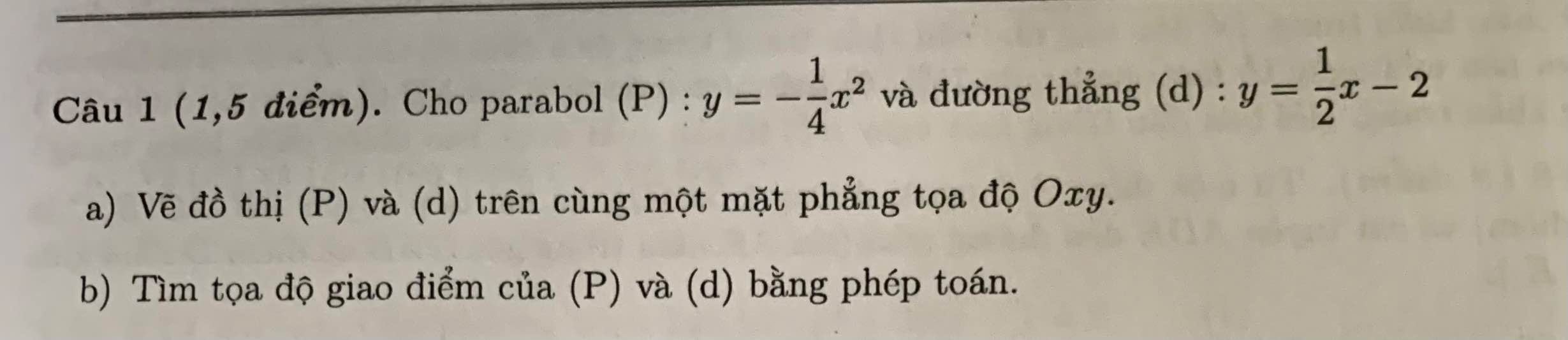
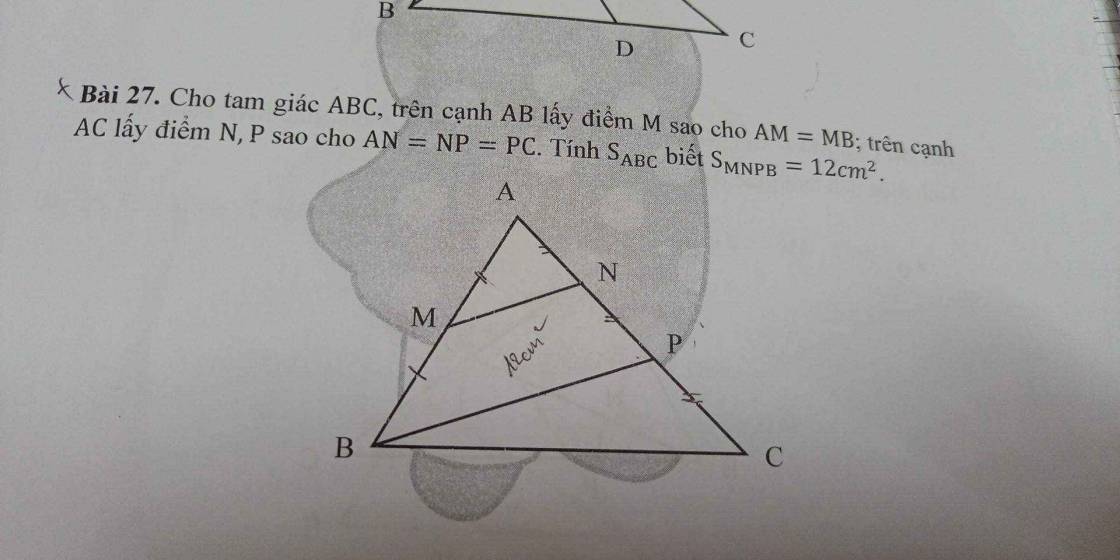
Dài qa nên jup 10 câu thoi nha =))
higher => highest
gives => gave
pleasing => pleased
lacing => to lace
quite so => bỏ quite
to turn on => turning on
would rather live on => would rather to live on
open for => open to
in => from
were => was
Giúp mk làm nốt đc ko bạn ?😢