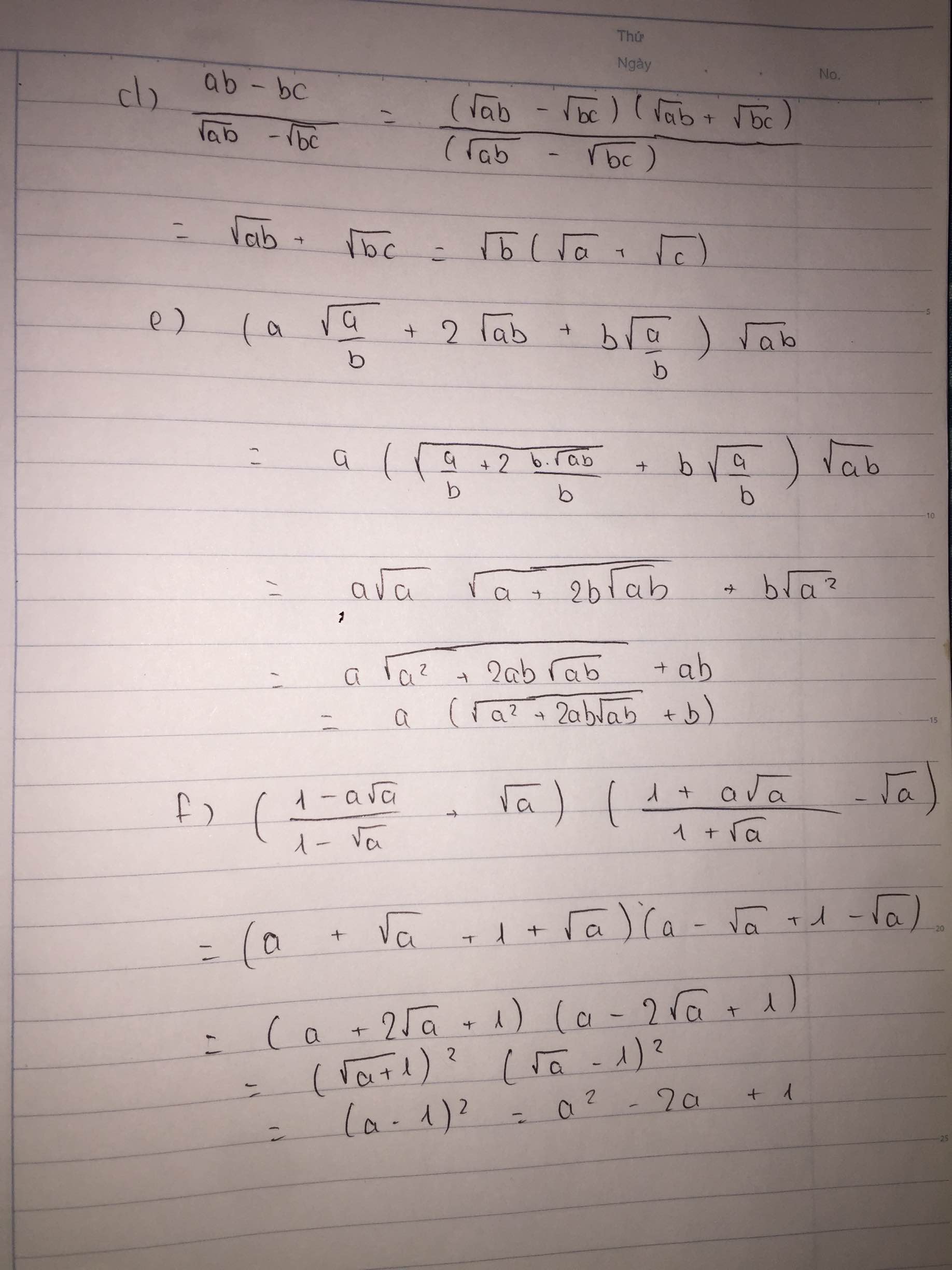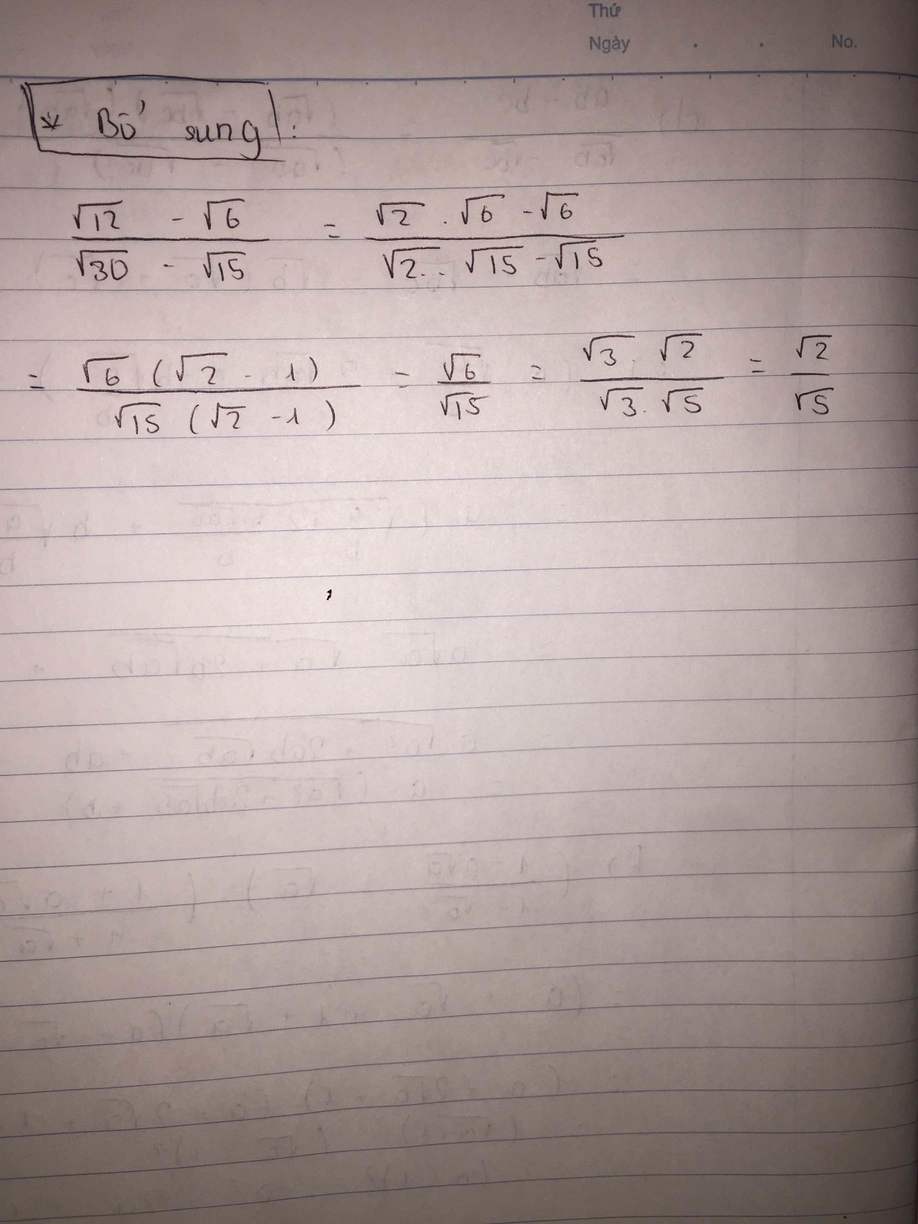Mọi người giúp em câu b) 3, 4, 5 với ạ. Em cảm ơn nhiều
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


1, \(\left\{{}\begin{matrix}4x+2y=24\\7x-2y=31\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}11x=55\\y=12-2x\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=5\\y=2\end{matrix}\right.\)
2, thiếu đề
4, \(\left\{{}\begin{matrix}4x-y-24=10x-4y\\3y-2=4-x+y\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-6x+3y=24\\x+2y=6\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-6x+3y=24\\-6x-12y=-36\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}15y=60\\x=6-2y\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=4\\x=-2\end{matrix}\right.\)


Bài 3:
Gọi K là giao của AH và BC thì AK là đường cao thứ 3 (H là trực tâm)
Vì \(\widehat{BDC}=\widehat{BEC}=90^0\) nên BEDC nội tiếp
Lại có \(BI=IC=ID=IE=\dfrac{1}{2}BC\) (trung tuyến ứng cạnh huyền) nên I là tâm đg tròn ngoại tiếp BDEC
Gọi G là trung điểm AH thì \(AG=GD=DE=\dfrac{1}{2}AH\) (trung tuyến ứng ch)
Do đó G là tâm () ngoại tiếp tg ADE
Vì \(GA=GD\Rightarrow\widehat{DAG}=\widehat{GDA}\)
Vì \(ID=IB\Rightarrow\widehat{ABI}=\widehat{IDB}\)
Do đó \(\widehat{IDB}+\widehat{GDA}=\widehat{DAG}+\widehat{ABI}=90^0\left(\Delta AKB\perp K\right)\)
Do đó \(\widehat{IDG}=180^0-\left(\widehat{IDB}+\widehat{GDA}\right)=90^0\)
Vậy \(ID\perp IG\) hay ...

Gọi 3 số nguyêntố đó là: a, b, c
Ta có: 5(a+b+c)
=>abc chia hết cho 5, do a,b,c nguyên tố
=>chỉ có trường hợp 1 trong 3 số bằng 5, giả sử a=5
=>bc=b+c+5=>(b-1)(c-1)=6
trương hợp 1: b - 1 = 1=>b=2;c - 1 = 6=>c=7
trường hợp 2: b - 1= 2, c - 1 = 3 =>c=4(loại)
vậy 3 số nguyên tố đó là: 2;5;7

Câu 16:
PTHH: \(Cl_2+2NaOH\rightarrow NaCl+NaClO+H_2O\)
Ta có: \(\left\{{}\begin{matrix}n_{Cl_2}=\dfrac{33,6}{22,4}=1,5\left(mol\right)\\n_{NaOH}=\dfrac{600\cdot20\%}{40}=3\left(mol\right)\end{matrix}\right.\) \(\Rightarrow\) 2 chất p/ứ hết
Mặt khác: \(m_{Cl_2}=1,5\cdot71=106,5\left(g\right)\)
\(\Rightarrow m_{nướcjaven}=m_{Cl_2}+m_{ddNaOH}=706,5\left(g\right)\)

\(b,\dfrac{\sqrt{12}-\sqrt{6}}{\sqrt{30}-\sqrt{15}}=\dfrac{\sqrt{6}\left(\sqrt{2}-1\right)}{\sqrt{15}\left(\sqrt{2}-1\right)}=\dfrac{\sqrt{6}}{\sqrt{15}}=\dfrac{\sqrt{2}}{\sqrt{5}}\)
\(d,\dfrac{ab-bc}{\sqrt{ab}-\sqrt{bc}}=\dfrac{\left(\sqrt{ab}-\sqrt{bc}\right)\left(\sqrt{ab}+\sqrt{bc}\right)}{\left(\sqrt{ab}-\sqrt{bc}\right)}=\sqrt{ab}+\sqrt{bc}=\sqrt{b}\left(\sqrt{a}+\sqrt{c}\right)\)
\(e,\left(a\sqrt{\dfrac{a}{b}+2\sqrt{ab}}+b\sqrt{\dfrac{a}{b}}\right)\sqrt{ab}\)
\(=a\left(\sqrt{\dfrac{a}{b}+\dfrac{2b.\sqrt{ab}}{b}}+b\sqrt{\dfrac{a}{b}}\right)\sqrt{ab}\)
\(=a\sqrt{a}\sqrt{a+2b\sqrt{ab}}+b\sqrt{a^2}\)
\(=a\sqrt{a^2+2ab\sqrt{ab}}+ab\)
\(=a\left(\sqrt{a^2+2ab\sqrt{ab}}+b\right)\)
\(f,\left(\dfrac{1-a\sqrt{a}}{1-\sqrt{a}}+\sqrt{a}\right)\left(\dfrac{1+a\sqrt{a}}{1+\sqrt{a}}-\sqrt{a}\right)\)
\(=\left(a+\sqrt{a}+1+\sqrt{a}\right)\left(a-\sqrt{a}+1-\sqrt{a}\right)\)
\(=\left(a+2\sqrt{a}+1\right)\left(a-2\sqrt{a}+1\right)\)
\(=\left(\sqrt{a}+1\right)^2\left(\sqrt{a}-1\right)^2\)
\(=\left(a-1\right)^2=a^2-2a+1\)

a) Có: `1+tan^2a=1/(cos^2a)`
`<=> 1+(3/5)^2=1/(cos^2a)`
`=> cosa=\sqrt10/4`
`=> sina = \sqrt(1-cos^2a) = \sqrt6/4`
b) Có: `sin^2a + cos^2a=1`
`<=> sin^2a + (1/4)^2=1`
`=> sina=\sqrt15/4`
`=> tana = (sina)/(cosa) = \sqrt15`
Má ơi,tính sai:
a)\(\left[{}\begin{matrix}cos\alpha=\dfrac{5\sqrt{34}}{34}\\cos\alpha=\dfrac{-5\sqrt{34}}{34}\end{matrix}\right.\)\(\Rightarrow\left[{}\begin{matrix}sin\alpha=cos\alpha.tan\alpha=\dfrac{3\sqrt{34}}{34}\\sin\alpha=cos\alpha.tan\alpha=\dfrac{-3\sqrt{34}}{34}\end{matrix}\right.\)
b)\(\left[{}\begin{matrix}sin\alpha=\dfrac{\sqrt{15}}{4}\\sin\alpha=\dfrac{-\sqrt{15}}{4}\end{matrix}\right.\)\(\left[{}\begin{matrix}tan\alpha=\dfrac{sin\alpha}{cos\alpha}=\sqrt{15}\\tatn\alpha=-\sqrt{15}\end{matrix}\right.\)





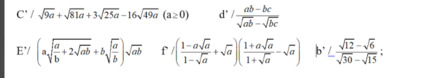 Giusp em với mọi người ạ. Rút gọn câu d, e, f, b giúp em với ạ, Em cảm ơn nhiều lắm.
Giusp em với mọi người ạ. Rút gọn câu d, e, f, b giúp em với ạ, Em cảm ơn nhiều lắm.