Giải bất phương trình: (x – 3)2 < x2 – 3
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
PT
1
CM
28 tháng 12 2019
Ta có: 3(x – 2)(x + 2) < 3 x 2 + x
⇔ 3( x 2 – 4) < 3 x 2 + x
⇔ 3 x 2 – 12 < 3 x 2 + x
⇔ 3 x 2 – 3 x 2 – x < 12
⇔ -x < 12
⇔ x > -12
Vậy tập nghiệm của bất phương trình là S = {x|x > -12}
B
Bài 1: Giải các bất phương trình sau
a) x+1/x+3 > 1
b) 2x-1/x-3 ≤ 2
c) x2+2x+2/x2+3 ≥ 1
d) 2x+1/x2+2 ≥ 1
2
13 tháng 4 2021
a, \(\dfrac{x+1}{x+3}>1\Leftrightarrow\dfrac{x+1}{x+3}-1>0\Leftrightarrow\dfrac{x+1-x-3}{x+3}>0\)
\(\Rightarrow x+3< 0\)do -2 < 0
\(\Rightarrow x< -3\)Vậy tập nghiệm BFT là S = { x | x < -3 }
b, \(\dfrac{2x-1}{x-3}\le2\Leftrightarrow\dfrac{2x-1}{x-3}-2\le0\Leftrightarrow\dfrac{2x-1-2x+6}{x-3}\le0\)
\(\Rightarrow x-3\le0\)do 5 > 0
\(\Rightarrow x\le3\)Vậy tập nghiệm BFT là S = { x | x \(\le\)3 }
c, \(\dfrac{x^2+2x+2}{x^2+3}\ge1\Leftrightarrow\dfrac{x^2+2x+2}{x^2+3}-1\ge0\)
\(\Leftrightarrow\dfrac{x^2+2x+2-x^2-3}{x^2+3}\ge0\Rightarrow2x-1\ge0\)do x^2 + 3 > 0
\(\Rightarrow x\ge\dfrac{1}{2}\)Vậy tập nghiệm BFT là S = { x | x \(\ge\)1/2 }
13 tháng 4 2021
mình ko chắc nên mình đăng sau :>
d, \(\dfrac{2x+1}{x^2+2}\ge1\Leftrightarrow\dfrac{2x+1}{x^2+2}-1\ge0\Leftrightarrow\dfrac{2x+1-x^2-2}{x^2+2}\ge0\)
\(\Rightarrow-x^2+2x-1\ge0\Rightarrow-\left(x-1\right)^2\ge0\)vô lí
23 tháng 3 2022
Tham Khảo nào
a) Điều kiện: x + 2 ≠ 0 và x – 2 ≠ 0 ⇔ x ≠ ± 2
(Khi đó: x2 – 4 = (x + 2)(x – 2) ≠ 0)
Vậy tập nghiệm của pt là: S = {-1; 1}
b) Điều kiện: 2x ≥ 0 ⇔ x ≥ 0
Khi đó: |x – 5| = 2x ⇔ x – 5 = 2x hoặc x – 5 = -2x
⇔ x = -5 hoặc x = 5/3
Vì x ≥ 0 nên ta lấy x = 5/3 . Tập nghiệm : S = {5/3}
c) x – 2)2 + 2(x – 1) ≤ x2 + 4
⇔ x2 – 4x + 4 + 2x – 2 ≤ x2 + 4
⇔ -2x ≤ 2
⇔ x ≥ -1
Tập nghiệm S = {x | x ≥ -1}
MV
1
NN
1
AH
Akai Haruma
Giáo viên
21 tháng 2 2021
Lời giải:
BPT \(\Leftrightarrow \left\{\begin{matrix} x+3\geq 0\\ (x^2+4x+3)^2\leq (x+3)^2\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x\geq -3\\ (x+1)^2(x+3)^2\leq (x+3)^2\end{matrix}\right.\)
\(\Leftrightarrow \left\{\begin{matrix} x\geq -3\\ (x+1)^2\leq 1\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x\geq -3\\ x(x+2)\leq 0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x\geq -3\\ -2\leq x\leq 0\end{matrix}\right.\)
\(\Rightarrow -2\leq x\leq 0\)
10 tháng 4 2018
Ta có:\(\left(x-3\right)\left(x+3\right)< x\left(x+2\right)+3\)
\(\Leftrightarrow x^2-9< x^2+2x+3\)
\(\Leftrightarrow x^2-x^2-2x< 3+9\)
\(\Leftrightarrow-2x< 12\)
\(\Leftrightarrow x>-6\)
Vậy tập nghiệm của BPT (1) là \(S=\left\{x\in R|x>-6\right\}\)
15 tháng 5 2023
a: =>4x^2-24x+36-4x^2+4x-1<10
=>-20x<10-35=-25
=>x>=5/4
b: =>x(x^2-25)-x^3-8<=3
=>x^3-25x-x^3-8<=3
=>-25x<=11
=>x>=-11/25
20 tháng 9 2020
1) \(\frac{x-1}{x+3}-\frac{x}{x-3}=\frac{4x+15}{9-x^2}\)
ĐKXĐ : \(x\ne\pm3\)
\(\Leftrightarrow\frac{x-1}{x+3}-\frac{x}{x-3}=\frac{-4x-15}{x^2-9}\)
\(\Leftrightarrow\frac{\left(x-1\right)\left(x-3\right)}{\left(x-3\right)\left(x+3\right)}-\frac{x\left(x+3\right)}{\left(x-3\right)\left(x+3\right)}=\frac{-4x-15}{\left(x-3\right)\left(x+3\right)}\)
\(\Leftrightarrow\frac{x^2-4x+3}{\left(x-3\right)\left(x+3\right)}-\frac{x^2+3x}{\left(x-3\right)\left(x+3\right)}=\frac{-4x-15}{\left(x-3\right)\left(x+3\right)}\)
\(\Leftrightarrow\frac{x^2-4x+3-x^2-3x}{\left(x-3\right)\left(x+3\right)}=\frac{-4x-15}{\left(x-3\right)\left(x+3\right)}\)
\(\Leftrightarrow-7x+3=-4x-15\)
\(\Leftrightarrow-7x+4x=-15-3\)
\(\Leftrightarrow-3x=-18\)
\(\Leftrightarrow x=6\)( tmđk )
Vậy x = 6 là nghiệm của phương trình
2) 2x + 3 < 6 - ( 3 - 4x )
<=> 2x + 3 < 6 - 3 + 4x
<=> 2x - 4x < 6 - 3 - 3
<=> -2x < 0
<=> x > 0
Vậy nghiệm của bất phương trình là x > 0
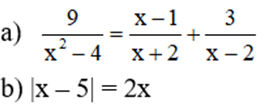
(x – 3)2 < x2 – 3
⇔ x2 – 6x + 9 < x2 – 3
⇔ x2 – 6x – x2 < -3 – 9
⇔ -6x < -12
⇔ x > 2 (Chia cả hai vế cho -6 < 0, BPT đổi chiều)
Vậy BPT có nghiệm x > 2.