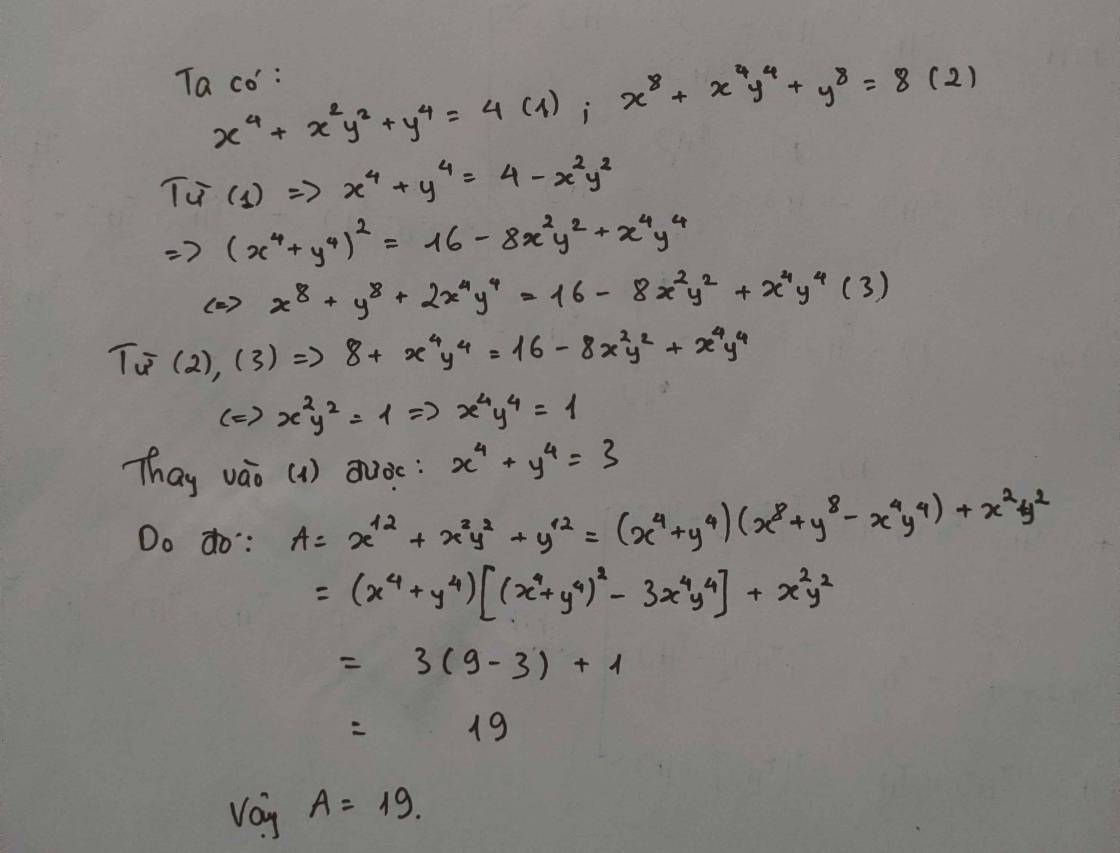Cho biểu thức với x 0;x 4; x 9.
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

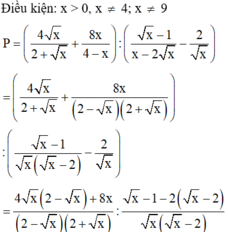
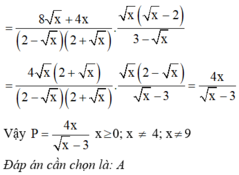

\(P=\left(x-y\right)\left(x^2+y^2\right)\left(x^4+y^4\right)-x^8+y^8+1\)
\(\Leftrightarrow P=\left(x-y\right)\left(x+y\right)\left(x^2+y^2\right)\left(x^4+y^4\right)-x^8+y^8+1\) (Vì: \(x-y=1\))
\(\Leftrightarrow P=\left(x^2-y^2\right)\left(x^2+y^2\right)\left(x^4+y^4\right)-x^8+y^8+1\)
\(\Leftrightarrow P=\left(x^4-y^4\right)\left(x^4+y^4\right)-x^8+y^8+1\)
\(\Leftrightarrow P=x^8-y^8-x^8+y^8+1\)
\(\Leftrightarrow P=1\)

Bài 2:
\(A=\left(x+y\right)^3-3xy\left(x+y\right)+3xy=1^3-3xy+3xy=1\)
Bài 3:
\(M=x^6-x^4-x^4+x^2+x^3-x\)
\(=x^3\left(x^3-x\right)-x\left(x^3-x\right)+\left(x^3-x\right)\)
\(=8x^3-8x+8\)
\(=8\cdot8+8=72\)

Từ x8+x4y4+y8=(x4+y4)2-x4y4=(x4+y4-x2y2) (x4+y4+x2y2)=4(x4+y4-x2y2) =8
=>(x4+y4-x2y2)=2=>x4+y4=2+x2y2 kết hợp với x4+y4+x2y2=4
=> 2+x2y2+x2y2=4 => x2y2=1 (x4y4 sẽ = 1 nốt ) => x4+y4=3 và x8+y8=7
Xét (x4+y4)3=x12+y12+3x4y4(x4+y4)=x12+y12+3.1.3=33=27
=>x12+y12=18=> A = 18+1=19

\(A=\dfrac{x+8+\sqrt{x}+2}{x\sqrt{x}+8}+\dfrac{2-\sqrt{x}}{x-4}\)
\(=\dfrac{x+\sqrt{x}+10}{x\sqrt{x}+8}-\dfrac{1}{\sqrt{x}+2}\)
\(=\dfrac{x+\sqrt{x}+10-x+2\sqrt{x}-4}{\left(\sqrt{x}+2\right)\left(x-2\sqrt{x}+4\right)}=\dfrac{3\sqrt{x}+6}{\left(\sqrt{x}+2\right)\left(x-2\sqrt{x}+4\right)}\)
\(=\dfrac{3}{x-2\sqrt{x}+4}\)
Để A là số nguyên thì \(x-2\sqrt{x}+4\in\left\{1;-1;3;-3\right\}\)
\(\Leftrightarrow x-2\sqrt{x}+1=0\)
=>x=1

a.\(A=\dfrac{x^2-4x+4}{x^3-2x^2-\left(4x-8\right)}=\dfrac{\left(x-2\right)^2}{x^2\left(x-2\right)-4\left(x-2\right)}=\dfrac{\left(x-2\right)^2}{\left(x^2-4\right)\left(x-2\right)}=\dfrac{x-2}{\left(x-2\right)\left(x+2\right)}=\dfrac{1}{x+2}\)
\(A=\dfrac{\left(x-2\right)^2}{x^2\left(x-2\right)-4\left(x-2\right)}\left(x\ne\pm2\right)\\ A=\dfrac{\left(x-2\right)^2}{\left(x-2\right)^2\left(x+2\right)}=\dfrac{1}{x+2}\\ B=\dfrac{x+2-x+\sqrt{x}-1}{\left(\sqrt{x}+1\right)\left(x-\sqrt{x}+1\right)}\cdot\dfrac{4\sqrt{x}}{3}\left(x>0\right)\\ B=\dfrac{4\sqrt{x}\left(\sqrt{x}+1\right)}{3\left(\sqrt{x}+1\right)\left(x-\sqrt{x}+1\right)}=\dfrac{4\sqrt{x}}{3\left(x-\sqrt{x}+1\right)}\)

a, ĐKXĐ : x khác -4;4;-2
P =[ 8+x-4/(x-4).(x+4) ] : 1/(x+2).(x-4)
= x+4/(x+4).(x-4) . (x+2).(x-4)
= x+2
b, x^2-9x+20 = 0
<=> (x^2-4x)-(5x-20)=0
<=> (x-4).(x-5)=0
<=> x-4=0 hoặc x-5=0
<=> x=4 hoặc x=5
+, Với x=4 thì P = 4+2 = 6
+, Với x=5 thì P = 5+2 = 7
k mk nha