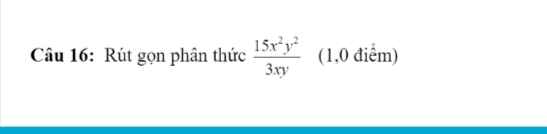Giúp với!
Mk cảm ơn trước ^^
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
PL
0
Những câu hỏi liên quan
DT
Đỗ Thanh Hải
CTVVIP
12 tháng 7 2021
1 me
2 too
3 done
doing
having
4 interested
5 as to
6 standing
when
7 helping
8 too
9 such
a
10 used
11 when
12 shouldn't
13 is - cost
CB
2
29 tháng 10 2021
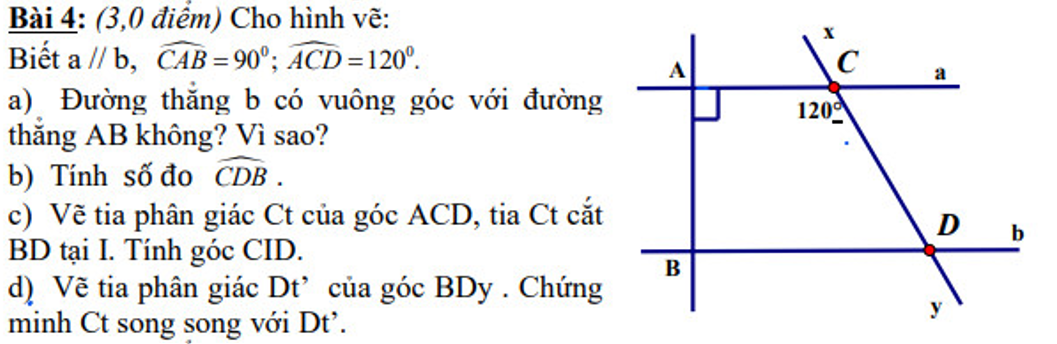
a, Vì a//b mà a⊥AB nên b⊥AB
b, Vì a//b nên \(\widehat{CDB}+\widehat{ACD}=180^0\) (trong cùng phía)
Do đó \(\widehat{CDB}=180^0-120^0=60^0\)
c, Vì Ct là p/g nên \(\widehat{ICD}=\dfrac{1}{2}\widehat{ACD}=60^0\)
Xét tg CID có \(\widehat{CID}=180^0-\widehat{ICD}-\widehat{CDB}=180^0-60^0-60^0=60^0\)
d, Vì Dt' là p/g nên \(\widehat{BDt'}=\dfrac{1}{2}\widehat{BDy}=\dfrac{1}{2}\widehat{ACD}\left(đồng.vị\right)=60^0=\widehat{CID}\)
Mà 2 góc này ở vị trí so le trong nên Ct//Dt'
DN
15 tháng 8 2023
a, Vì a//b mà a⊥AB nên b⊥AB
b, Vì a//b nên ���^+���^=1800CDB+ACD=1800 (trong cùng phía)
Do đó ���^=1800−1200=600CDB=1800−1200=600
c, Vì Ct là p/g nên ���^=12���^=600ICD=21ACD=600
Xét tg CID có ���^=1800−���^−���^=1800−600−600=600CID=1800−ICD−CDB=1800−600−600=600
d, Vì Dt' là p/g nên ���′^=12���^=12���^(đ�^ˋ��.�ị)=600=���^BDt′=21BDy=21ACD(đo^ˋng.vị)=600=CID
Mà 2 góc này ở vị trí so le trong nên Ct//Dt'
QN
1
29 tháng 6 2021
8) Ta có: \(x+\dfrac{3}{2}=-\dfrac{5}{3}\)
\(\Leftrightarrow x=-\dfrac{5}{3}-\dfrac{3}{2}=\dfrac{-10}{6}-\dfrac{9}{6}\)
hay \(x=-\dfrac{19}{6}\)
Vậy: \(x=-\dfrac{19}{6}\)
10) Ta có: \(\left|x-\dfrac{1}{2}\right|+75\%=\dfrac{9}{10}\)
\(\Leftrightarrow\left|x-\dfrac{1}{2}\right|=\dfrac{3}{20}\)
\(\Leftrightarrow\left[{}\begin{matrix}x-\dfrac{1}{2}=\dfrac{3}{20}\\x-\dfrac{1}{2}=-\dfrac{3}{20}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{13}{20}\\x=\dfrac{7}{20}\end{matrix}\right.\)
Vậy: \(S=\left\{\dfrac{13}{20};\dfrac{7}{20}\right\}\)
11) Ta có: \(x+\dfrac{2}{3}=-\dfrac{1}{2}\)
nên \(x=-\dfrac{1}{2}-\dfrac{2}{3}=\dfrac{-3}{6}-\dfrac{4}{6}\)
hay \(x=-\dfrac{7}{6}\)
Vậy: \(S=\left\{-\dfrac{7}{6}\right\}\)
28 tháng 8 2021
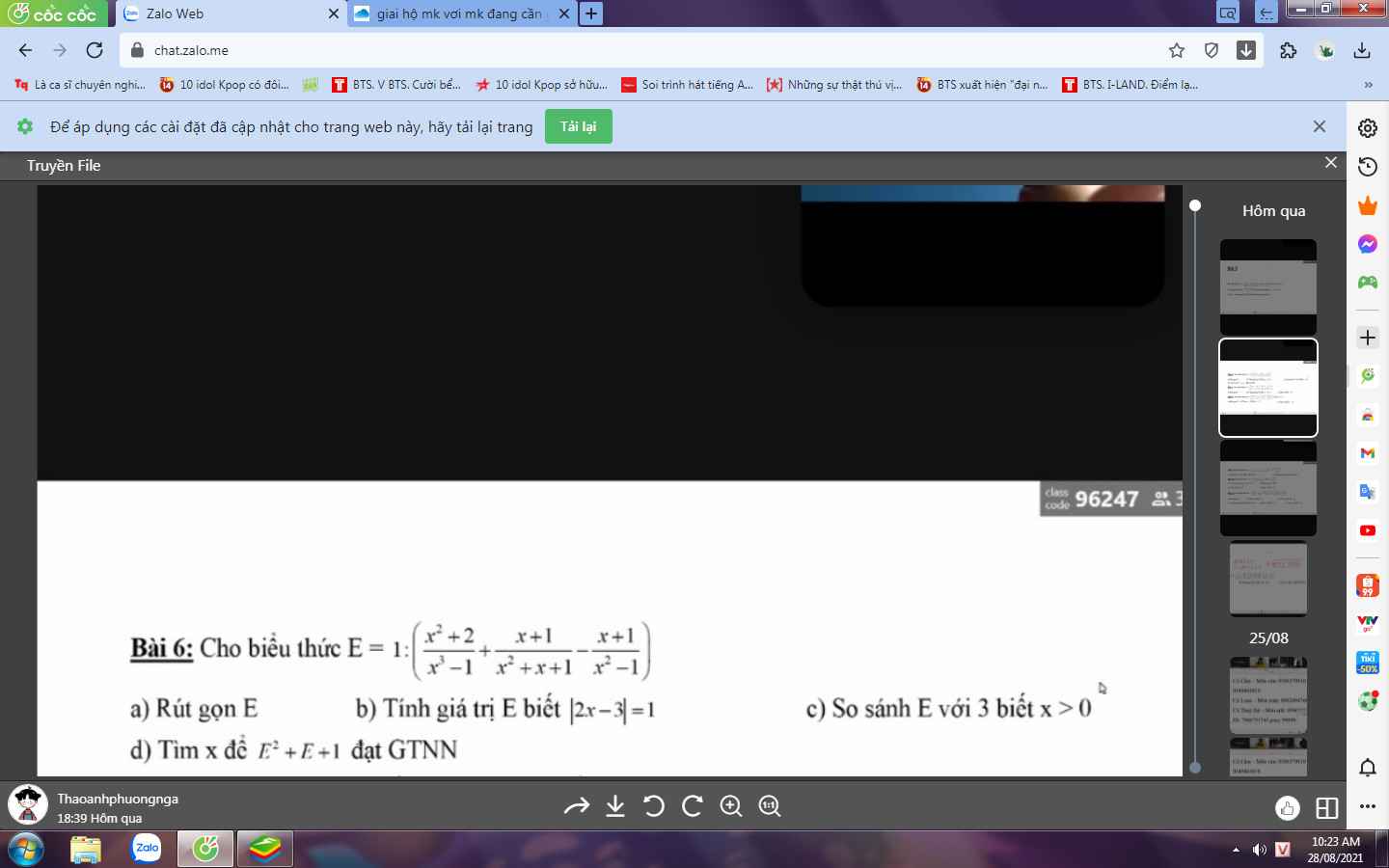
Bài 6:
a: Ta có: \(E=1:\left(\dfrac{x^2+2}{x^3-1}-\dfrac{x+1}{x^2+x+1}-\dfrac{x+1}{x^2-1}\right)\)
\(=1:\left(\dfrac{x^2+2}{\left(x-1\right)\left(x^2+x+1\right)}-\dfrac{x+1}{x^2+x+1}-\dfrac{1}{x-1}\right)\)
\(=1:\dfrac{x^2+2-x^2+1-x^2-x-1}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\dfrac{\left(x-1\right)\left(x^2+x+1\right)}{-x^2-x+2}\)
\(=\dfrac{-\left(x-1\right)\left(x^2+x+1\right)}{\left(x+2\right)\left(x-1\right)}\)
\(=\dfrac{-x^2-x-1}{x+2}\)
VT
3
NT
28 tháng 8 2021
a. ĐKXĐ: \(x\ge4\)
\(F=\left(\dfrac{2+x}{2-x}-\dfrac{4x^2}{x^2-4}-\dfrac{2-x}{2+x}\right):\dfrac{x^2-3x}{2x^2-x^3}\)
\(=\left(\dfrac{\left(2+x\right)\left(2+x\right)}{\left(2-x\right)\left(2+x\right)}+\dfrac{4x^2}{\left(2-x\right)\left(2+x\right)}-\dfrac{\left(2-x\right)\left(2-x\right)}{\left(2-x\right)\left(2+x\right)}\right):\dfrac{x\left(x-3\right)}{x^2\left(2-x\right)}\)
\(=\dfrac{4+4x+x^2+4x^2-4+4x-x^2}{\left(2-x\right)\left(2+x\right)}.\dfrac{x^2\left(2-x\right)}{x\left(x-3\right)}\)
\(=\dfrac{4x^2+8x}{\left(2-x\right)\left(2+x\right)}.\dfrac{x^2\left(2-x\right)}{x\left(x-3\right)}=\dfrac{4x\left(x+2\right)x^2\left(2-x\right)}{\left(x+2\right)\left(2-x\right)x\left(x-3\right)}=\dfrac{4x^2}{x-3}\)
b. Ta có \(\left|x-5\right|=2\) \(\Leftrightarrow\left[{}\begin{matrix}x-5=2\\5-x=2\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=7\\x=3\end{matrix}\right.\)
* Với \(x=7\), ta có biểu thức \(F=\dfrac{4.7^2}{7-3}=\dfrac{196}{4}=49\)
* Với \(x=3\), ta có biểu thức \(F=\dfrac{4.3^2}{3-3}=\dfrac{36}{0}\), lúc này biểu thức không xác định
c. \(F>0\Leftrightarrow\dfrac{4x^2}{x-3}>0\), vì \(4x^2\ge0\forall x\) nên để \(\dfrac{4x^2}{x-3}>0\) thì \(\left\{{}\begin{matrix}4x^2>0\\x-3>0\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x>0\\x>3\end{matrix}\right.\) \(\Leftrightarrow x>3\)
AH
Akai Haruma
Giáo viên
28 tháng 8 2021
\(4x^2>0\) thì không tương đương với \(x>0\) mà tương đương với \(x\ne0\)
VT
3
AH
Akai Haruma
Giáo viên
28 tháng 8 2021
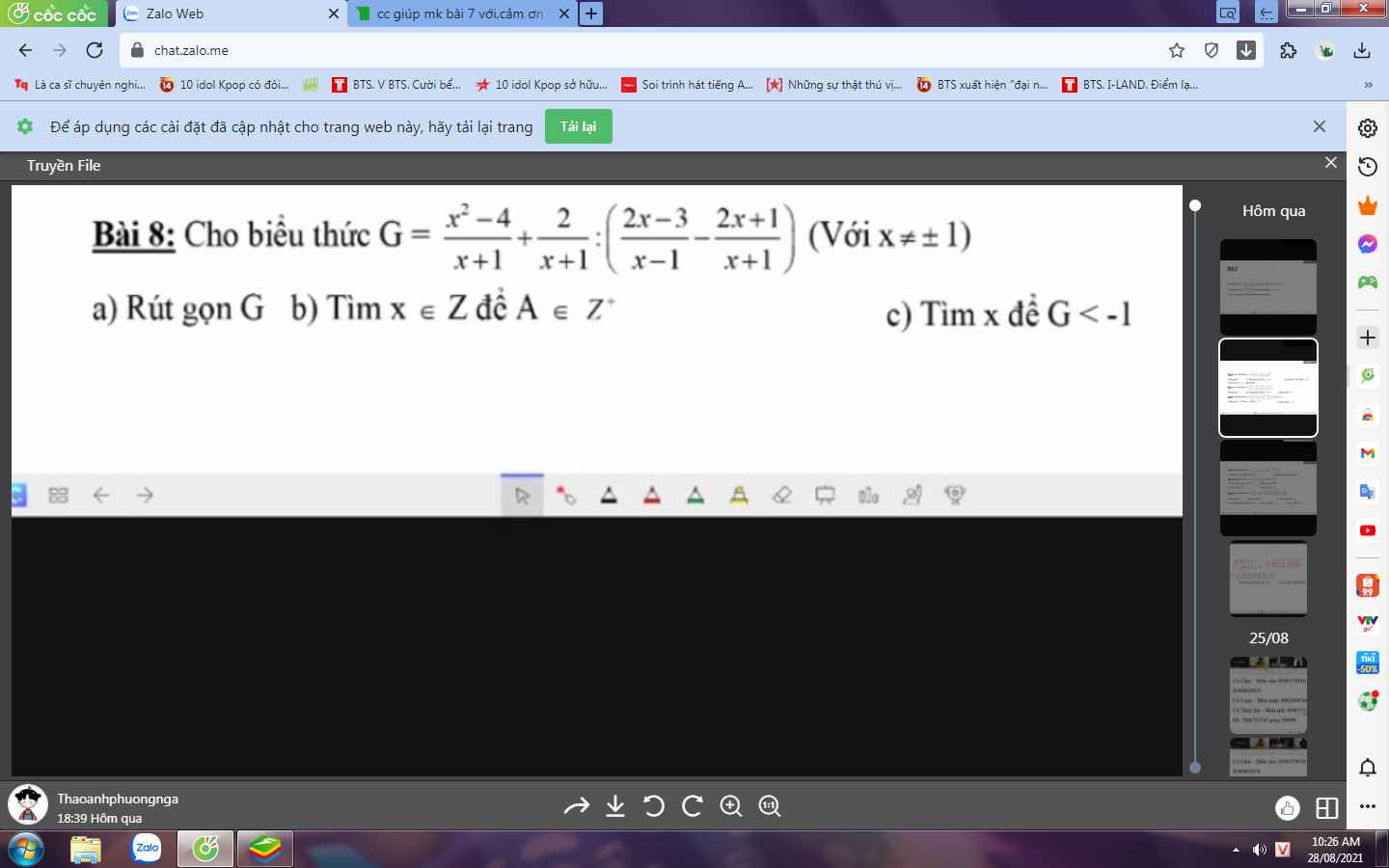
Lời giải:
a.
\(G=\frac{x^2-4}{x+1}+\frac{2}{x+1}:\frac{(2x-3)(x+1)-(2x+1)(x-1)}{(x-1)(x+1)}\)
\(=\frac{x^2-4}{x+1}+\frac{2}{x+1}:\frac{-2}{(x-1)(x+1)}=\frac{x^2-4}{x+1}+\frac{2}{x+1}.\frac{(x+1)(x-1)}{-2}\)
\(=\frac{x^2-4}{x+1}-(x-1)=\frac{x^2-4-(x^2-1)}{x+1}=\frac{-3}{x+1}\)
b.
Để $A\in\mathbb{Z}^+$ thì $x+1$ là ước âm của $-3$
$\Rightarrow x+1\in\left\{-1;-3\right\}$
$\Leftrightarrow x\in\left\{-2;-4\right\}$ (tm)
c.
$G< -1\Leftrightarrow \frac{-3}{x+1}+1< 0$
$\Leftrightarrow \frac{x-2}{x+1}< 0$
$\Leftrightarrow x-2<0< x+1$ hoặc $x-2>0>x+1$
$\Leftrightarrow -1< x< 2$ (chọn) hoặc $-1> x>2$ (loại)
Vậy $-1< x< 2$ và $x\neq 1$
28 tháng 8 2021
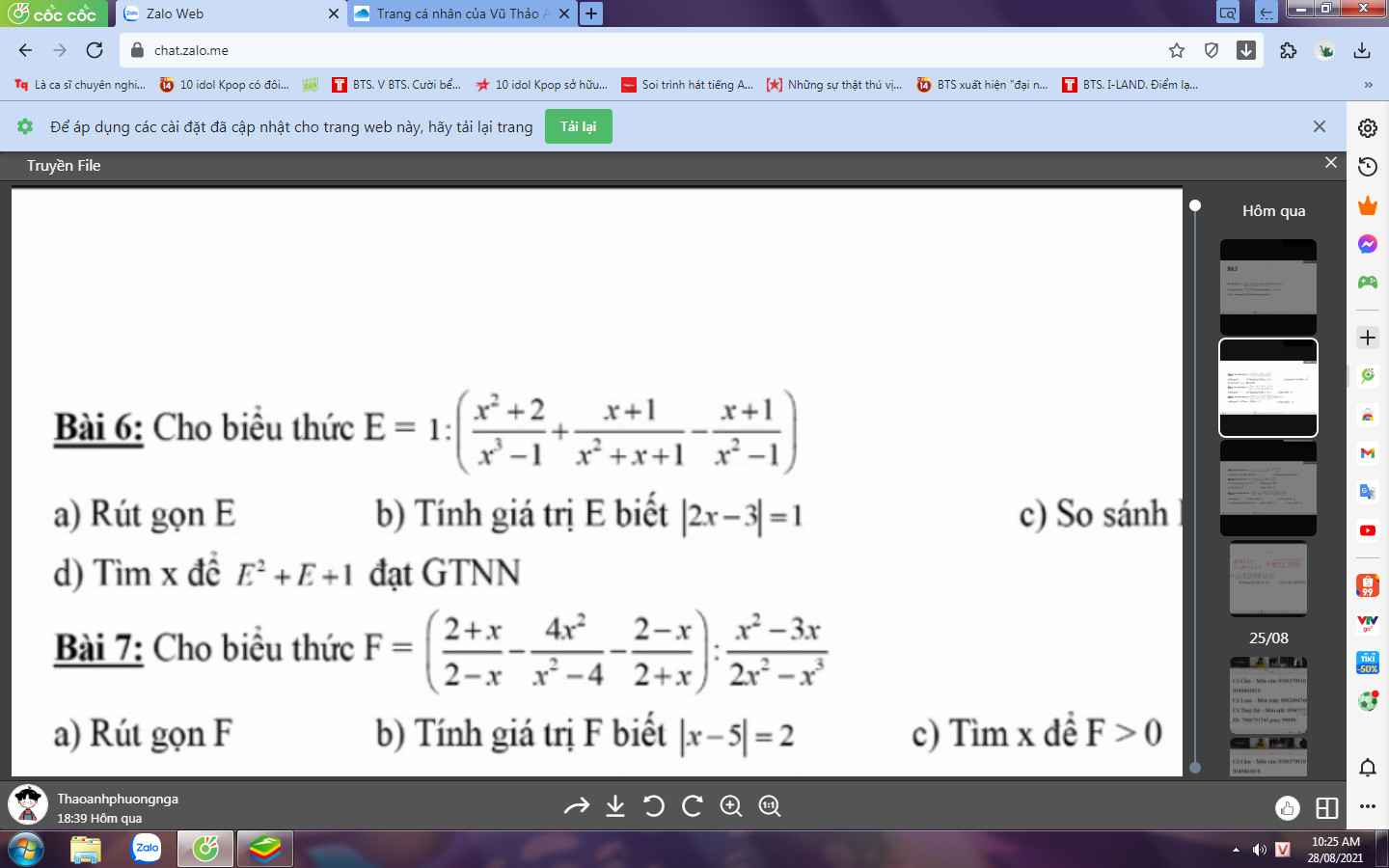
Bài 8:
a: Ta có: \(G=\dfrac{x^2-4}{x+1}+\dfrac{2}{x+1}:\left(\dfrac{2x-3}{x-1}-\dfrac{2x+1}{x+1}\right)\)
\(=\dfrac{x^2-4}{x+1}+\dfrac{2}{x+1}:\dfrac{2x^2+2x-3x-3-2x^2+2x-x+1}{\left(x-1\right)\left(x+1\right)}\)
\(=\dfrac{\left(x-2\right)\left(x+2\right)}{x+1}+\dfrac{2}{x+1}\cdot\dfrac{\left(x-1\right)\left(x+1\right)}{-2}\)
\(=\dfrac{\left(x-2\right)\left(x+2\right)}{x+1}+\dfrac{-x+1}{1}\)
\(=\dfrac{x^2-4-\left(x-1\right)\left(x+1\right)}{x+1}\)
\(=\dfrac{x^2-4-x^2+1}{x+1}\)
\(=-\dfrac{3}{x+1}\)
VT
1
28 tháng 8 2021
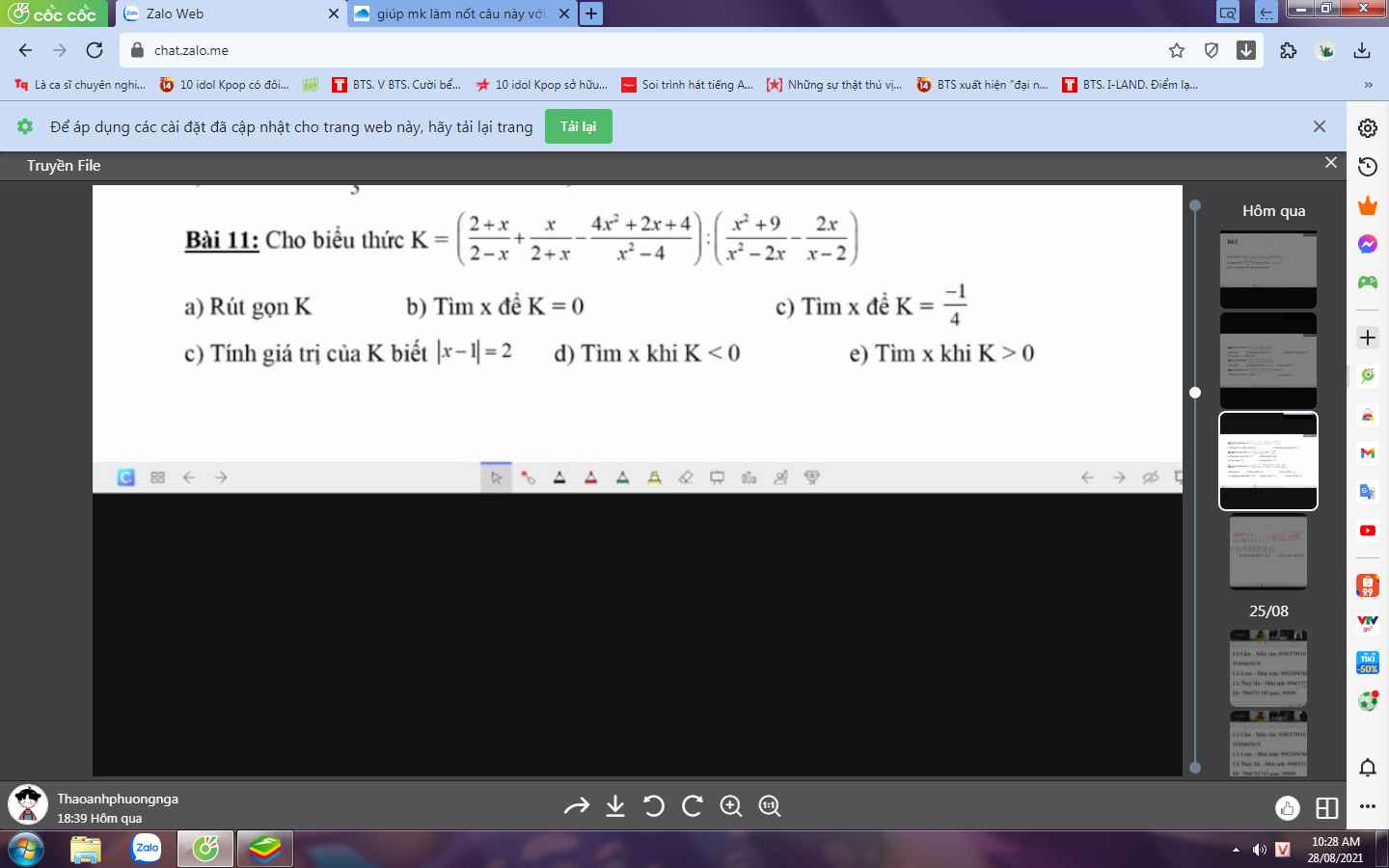
a: Ta có: \(K=\left(\dfrac{2+x}{2-x}+\dfrac{x}{2+x}-\dfrac{4x^2+2x+4}{x^2-4}\right):\left(\dfrac{x^2+9}{x^2-2x}-\dfrac{2x}{x-2}\right)\)
\(=\dfrac{-x^2-4x-4+x^2-2x-4x^2-2x-4}{\left(x-2\right)\left(x+2\right)}:\dfrac{x^2+9-2x^2}{x\left(x-2\right)}\)
\(=\dfrac{-4x^2-8x-8}{\left(x-2\right)\left(x+2\right)}\cdot\dfrac{x\left(x-2\right)}{-x^2+9}\)
\(=\dfrac{-4\left(x^2+2x+1\right)}{x+2}\cdot\dfrac{x}{-\left(x-3\right)\left(x+3\right)}\)
\(=\dfrac{-4x\left(x+1\right)^2}{-\left(x-3\right)\left(x+3\right)\left(x+2\right)}\)