Giải phương trình
x3 - 8 - ( x2 - 4x + 4 ) = 0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
DP
1
1 tháng 4 2020
\(a,x^2+4x=-3\Leftrightarrow x^2+4x+3=0\Leftrightarrow\left(x+1\right)\left(x+3\right)=0\)
\(\left[{}\begin{matrix}x=-1\\x=-3\end{matrix}\right.\)
\(b,3x^2+4x-4=0\Leftrightarrow3x^2+6x-2x-4=0\Leftrightarrow3x\left(x+2\right)-2\left(x+2\right)=0\Leftrightarrow\left(3x-2\right)\left(x+2\right)=0\)
\(\left[{}\begin{matrix}x=-2\\3x=2\end{matrix}\right.\)
\(\left[{}\begin{matrix}x=-2\\x=\frac{2}{3}\end{matrix}\right.\)
\(c,x^2+5x-6=0\Leftrightarrow\left(x-1\right)\left(x+6\right)=0\)
\(\left[{}\begin{matrix}x=1\\x=-6\end{matrix}\right.\)
\(d,x^2-6x=-9\Leftrightarrow x^2+6x+9=0\Leftrightarrow\left(x-3\right)^2=0\Leftrightarrow x-3=0\Leftrightarrow x=3\)
NT
1
NV
Nguyễn Việt Lâm
Giáo viên
10 tháng 1 2021
\(3x^3+6x^2-12x+8=0\)
\(\Leftrightarrow4x^3=x^3-6x^2+12x-8\)
\(\Leftrightarrow4x^3=\left(x-2\right)^3\)
\(\Rightarrow\sqrt[3]{4}.x=x-2\)
\(\Rightarrow x=\dfrac{2}{1-\sqrt[3]{4}}\)
V
0
24 tháng 2 2021
a) Ta có: \(f\left(x\right)=x\left(x^2+x-2\right)=x\left(x-1\right)\left(x+2\right)\)
Lập bảng xét dấu
Vậy để \(f\left(x\right)>0\) \(\Leftrightarrow x\in\left(-2;0\right)\cup\left(1;+\infty\right)\)
b) Ta có: \(\left(3x^2+7x-6\right)\left(5x+8\right)^2\le0\)
\(\Leftrightarrow3x^2+7x-6\le0\) \(\Leftrightarrow-3\le x\le\dfrac{2}{3}\)
Vậy \(x\in\left[-3;\dfrac{2}{3}\right]\)
TH
1
H9
HT.Phong (9A5)
CTVHS
24 tháng 10 2023
\(4x^2-5x-4\sqrt{x-1}-2=0\left(x\ge1\right)\)
\(\Leftrightarrow\left(4x^2-4x+1\right)-\left(x-1+4\sqrt{x-1}+4\right)=0\)
\(\Leftrightarrow\left(2x-1\right)^2-\left(\sqrt{x-1}+2\right)^2=0\)
\(\Leftrightarrow\left(2x-1-\sqrt{x-1}-2\right)\left(2x-1+\sqrt{x-1}+2\right)=0\)
\(\Leftrightarrow\left(2x-\sqrt{x-1}-3\right)\left(2x+\sqrt{x-1}+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{x-1}=2x-3\\\sqrt{x-1}=-\left(2x+1\right)\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=2\left(tm\right)\\x\in\varnothing\end{matrix}\right.\)
Vậy với x = 2 thì thỏa mãn pt
6 tháng 3 2021
1) Ta có: \(x^2-4x+4=0\)
\(\Leftrightarrow\left(x-2\right)^2=0\)
\(\Leftrightarrow x-2=0\)
hay x=2
Vậy: S={2}
LP
2
25 tháng 2 2017
c) \(x^2-6x+8=0\\ < =>x^2-2x-4x+8=0\\ < =>\left(x^2-2x\right)-\left(4x-8\right)=0\\ < =>x\left(x-2\right)-4\left(x-2\right)=0\\ < =>\left(x-2\right)\left(x-4\right)=0\\ \left\{\begin{matrix}x-2=0\\x-4=0\end{matrix}\right.=>\left\{\begin{matrix}x=2\\x=4\end{matrix}\right.\)
Vậy: tập nghiệm của pt là S= {2;4}.
25 tháng 2 2017
a) \(x^2-4x+1=0\\ < =>\left(x^2-4x+4\right)-3=0\\ < =>\left(x-2\right)^2-3=0\\ < =>\left(x-2\right)^2=3\\ =>\left(x-2\right)=\sqrt{3}hoặc\left(x-2\right)=-\sqrt{3}\)
+) x-2= \(\sqrt{3}\) => x= \(\sqrt{3}+2\)
+) x-2 = \(-\sqrt{3}\)=> x= \(-\sqrt{3}+2\)
Vậy: tập nghiệm của pt là S= { \(-\sqrt{3}+2;\sqrt{3}+2\)}
CM
6 tháng 12 2017
(x2 – 4x + 2)2 + x2 – 4x – 4 = 0
⇔ (x2 – 4x + 2)2 + x2 – 4x + 2 – 6 = 0 (1)
Đặt x2 – 4x + 2 = t,
Khi đó (1) trở thành: t2 + t – 6 = 0 (2)
Giải (2): Có a = 1; b = 1; c = -6
⇒ Δ = 12 – 4.1.(-6) = 25 > 0
⇒ (2) có hai nghiệm
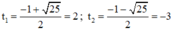
+ Với t = 2 ⇒ x2 – 4x + 2 = 2
⇔ x2 – 4x = 0
⇔ x(x – 4) = 0
⇔ x = 0 hoặc x = 4.
+ Với t = -3 ⇒ x2 – 4x + 2 = -3
⇔ x2 – 4x + 5 = 0 (*)
Có a = 1; b = -4; c = 5 ⇒ Δ’ = (-2)2 – 1.5 = -1 < 0
⇒ (*) vô nghiệm.
Vậy phương trình ban đầu có tập nghiệm S = {0; 4}.
Đây là phương trình bậc 3
\(x^3-8-\left(x^2-4x+4\right)=0\Leftrightarrow x^3-8-x^2+4x-4=0\Leftrightarrow x^3-x^2+4x-12=0\Leftrightarrow x=2\)
Vậy phương trình có 1 nghiệm là x=2
x3 - 8 - (x2 - 4x + 4) = 0
<=> x3 - x2 + 4x - 8 - 4 = 0
<=> x3 - x2 + 4x - 12 = 0
<=> (x - 2)(x2 + x + 6) = 0
<=> x - 2 = 0 hoặc x2 + x + 6 khác 0
<=> x = 2