Giải phương trình:
(x + 2)2 - (x - 2)2 = 12(x2 - x) + 8
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Lời giải:
Đặt $x^2+x=a$ thì pt trở thành:
$(a-2)(a-3)=12$
$\Leftrightarrow a^2-5a+6=12$
$\Leftrightarrow a^2-5a-6=0$
$\Leftrightarrow (a+1)(a-6)=0$
$\Leftrightarrow a+1=0$ hoặc $a-6=0$
$\Leftrightarrow x^2+x+1=0$ hoặc $x^2+x-6=0$
Nếu $x^2+x+1=0$
$\Leftrightarrow (x+\frac{1}{2})^2=-\frac{3}{4}<0$ (vô lý - loại)
Nếu $x^2+x-6=0$
$\Leftrightarrow (x-2)(x+3)=0$
$\Leftrightarrow x=2$ hoặc $x=-3$

a.\(x^2-25=8\left(5-x\right)\)
\(\Leftrightarrow\left(x-5\right)\left(x+5\right)-8\left(5-x\right)=0\)
\(\Leftrightarrow\left(x-5\right)\left(x+5\right)+8\left(x-5\right)=0\)
\(\Leftrightarrow\left(x-5\right)\left(x+13\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=5\\x=-13\end{matrix}\right.\)
b.\(\dfrac{x-2}{x+2}-\dfrac{2\left(x-11\right)}{x^2-4}=\dfrac{3}{x-2}\) ; \(ĐK:x\ne\pm2\)
\(\Leftrightarrow\dfrac{\left(x-2\right)\left(x-2\right)-2\left(x-11\right)}{\left(x-2\right)\left(x+2\right)}=\dfrac{3\left(x+2\right)}{\left(x-2\right)\left(x+2\right)}\)
\(\Leftrightarrow\left(x-2\right)^2-2\left(x-11\right)=3\left(x+2\right)\)
\(\Leftrightarrow x^2-4x+4-2x+22=3x+6\)
\(\Leftrightarrow x^2-9x+20=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=5\left(tm\right)\\x=4\left(tm\right)\end{matrix}\right.\)

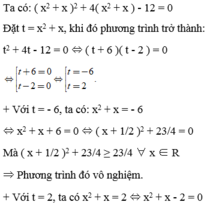
⇔ ( x + 2 )( x - 1 ) = 0 ⇔ 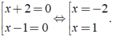
Vậy phương trình có tập nghiệm là S = { - 2;1 }.

1. Đặt $x^2+x=a$ thì pt trở thành:
$a^2+4a=12$
$\Leftrightarrow a^2+4a-12=0$
$\Leftrightarrow (a-2)(a+6)=0$
$\Leftrightarrow a-2=0$ hoặc $x+6=0$
$\Leftrightarrow x^2+x-2=0$ hoặc $x^2+x+6=0$
Dễ thấy $x^2+x+6=0$ vô nghiệm.
$\Rightarrow x^2+x-2=0$
$\Leftrightarrow (x-1)(x+2)=0$
$\Leftrightarrow x=1$ hoặc $x=-2$
2.
$x(x-1)(x+1)(x+2)=24$
$\Leftrightarrow [x(x+1)][(x-1)(x+2)]=24$
$\Leftrightarrow (x^2+x)(x^2+x-2)=24$
$\Leftrightarrow a(a-2)=24$ (đặt $x^2+x=a$)
$\Leftrightarrow a^2-2a-24=0$
$\Leftrightarrow (a+4)(a-6)=0$
$\Leftrightarrow a+4=0$ hoặc $a-6=0$
$\Leftrightarrow x^2+x+4=0$ hoặc $x^2+x-6=0$
Nếu $x^2+x+4=0$
$\Leftrightarrow (x+\frac{1}{2})^2=\frac{1}{4}-4<0$ (vô lý - loại)
Nếu $x^2+x-6=0$
$\Leftrightarrow (x-2)(x+3)=0$
$\Leftrightarrow x-2=0$ hoặc $x+3=0$
$\Leftrightarrow x=2$ hoặc $x=-3$

\(\dfrac{1}{x+2}\)+\(\dfrac{5}{x-2}\)=\(\dfrac{2x-12}{x^2-4}\)
(đkxđ: x≠2, x≠-2)
⇔ \(\dfrac{x-2}{x^2-4}\)+\(\dfrac{5\left(x+2\right)}{x^2-4}\)= \(\dfrac{2x-12}{x^2-4}\)
⇔ x-2+5(x+2)=2x-12
⇔ x-2+5x+10=2x-12
⇔ 4x=-20
⇔ x=-5(tm)


\(ĐKXĐ:x\ge2\)
Phương trình đã cho \(\Leftrightarrow x^2-5x-2\sqrt{x-2}+8=0\)
\(\Leftrightarrow\left(x^2-6x+9\right)+\left(x-2-2\sqrt{x-2}+1\right)=0\)
\(\Leftrightarrow\left(x-3\right)^2+\left(\sqrt{x-2}-1\right)^2=0\)
Dấu "=" xảy ra \(\Leftrightarrow\left\{{}\begin{matrix}\left(x-3\right)^2=0\\\left(\sqrt{x-2}-1\right)^2=0\end{matrix}\right.\) \(\Leftrightarrow x=3\) ( Thỏa mãn )
Vậy pt đã cho có nghiệm duy nhất \(x=3\)
\(\left(x+2\right)^2-\left(x-2\right)^2=12\left(x^2-x\right)+8\)
\(\Leftrightarrow\left(x+2+x-2\right)\left(x+2-x+2\right)=12x^2-12x+8\)
\(\Leftrightarrow8x=12x^2-12x+8\)
\(\Leftrightarrow0=12x^2-20x+8\)
\(\Leftrightarrow3x^2-4x+2=0\left(\text{chia 2 vế cho 4}\right)\)
\(\text{Giải một hồi bạn sẽ có PTVN}\)
\(\text{À xin lỗi mk lộn ^_^}\)
\(\Leftrightarrow3x^2-5x+2=0\)
\(\Leftrightarrow3x^2-3x-2x+2=0\)
\(\Leftrightarrow3x\left(x-1\right)-2\left(x-1\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(3x-2\right)=0\)
\(\text{Hoặc }x-1=0\Leftrightarrow x=1\)
\(\text{Hoặc }3x-2=0\Leftrightarrow x=\frac{2}{3}\)
\(\text{Vậy }x=1\text{ hoặc }x=\frac{2}{3}\)