tìm x biết \(\left(x^2-10x+15\right)\left(x^2-12x+15\right)\)=\(4x\left(x^2-6x+15\right)\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
PA
25 tháng 1 2017
2x3 + 3x2 + 6x + 5 = 02
<=> 2x3 + x2 + 5x + 2x2 + x + 5 = 0
<=> x(2x2 + x + 5) + (2x2 + x + 5) = 0
<=> (2x2 + x + 5)(x + 1) = 0
<=> x + 1 = 0 (vì 2x2 + x + 5 \(\ge\) 4,875 > 0 \(\forall\) x)
<=> x = - 1
Vậy tập nghiệm của pt là \(S=\left\{-1\right\}\)
25 tháng 1 2017
b) 4x4 + 12x3 + 5x2 - 6x - 15 = 0
<=> 4x4 + 10x3 + 2x3 + 5x2 - 6x - 15 = 0
<=> 2x3(2x + 5) + x2(2x + 5) - 3(2x + 5) = 0
<=> (2x + 5)(2x3 + x2 - 3) = 0
<=> (2x + 5)(2x3 - 2x2 + 3x2 - 3) = 0
<=> (2x + 5)(x - 1)(2x2 + 3x + 3) = 0
<=> (2x + 5)(x - 1)[x2 + (x + 3/2)2 + 3/4]= 0
Mà x2 + (x + 3/2)2 + 3/4 > 0\(\forall x\)
\(\Rightarrow\left[\begin{matrix}2x+5=0\\x-1=0\end{matrix}\right.\)\(\Leftrightarrow\left[\begin{matrix}x=-\frac{5}{2}\\x=1\end{matrix}\right.\)
Vậy ...
DV
1
12 tháng 10 2018
\(A=\left(x^2+6x+5\right)\left(x^2+10x+21\right)+15\)
\(=\left[x\left(x+1\right)+5\left(x+1\right)\right].\left[x\left(x+3\right)+7\left(x+3\right)\right]+15\)
\(=\left(x+1\right)\left(x+5\right)\left(x+3\right)\left(x+7\right)+15\)
\(=\left[\left(x+1\right)\left(x+7\right)\right].\left[\left(x+3\right)\left(x+5\right)\right]+15\)
\(=\left(x^2+8x+7\right)\left(x^2+8x+15\right)+15\)
Đặt \(x^2+8x+11=a\)
Ta có:
\(A=\left(a-4\right)\left(a+4\right)+15\)
\(=a^2-1=\left(a-1\right)\left(a+1\right)\)
\(=\left(x^2+8x+10\right)\left(x^2+8x+12\right)\)
\(=\left(x^2+8x+10\right)\left[x\left(x+2\right)+6\left(x+2\right)\right]=\left(x^2+8x+10\right)\left(x+2\right)\left(x+6\right)\)
Chúc bạn học tốt.
HL
28 tháng 1 2018
a)\(4x^4+12x^3+5x^2-6x-15=0\) ⇔(x-1)(2x+5)(2\(x^2\)+3x+3)=0\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\2x+5=0\\2x^2+3x+3=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\\x=\dfrac{-5}{2}\\x=\varnothing\end{matrix}\right.\)
Vậy phương trình đã cho có tập nghiệm S=\(\left\{1;\dfrac{-5}{2}\right\}\)
b)(x+1)(x+2)(x+4)(x+5)=40 ⇔(x+1)(x+5)(x+2)(x+4)-40=0 ⇔(\(x^2\)+6x+5)(\(x^2\)+6x+8)-40=0 Đặt \(x^2+6x+5=a \) ta có: a(a+3)-40=0⇔\(a^2\)+3a-40=0⇔\((a^2-5a)+(8a-40)=0\) ⇔a(a-5)+8(a-5)=0⇔(a-5)(a+8)=0 \(\Leftrightarrow\left[{}\begin{matrix}a-5=0\\a+8=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x^2+6x+5-5=0\\x^2+6x+5+8=0\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x^2+6x=0\\x^2+6x+13=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x\left(x+6\right)=0\\x^2+6x+9+2=0\end{matrix}\right.\) \(\circledast x\left(x+6\right)=0\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-6\end{matrix}\right.\) \(\circledast x^2+6x+9+2=0\Leftrightarrow\left(x^2+6x+9\right)+2=0\Leftrightarrow\left(x+3\right)^2+2=0\Leftrightarrow\left(x+3\right)^2=-2\left(lo\text{ại}\right)\)
Vậy phương trình đã cho có tập nghiệm S=\(\left\{0;-6\right\}\)
26 tháng 2 2019
c) (x+1)(x+2)(x+4)(x+5)=40
<=> (x+1)(x+5)(x+2)(x+4)=40
<=>(x^2+6x+5)(x^2+6x+8)=40
Đặt x^2+6x+5=y
=>y(y+3)=40
=>y^2+3y=40<=>y^2+2.\(\frac{3}{2}\)y+\(\frac{9}{4}\)=40+\(\frac{9}{4}\)<=> (y+\(\frac{3}{2}\))2=42,25<=> y+\(\frac{3}{2}\)=6,5 hoặc -6,5
Bạn tự làm tiếp nha :333
23 tháng 11 2019
a)x4 - 4x3 - 19x2 +106x - 120 = 0
=>x4 -2x3 -2x3+4x2 -23x2 +46x +60x - 120 = 0
=>x3(x-2) -2x2(x-2) -23x(x-2) +60(x-2)= 0
=>(x3- 2x2 -23x+ 60)(x-2) =0
=>(x3 - 3x2 +x2 -3x -20x+60)(x -2) = 0
=>(x2 +x -20)(x-3)(x-2) = 0
=>(x2 -4x +5x -20)(x-3)(x-2) = 0
=>(x+5)(x-4)(x-3)(x-2) =0
=>x= -5; 4; 3; 2
b)=>4x4 -4x3 +16x3 -16x2 +21x2 -21x +15x -15= 0
=>(x-1)(4x3 +16x2 +21x+15)= 0
=>...bạn tự làm phần tiếp theo nhé
c)Làm giống nguyễn thị ngọc linh
4 tháng 8 2021
B: rút gọn
a) Ta có: \(\left(x-2\right)\left(x^2+2x+4\right)-6x^2+12x\)
\(=x^3-6x^2+12x-8\)
\(=\left(x-2\right)^3\)
b) Ta có: \(\left(2x+5\right)\left(5-2x\right)+\left(x-5\right)\left(4x+5\right)\)
\(=25-4x^2+4x^2+5x-20x-25\)
=-15x
29 tháng 1 2023
\(a.\) \(ax^2-a^2x-x+a\)
\(=\left(ax^2-a^2x\right)-\left(x-a\right)\)
\(=ax\left(x-a\right)-\left(x-a\right)\)
\(=\left(ax-1\right)\left(x-a\right)\)
\(b.\) \(18x^3-12x^2+2x\)
\(=2x\left(9x^2-6x+1\right)\)
\(=2x\left(3x-1\right)^2\)
\(c.\) \(x^3-5x^2-4x+20\)
\(=\left(x^3-5x^2\right)-\left(4x-20\right)\)
\(=x^2\left(x-5\right)-4\left(x-5\right)\)
\(=\left(x^2-4\right)\left(x-5\right)\)
\(=\left(x-2\right)\left(x+2\right)\left(x-5\right)\)
\(d.\) \(\left(x+7\right)\left(x+15\right)+15\)
\(=x^2+15x+7x+105+15\)
\(=x^2+22x+120\)
\(=\left(x+10\right)\left(x+12\right)\)
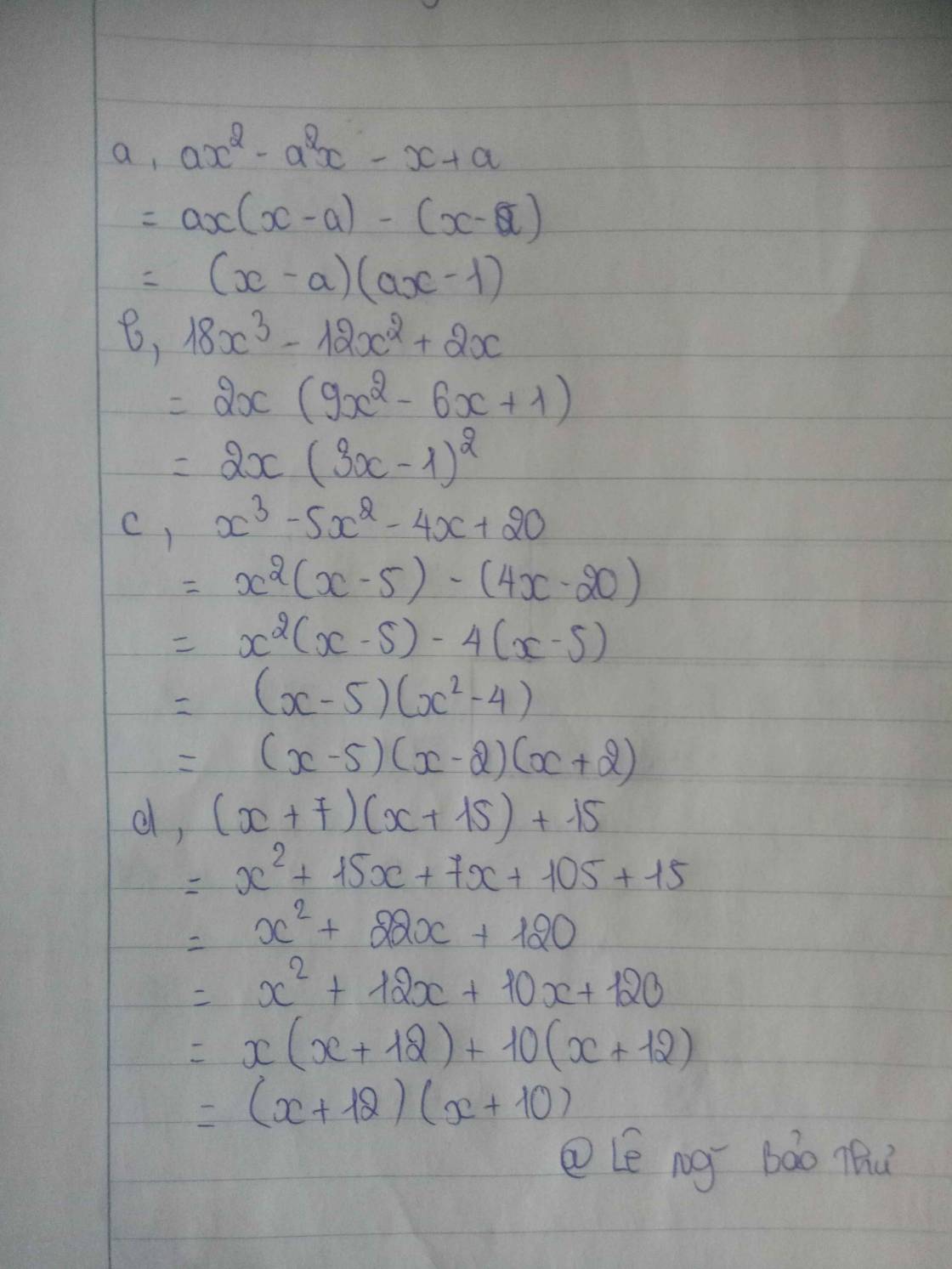
Đặt \(x^2-6x+15=a,2x=b\)
\(PT\Leftrightarrow\left(a-2b\right)\left(a-3b\right)=2ab\)
\(\Leftrightarrow a^2-7ab+6b^2=0\)
\(\Leftrightarrow\left(a-b\right)\left(a-6b\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}a=b\\a=6b\end{cases}}\)
Đến đây đơn giản rồi nhé :))))