Question

[Võ Hương Thơm]

Tìm GTNN , GTLN

\(\sqrt{x-2}\)+ \(\sqrt{4-x}\)

Answer 1

[tthnew]

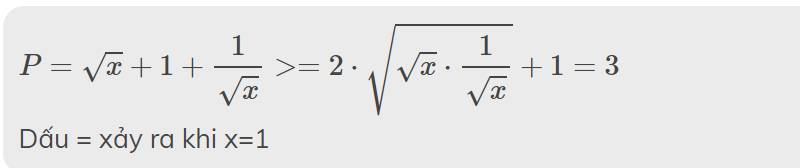
ĐK: \(2\le x\le4\)

Tìm max:

Ta có: \(\left(a-b\right)^2\ge0\Rightarrow2\left(a^2+b^2\right)\ge\left(a+b\right)^2\Rightarrow a+b\le\sqrt{2\left(a^2+b^2\right)}\)

Đẳng thức xảy ra khi a = b

Áp dụng vào \(\sqrt{x-2}+\sqrt{4-x}\le\sqrt{2\left(x-2+4-x\right)}=2\)

Tìm min: Áp dụng BĐT sau \(\sqrt{a}+\sqrt{b}\ge\sqrt{a+b}\)(tự chứng minh)

Đẳng thức xảy ra khi a = 0 hoặc b = 0

\(\sqrt{x-2}+\sqrt{4-x}\ge\sqrt{x-2+4-x}=\sqrt{2}\)

Đẳng thức xảy ra khi x = 2 hoặc x = 4

Answer 2

[svtkvtm]

\(\text{Ta co BĐT: }\sqrt{a\: }+\sqrt{b}\le\sqrt{2\left(a+b\right)}\text{ thật vậy:}\sqrt{a}+\sqrt{b}\le\sqrt{2\left(a+b\right)}\Leftrightarrow\left(\sqrt{a}+\sqrt{b}\right)^2\le2a+2b\left(vì:\sqrt{a}+\sqrt{b};\sqrt{2\left(a+b\right)}\ge0\right)\Leftrightarrow a+2\sqrt{ab}+b\le2a+2b\Leftrightarrow2\sqrt{ab}\le a+b\Leftrightarrow\left(\sqrt{a}-\sqrt{b}\right)^2\ge0\left(\text{luôn đung}\right)\Rightarrow\sqrt{x\: -2}+\sqrt{4-x\: }\le\sqrt{2\left(x\: -2+4-x\: \right)}=\sqrt{4}=2\Rightarrow A_{max\: }=2\)

\(Dâu "=" \text{ra }\Leftrightarrow x\: =3\)

\(\text{Đạt: A=}\sqrt{x\: -2}+\sqrt{4-x\: }\Rightarrow A^2=x\: -2+4-x+2\sqrt{\left(x-2\right)\left(4-x\: \right)}\: =2+2\sqrt{\left(x-2\right)\left(4-x\: \right)}\ge2+0=2\left(vì:2\sqrt{\left(x\: -2\right)\left(4-x\: \right)}\ge2.0=0\right)\Rightarrow A_{min}=\sqrt{2}\left(vì:A=\sqrt{x\: -2}+\sqrt{4-x\: }\ge0+0=0\right).\text{Dâu "=" xay ra }\)\(khi:x\: =2hoac:x\: =4\)