tập nghiệm của bất phương trình \(\left\{{}\begin{matrix}x+3< 4+2x\\5x-3< 4x-1\end{matrix}\right.\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
BT
9 tháng 5 2017
a) \(\left\{{}\begin{matrix}2x-1\le0\\-3x+5< 0\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}x\le\dfrac{1}{2}\\x>\dfrac{5}{3}\end{matrix}\right.\)\(\Leftrightarrow x\in\varnothing\).
b) Vẽ hai đường thẳng \(y=3;2x-3y+1=0\).
Vì điểm \(O\left(0;0\right)\) có tọa độ thỏa mãn bất phương trình \(2x-3y+1>0\) và không thỏa mãn bất phương trình \(3-y< 0\) nên phần không tô màu là miền nghiệm của hệ bất phương trình: \(\left\{{}\begin{matrix}3-y< 0\\2x-3y+1>0\end{matrix}\right.\).
TenAnh1
TenAnh1
A = (-4.34, -5.96)
A = (-4.34, -5.96)
A = (-4.34, -5.96)
B = (11.02, -5.96)
B = (11.02, -5.96)
B = (11.02, -5.96)
8 tháng 1 2021
1)
HPT \(\Leftrightarrow\left\{{}\begin{matrix}15x-6y=-27\\8x+6y=4\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}2y=5x+9\\23x=-23\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x=-1\\y=2\end{matrix}\right.\)
Vậy \(\left(x;y\right)=\left(-1;2\right)\)
2)
HPT \(\Leftrightarrow\left\{{}\begin{matrix}2x+y=4\\2x+4y=10\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}-3y=-6\\x=5-2y\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}y=2\\x=1\end{matrix}\right.\)
Vậy \(\left(x;y\right)=\left(1;2\right)\)
3)
HPT \(\Leftrightarrow\left\{{}\begin{matrix}4x+6y=14\\3x+6y=12\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x=2\\2y=4-x\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x=2\\y=1\end{matrix}\right.\)
Vậy \(\left(x;y\right)=\left(2;1\right)\)
4)
HPT \(\Leftrightarrow\left\{{}\begin{matrix}5x+6y=17\\54x-6y=42\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}59x=59\\y=9x-7\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=2\end{matrix}\right.\)
Vậy \(\left(x;y\right)=\left(1;2\right)\)
8 tháng 1 2021
1) \(\left\{{}\begin{matrix}3x-2y=4\\4x+2y=10\end{matrix}\right.\)
<=> \(\left\{{}\begin{matrix}3x-2y=4\\7x=14\end{matrix}\right.< =>\left\{{}\begin{matrix}x=2\\y=1\end{matrix}\right.\)
2)\(\left\{{}\begin{matrix}2x+3y=5\\4x+6y=10\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}4x+6y=10\\4x=6y=10\end{matrix}\right.\)
=> Hệ có vô số nghiệm.
3)\(\left\{{}\begin{matrix}3x-4y=-2\\10x+4y=28\end{matrix}\right.\)
<=>\(\left\{{}\begin{matrix}3x-4y=-2\\13x=26\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=2\\y=2\end{matrix}\right.\)
4)\(\left\{{}\begin{matrix}6x+15y=9\\6x-4y=28\end{matrix}\right.\)
<=>\(\left\{{}\begin{matrix}6x+15y=9\\19y=19\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=4\\y=-1\end{matrix}\right.\)
6 tháng 4 2017
a) \(x^2\ge4x\)(1)
Nếu \(\left[{}\begin{matrix}x_1=0\\x_2=4\end{matrix}\right.\) \(\Rightarrow VT=VP\)
Nếu \(x< 0\Rightarrow VT>0;VP< 0\)=> \(VT>VP\)
Nếu 0<x<4 \(\Rightarrow VT< VP\)
nếu x> 4\(\Rightarrow VT>VP\)
Kết luận nghiệm BPT (1): \(\left[{}\begin{matrix}x\le0\\x\ge4\end{matrix}\right.\)
b)
(1) \(\Rightarrow\left[{}\begin{matrix}x< \dfrac{3-\sqrt{5}}{2}\\x>\dfrac{3+\sqrt{5}}{2}\end{matrix}\right.\)
(2) \(\Rightarrow-2\le x\le3\)
KL nghiệm
\(\left[{}\begin{matrix}-2\le x< \dfrac{3-\sqrt{5}}{2}\\\dfrac{3+\sqrt{5}}{2}< x\le3\end{matrix}\right.\)
BT
9 tháng 5 2017
a)\(Bpt\Leftrightarrow\) \(\left\{{}\begin{matrix}x^2-4x\ge0\left(1\right)\\\left(2x-1\right)^2-9>0\left(2\right)\end{matrix}\right.\)
Giải (1): \(x^2-4x\ge0\Leftrightarrow\left[{}\begin{matrix}x\ge4\\x\le0\end{matrix}\right.\)
Giải (2): \(\left(2x-1\right)^2-9=\left(2x-1\right)^2-3^2=\left(2x-4\right)\left(2x+2\right)\)
\(\left(2x-4\right)\left(2x+2\right)=0\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-1\end{matrix}\right.\)
Vì vậy: \(\left(2x-1\right)^2-9< 0\Leftrightarrow-1< x< 2\).
Kết hợp điều kiện \(\left(1\right)\) và \(\left(2\right)\) suy ra: \(-1< x\le0\) thỏa mãn hệ bất phương trình.
15 tháng 4 2017
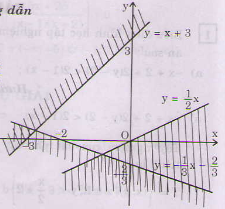
a) <=>
Miền nghiệm của hệ bất phương trình là miền không bị gạch sọc ở hình bên (không kể các điểm).
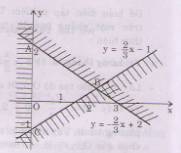
b) <=>
Miền nghiệm của hệ bất phương trình là miền tam giác ABC bao gồm cả các điểm trên cạnh AC và cạnh BC (không kể các điểm của cạnh AB).
HT
19 tháng 3 2021
a, hệ\(\Leftrightarrow\)$\left \{ {{x>\frac{1}{2} } \atop {x<m+2}} \right.$
để hệ có nghiệm ⇒ m+2< $\frac{1}{2}$ ⇒ m<$\frac{-3}{2}$