Phương trình sin3x = −sin x có bao nhiêu nghiệm thuộc đoạn [0;100π] ?
A. 300 B. 101 C. 299 D. 301
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Y
1
Những câu hỏi liên quan
NV
Nguyễn Việt Lâm
Giáo viên
5 tháng 10 2021
\(cos^4x-sin^4x=sin3x+cos4x\)
\(\Leftrightarrow\left(cos^2x+sin^2x\right)\left(cos^2x-sin^2x\right)=sin3x+cos4x\)
\(\Leftrightarrow cos2x=sin3x+cos4x\)
\(\Leftrightarrow cos4x-cos2x+sin3x=0\)
\(\Leftrightarrow-2sin3x.sinx+sin3x=0\)
\(\Leftrightarrow sin3x\left(1-2sinx\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sin3x=0\\sinx=\dfrac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{k\pi}{3}\\x=\dfrac{\pi}{6}+k2\pi\\x=\dfrac{5\pi}{6}+k2\pi\end{matrix}\right.\)
\(\Rightarrow x=\left\{0;\dfrac{\pi}{3};\dfrac{2\pi}{3};\pi;\dfrac{\pi}{6};\dfrac{5\pi}{6}\right\}\)
\(\Rightarrow\sum x=3\pi\)
NV
Nguyễn Việt Lâm
Giáo viên
25 tháng 12 2020
\(cosx-\left(3sinx-4sin^3x\right)=\sqrt{2}\left(cosx-sinx\right)sin4x\)
\(\Leftrightarrow cosx-sinx+2sinx\left(2sin^2x-1\right)=\sqrt{2}\left(cosx-sinx\right)sin4x\)
\(\Leftrightarrow cosx-sinx-2sinx\left(cosx-sinx\right)\left(cosx+sinx\right)=\sqrt{2}\left(cosx-sinx\right)sin4x\)
\(\Leftrightarrow\left(cosx-sinx\right)\left(1-2sinx\left(sinx+cosx\right)-\sqrt{2}sin4x\right)=0\)
\(\Leftrightarrow\left(cosx-sinx\right)\left(1-2sin^2x-2sinx.cosx-\sqrt{2}sin4x\right)=0\)
\(\Leftrightarrow\left(cosx-sinx\right)\left(cos2x-sin2x-\sqrt{2}sin4x=0\right)\)
\(\Leftrightarrow\left(cosx-sinx\right)\left[sin\left(\dfrac{\pi}{4}-2x\right)-sin4x\right]=0\)
\(\Leftrightarrow...\)
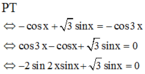
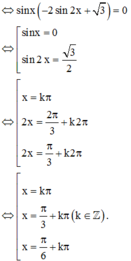
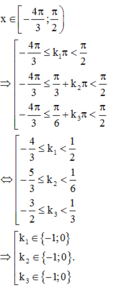
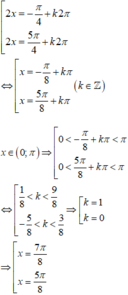
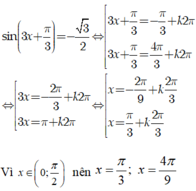
Lời giải:
\(\sin 3x=-\sin x=\sin (-x)\)
\(\Leftrightarrow \left[\begin{matrix} 3x=-x+2k\pi\\ 3x=\pi +x+2t\pi\end{matrix}\right.\) với $t,k$ nguyên bất kỳ
\(\Leftrightarrow \left[\begin{matrix} x=\frac{k\pi}{2}\\ x=\frac{(2t+1)\pi}{2}\end{matrix}\right.\) với $k,t$ nguyên bất kỳ
Để $x\in [0; 100\pi]$ thì \(\left\{\begin{matrix} 0\leq \frac{k}{2}\leq 100\\ 0\leq \frac{2t+1}{2}\leq 100\end{matrix}\right.\)
Vì $t,k$ nguyên nên:
$k\in \left\{0;1;2;...;200\right\}$ $\rightarrow 201$ giá trị
$t\in \left\{0;1;2;,,,;99\right\}$ $\rightarrow 100$ giá trị
Vậy có: $201+100=301$ nghiệm.
Em cảm mơn nhiều ạ