37 × 39 ÷ 314 + x2 =25
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
LN
2
BD
15 tháng 6 2023
\(25\cdot x^2=25\\ x^2=25\div25\\ x^2=1\\ x^2=1^2\\ x=1\\ 8^{x-3}=1\\ 8^{x-3}=8^0\\ x-3=0\\ x=0+3\\ x=3\)
15 tháng 6 2023
`@` `\text {Ans}`
`\downarrow`
\(3^{x+6}=37\)
`=>`\(3^x\cdot3^6=37\)
`=>`\(3^x=37\div3^6\)
`=>` \(3^x=\dfrac{37}{729}\)
Bạn xem lại đề.
\(25x^2=25\)
`=>`\(x^2=25\div25\)
`=>`\(x^2=1\)
`=> x=1`
\(8^{x-3}=1\)
`=>`\(8^x\div8^3=1\)
`=>`\(8^x=8^3\)
`=> x=3`
PT
1
CM
14 tháng 1 2019
37 x 25 + 37 x 35 + 37x 39 + 37
= 37x ( 25+ 35+ 39 + 1)
= 37 x 100
= 3700
KN
2
16 tháng 2 2022
Câu 9: Tính bằng cách thuận tiện nhất:
a) 876 × 64 – 876 × 54 |
=>876x(64-54)=876x10=8760
b) 37 × 25 + 37 × 35 + 37 × 39 + 37
37x(25+35+39)=37x99=3663
Chúc em học tốt
23 tháng 7 2021
a) \(\sin25^017'=\cos64^043'\)
b) \(\cos43^019'=\sin46^041'\)
c) \(\tan55^037'=\cot34^023'\)
d) \(\cot41^049'=\tan48^011'\)
AT
9 tháng 1 2017
a/ 215 + [170 -(215+170)]
= 215 +[170 - 215 - 170]
= 215 + 170 - 215 - 170
= (215 - 215) + (170 - 170) = 0
b/ (-371)- [(-371 + 29) - 229]
= (-371) - [-371 + 29 - 229]
= (-371) + 371 - 29 + 229
= (-371 + 371) + (-29 + 229)
= 0 + 200 = 200
c/ (18 + 39) + ( 158 - 39 - 18)
= 18 + 19 + 158 - 39 - 18
= (18 - 18) + (19 - 39) + 158
= 0 - 20 + 158 = 138
d/ 25 + [-37 - 25 + (-63)]
= 25 + [-37 - 25 - 63]
= 25 - 37 - 25 - 63 = (25 - 25) + (37 - 63)
= 0 - 26 = -26
TT
Bài 2:
a) 37 x 7 - 17 x 11 + 13 x 37 + 17 x 21
b) 55 x 52 : 54 - 28 : 24
c) 311 x 12 + 312 x 5 - 314
3
19 tháng 8 2023
`#040911`
`a)`
\(37 \times 7 - 17 \times 11 + 13 \times 37 + 17 \times 21\)
`= 37 \times (7 + 13) + 17 \times (21 - 11)`
`= 37 \times 20 + 17 \times 10`
`= 10 \times (37 \times 2 + 17)`
`= 10 \times 91`
`= 910`
`b)`
\(5^5 \times 5^2 \div 5^4 - 2^8 \div 2^4\)
`=`\(5^{5+2-4}-2^{8-4}\)
`= 5^3 - 2^4`
`= 125 - 16`
`= 109`
`c)`
\(3^{11}\times12+3^{12}\times5-3^{14}\)
`=`\(3^{11}\times\left(12+3\times5-3^3\right)\)
`=`\(3^{11}\times\left(12+15-27\right)\)
`=`\(3^{11}\times0=0\)
19 tháng 8 2023
a) = 259 - 187 + 481 + 357
= 910
b) = 57 : 54 - 24
= 53 - 24
= 125 - 16
= 109
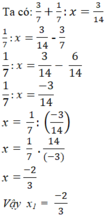
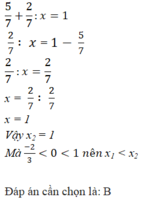
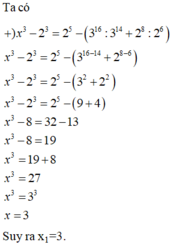
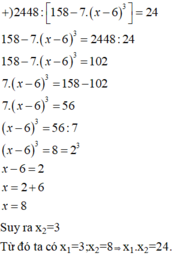
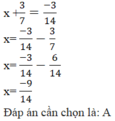
\(\frac{3^7.3^9}{3^{14}}+x^2=25\)
\(\frac{3^{16}}{3^{14}}+x^2=5^2\)
\(3^2+x^2=5^2\)
\(x^2=5^2-3^2=16=4^2=\left(-4\right)^2\)
\(=>\orbr{\begin{cases}x=4\\x=-4\end{cases}}\)
Vậy ...
\(\Leftrightarrow3^{16}\text{:}3^{14}+x^2=25.\)
\(\Leftrightarrow3^2+x^2=25\)
\(\Leftrightarrow x^2=25-3^2=16\)
\(\Rightarrow\orbr{\begin{cases}x=5\\x=-5\end{cases}}\)
Vậy \(x\in\left\{-5,5\right\}.\)