\(\dfrac{\sqrt{x^2-16}}{\sqrt{x-3}}+\sqrt{x+3}=\dfrac{7}{\sqrt{x-3}}\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(a) \sqrt{4x^2− 9} = 2\sqrt{x + 3}\)
\(ĐK:x\ge\dfrac{3}{2}\)
\(pt\Leftrightarrow4x^2-9=4\left(x+3\right)\)
\(\Leftrightarrow4x^2-9=4x+12\)
\(\Leftrightarrow4x^2-4x-21=0\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1-\sqrt{22}}{2}\left(l\right)\\x=\dfrac{1+\sqrt{22}}{2}\left(tm\right)\end{matrix}\right.\)
\(b)\sqrt{4x-20}+3.\sqrt{\dfrac{x-5}{9}}-\dfrac{1}{3}\sqrt{9x-45}=4\)
\(ĐK:x\ge5\)
\(pt\Leftrightarrow2\sqrt{x-5}+\sqrt{x-5}-\sqrt{x-5}=4\)
\(\Leftrightarrow2\sqrt{x-5}=4\Leftrightarrow\sqrt{x-5}=2\)
\(\Leftrightarrow x-5=4\Leftrightarrow x=9\left(tm\right)\)
\(c)\dfrac{2}{3}\sqrt{9x-9}-\dfrac{1}{4}\sqrt{16x-16}+27.\sqrt{\dfrac{x-1}{81}}=4\)
ĐK:x>=1
\(pt\Leftrightarrow2\sqrt{x-1}-\sqrt{x-1}+3\sqrt{x-1}=4\)
\(\Leftrightarrow4\sqrt{x-1}=4\Leftrightarrow\sqrt{x-1}=1\)
\(\Leftrightarrow x-1=1\Leftrightarrow x=2\left(tm\right)\)
\(d)5\sqrt{\dfrac{9x-27}{25}}-7\sqrt{\dfrac{4x-12}{9}}-7\sqrt{x^2-9}+18\sqrt{\dfrac{9x^2-81}{81}}=0\)
\(ĐK:x\ge3\)
\(pt\Leftrightarrow3\sqrt{x-3}-\dfrac{14}{3}\sqrt{x-3}-7\sqrt{x^2-9}+6\sqrt{x^2-9}=0\)
\(\Leftrightarrow-\dfrac{5}{3}\sqrt{x-3}-\sqrt{x^2-9}=0\Leftrightarrow\dfrac{5}{3}\sqrt{x-3}+\sqrt{x^2-9}=0\)
\(\Leftrightarrow(\dfrac{5}{3}+\sqrt{x+3})\sqrt{x-3}=0\)
\(\Leftrightarrow\sqrt{x-3}=0\) (vì \(\dfrac{5}{3}+\sqrt{x+3}>0\))
\(\Leftrightarrow x-3=0\Leftrightarrow x=3\left(nhận\right)\)

1) Đk: \(x\ge4\)
\(\dfrac{\sqrt{x^2-16}}{\sqrt{x-3}}+\sqrt{x-3}=\dfrac{7}{\sqrt{x-3}}\)
\(\Leftrightarrow\dfrac{\sqrt{x^2-16}}{\sqrt{x-3}}+\dfrac{x-3}{\sqrt{x-3}}=\dfrac{7}{\sqrt{x-3}}\)
\(\Leftrightarrow\dfrac{\sqrt{x^2-16}+x-10}{\sqrt{x-3}}=0\)
\(\Leftrightarrow\sqrt{x^2-16}+x-10=0\)
\(\Leftrightarrow\sqrt{x^2-16}=10-x\)
\(\Leftrightarrow\left\{{}\begin{matrix}x^2-16=100-20x+x^2\\x\le10\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}20x=116\\x\le10\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{29}{5}\left(N\right)\\x\le10\end{matrix}\right.\)
Kl: x= 29/5
2) Đk: \(x\ge-1\)
\(x^2-5x+14=4\sqrt{x+1}\)
\(\Leftrightarrow x^4+25x^2+196-10x^3-140x+28x^2=16x+16\)
\(\Leftrightarrow x^4-10x^3+53x^2-156x+180=0\)
\(\Leftrightarrow\left(x-3\right)\left(x^3-7x^2+32x-60\right)=0\)
\(\Leftrightarrow\left(x-3\right)^2\left(x^2-4x+20\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-3=0\\x^2-4x+20=0\left(vn\right)\end{matrix}\right.\)
\(\Leftrightarrow x=3\left(N\right)\)
Kl: x=3

1: \(\Leftrightarrow\dfrac{3x-1}{x+2}=4\)
=>4x+8=3x-1
=>x=-9
2: \(\Leftrightarrow\dfrac{5x-7}{2x-1}=4\)
=>8x-4=5x-7
=>3x=-3
=>x=-1
3: ĐKXD: x>=0
\(PT\Leftrightarrow\left(\sqrt{x}-2\right)\left(\sqrt{x}+3\right)=\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)\)
=>\(x+\sqrt{x}-6=x-1\)
=>căn x=-1+6=5
=>x=25
4: ĐKXĐ: x>=0
PT =>\(\left(\sqrt{x}-3\right)\left(\sqrt{x}+1\right)=\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)\)
=>x-2*căn x-3=x-4
=>-2căn x-3=-4
=>2căn x+3=4
=>2căn x=1
=>căn x=1/2
=>x=1/4

Ta có: \(\dfrac{\sqrt{x}\left(16-\sqrt{x}\right)}{x-4}+\dfrac{3+2\sqrt{x}}{2-\sqrt{x}}-\dfrac{2-3\sqrt{x}}{\sqrt{x}+2}\)
\(=\dfrac{16\sqrt{x}-x-\left(3+2\sqrt{x}\right)\left(\sqrt{x}+2\right)+\left(3\sqrt{x}-2\right)\left(\sqrt{x}-2\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\)
\(=\dfrac{16\sqrt{x}-x-3\sqrt{x}-6-2x-4\sqrt{x}+3x-6\sqrt{x}-2\sqrt{x}+4}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\)
\(=\dfrac{\sqrt{x}-2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}=\dfrac{1}{\sqrt{x}+2}\)

a) Ta có: \(P=\left[\left(\dfrac{1}{\sqrt{x}}+\dfrac{1}{\sqrt{y}}\right)\cdot\dfrac{2}{\sqrt{x}+\sqrt{y}}+\dfrac{1}{x}+\dfrac{1}{y}\right]:\dfrac{\sqrt{x^3}+y\sqrt{x}+x\sqrt{y}+\sqrt{y^3}}{\sqrt{x^3y}+\sqrt{xy^3}}\)
\(=\left(\dfrac{2}{\sqrt{xy}}+\dfrac{1}{x}+\dfrac{1}{y}\right):\dfrac{x\sqrt{x}+y\sqrt{x}+x\sqrt{y}+y\sqrt{y}}{x\sqrt{xy}+y\sqrt{xy}}\)
\(=\left(\dfrac{x+2\sqrt{xy}+y}{xy}\right):\dfrac{\left(x+y\right)\left(\sqrt{x}+\sqrt{y}\right)}{\sqrt{xy}\left(x+y\right)}\)
\(=\dfrac{\left(\sqrt{x}+\sqrt{y}\right)^2}{xy}\cdot\dfrac{\sqrt{xy}}{\sqrt{x}+\sqrt{y}}\)
\(=\dfrac{\sqrt{x}+\sqrt{y}}{\sqrt{xy}}\)
a) Đk:\(x>0;y>0\)
\(P=\left[\dfrac{\sqrt{x}+\sqrt{y}}{\sqrt{x}.\sqrt{y}}.\dfrac{2}{\sqrt{x}+\sqrt{y}}+\dfrac{1}{x}+\dfrac{1}{y}\right]:\dfrac{x\left(\sqrt{x}+\sqrt{y}\right)+y\left(\sqrt{x}+\sqrt{y}\right)}{x\sqrt{xy}+y\sqrt{xy}}\)
\(=\left[\dfrac{2}{\sqrt{xy}}+\dfrac{x+y}{xy}\right]:\dfrac{\left(x+y\right)\left(\sqrt{x}+\sqrt{y}\right)}{\sqrt{xy}\left(x+y\right)}\)
\(=\dfrac{2\sqrt{xy}+x+y}{xy}:\dfrac{\left(\sqrt{x}+\sqrt{y}\right)}{\sqrt{xy}}\)\(=\dfrac{\left(\sqrt{x}+\sqrt{y}\right)^2}{xy}.\dfrac{\sqrt{xy}}{\sqrt{x}+\sqrt{y}}=\dfrac{\sqrt{x}+\sqrt{y}}{\sqrt{xy}}\)
b) \(xy=16\Leftrightarrow x=\dfrac{16}{y}\)
\(P=\dfrac{\sqrt{x}+\sqrt{y}}{\sqrt{xy}}=\dfrac{1}{\sqrt{x}}+\dfrac{1}{\sqrt{y}}=\dfrac{1}{\sqrt{\dfrac{16}{y}}}+\dfrac{1}{\sqrt{y}}=\dfrac{\sqrt{y}}{4}+\dfrac{1}{\sqrt{y}}\)
Áp dụng AM-GM có:
\(\dfrac{\sqrt{y}}{4}+\dfrac{1}{\sqrt{y}}\ge2\sqrt{\dfrac{\sqrt{y}}{4}.\dfrac{1}{\sqrt{y}}}=1\)
\(\Rightarrow P\ge1\)
Dấu "=" xảy ra khi \(y=4\Rightarrow x=4\)
Vậy x=y=4 thì P đạt GTNN là 1

a) Ta có: \(\left(\dfrac{1}{2-\sqrt{3}}-\dfrac{3}{\sqrt{7}-2}\right):\dfrac{2}{\sqrt{7}+\sqrt{3}}\)
\(=\left(2+\sqrt{3}-\sqrt{7}-2\right):\dfrac{\left(\sqrt{7}-\sqrt{3}\right)}{2}\)
\(=\dfrac{-\left(\sqrt{7}-\sqrt{3}\right)}{1}\cdot\dfrac{2}{\sqrt{7}-\sqrt{3}}\)
=-2
b) Ta có: \(\left(\dfrac{x-\sqrt{x}}{1-\sqrt{x}}-1\right):\left(\sqrt{x}-x\right)+\dfrac{1}{x}\)
\(=\left(-\dfrac{\sqrt{x}\left(\sqrt{x}-1\right)}{\sqrt{x}-1}-1\right)\cdot\dfrac{-1}{\sqrt{x}\left(\sqrt{x}-1\right)}+\dfrac{1}{x}\)
\(=\left(-\sqrt{x}-1\right)\cdot\dfrac{-1}{\sqrt{x}\left(\sqrt{x}-1\right)}+\dfrac{1}{x}\)
\(=\dfrac{x+\sqrt{x}}{x\left(\sqrt{x}-1\right)}+\dfrac{\sqrt{x}-1}{x\left(\sqrt{x}-1\right)}\)
\(=\dfrac{x+2\sqrt{x}-1}{x\left(\sqrt{x}-1\right)}\)

a) ĐKXĐ: \(3\le x\le10\)
b) ĐKXĐ: \(\left\{{}\begin{matrix}x>-4\\x\ne4\end{matrix}\right.\)
c) ĐKXĐ: \(\left\{{}\begin{matrix}x\ge\dfrac{3}{2}\\x\ne4\end{matrix}\right.\)
d) ĐKXĐ: \(x\ge\dfrac{1}{2}\)
e) ĐKXĐ: \(x\in R\)
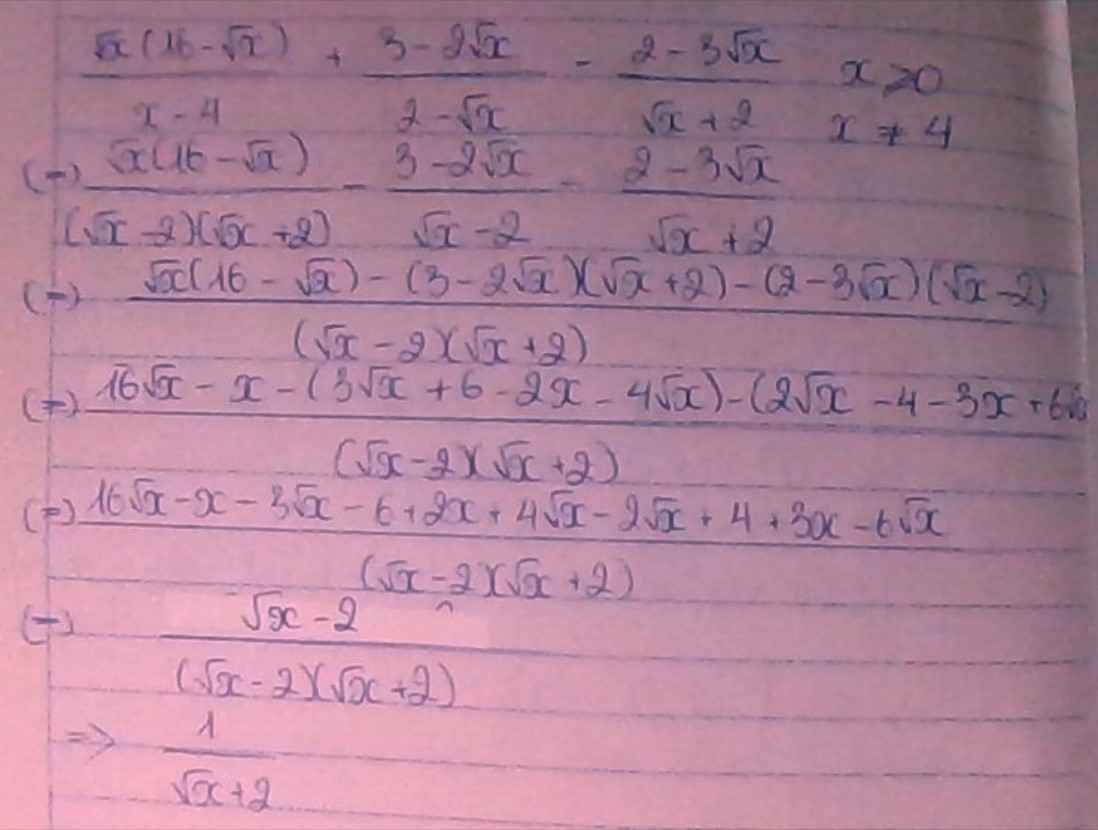
chữ xấu thông cảm ạ