 Giair hộ vs ạ
Giair hộ vs ạ
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Câu 1: She doesn't live in London because she doesn't know anyone there
->She'd.....live in London if she knew anyone there ......................................................................................................
Câu 2: I can't live in the country because I can't find a job there
->I'd...........live in the country if I could find a job there..............................................................................
Câu 3: I don't have a spare ticket. I can;t take you to the concert
->If I.........had a spare ticket, I could take you to the concert.................................
Câu 4: The schoolchildren sowed some seed, but they forgot to water them so they didn't go
-> If the children .....hadn't forgot to water the seeds, they would have grown................................
Câu 5: They don't understand a problem. They won't find a solution
-> If they...
Câu 6:He sit too much. He isn't fit
-> If he........didn't sit too much, he would be fit.....

\(\dfrac{x}{y+z+1}\) = \(\dfrac{y}{x+z+2}\) = \(\dfrac{z}{x+y-3}\) = \(x+y+z\)
Áp dụng tính chất dãy tỉ số bằng nhau ta có:
\(\dfrac{x}{y+z+1}\)=\(\dfrac{y}{x+z+2}\)=\(\dfrac{z}{x+y-3}\)=\(\dfrac{x+y+z}{y+z+1+x+z+2+x+y-3}\)
\(x+y+z\) = \(\dfrac{x+y+z}{2.\left(x+y+z\right)}\) = \(\dfrac{1}{2}\) (1)
\(\dfrac{x}{y+z+1}\) = \(\dfrac{1}{2}\) ⇒ 2\(x\) = y+z+1
⇒ 2\(x\) + \(x\) = \(x+y+z+1\) (2)
Thay (1) vào (2) ta có: 2\(x\) + \(x\) = \(\dfrac{1}{2}\) + 1
3\(x\) = \(\dfrac{3}{2}\) ⇒ \(x=\dfrac{1}{2}\)
\(\dfrac{y}{x+z+2}\) = \(\dfrac{1}{2}\) ⇒ 2y = \(x+z+2\) ⇒ 2y+y = \(x+y+z+2\) (3)
Thay (1) vào (3) ta có: 2y + y = \(\dfrac{1}{2}\) + 2
3y = \(\dfrac{5}{2}\) ⇒ y = \(\dfrac{5}{6}\)
Thay \(x=\dfrac{1}{2};y=\dfrac{5}{6}\) vào (1) ta có: \(\dfrac{1}{2}+\dfrac{5}{6}+z\) = \(\dfrac{1}{2}\)
\(\dfrac{5}{6}\) + z = 0 ⇒ z = - \(\dfrac{5}{6}\)
Kết luận: (\(x;y;z\)) = (\(\dfrac{1}{2}\); \(\dfrac{5}{6}\); - \(\dfrac{5}{6}\))

TH1: x + y + z ≠≠ 0
Áp dụng tính chất dãy tỉ số bằng nhau, ta có:
xy+z+1��+�+1 = yx+z+2��+�+2 = zx+y−3��+�−3 = x+y+zy+z+1+x+z+2+x+y−3�+�+��+�+1+�+�+2+�+�−3
= x+y+zx+y+z+x+y+z�+�+��+�+�+�+�+� = x+y+z2(x+y+z)�+�+�2(�+�+�) = 1212
⇒ x + y + z = 1212
⇒ x + y = 1212 - z
x + z = 1212 - y
y + z = 1212 - x
Thay y + z + 1 = 1212 - x + 1
⇒ x12−x+1�12−�+1 = 1212
⇒ 2x = 1212 - x + 1
⇒ 2x + x = 1212 + 1
⇒ 3x = 3232
⇒ x = 1212
Thay x + z + 2 = 1212 - y + 2
⇒ y12−y+2�12−�+2 = 1212
⇒ 2y = 1212 - y + 2
⇒ 2y + y = 1212 + 2
⇒ 3y = 5252
⇒ y = 5656
Thay x + y - 3 = 1212 - z - 3
⇒ z12−z−3=�12−�−3=\frac{1}{2}$
⇒ 2z = 1212 - z - 3
⇒ 2z + z = 1212 - 3
⇒ 3z = −52−52
⇒ z = −56−56
TH2: x + y + z = 0
⇒ xy+z+1��+�+1 = yx+z+2��+�+2 = zx+y−3��+�−3 = 0
⇒ x = y = z = 0
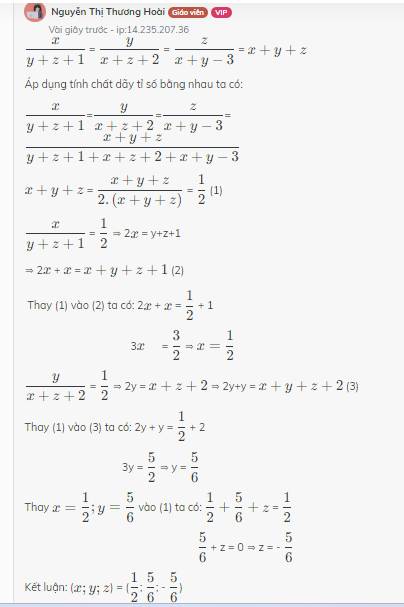
https://olm.vn/cau-hoi/tim-tat-ca-cac-so-xyz-biet-dfracxyz1dfracyxz2dfraczxy-3xyz-giair-chi-tiet-ho-e-vs-a.8297156371934

\(6,\\ a,P=9\left(x^2-2\cdot\dfrac{1}{9}x+\dfrac{1}{81}\right)+\dfrac{26}{9}=9\left(x-\dfrac{1}{9}\right)^2+\dfrac{26}{9}\ge\dfrac{26}{9}\\ P_{min}=\dfrac{26}{9}\Leftrightarrow x-\dfrac{1}{9}=0\Leftrightarrow x=\dfrac{1}{9}\\ b,Q=3\left(x^2-x+\dfrac{1}{4}\right)+\dfrac{1}{4}=3\left(x-\dfrac{1}{2}\right)^2+\dfrac{1}{4}\ge\dfrac{1}{4}\\ Q_{min}=\dfrac{1}{4}\Leftrightarrow x-\dfrac{1}{2}=0\Leftrightarrow x=\dfrac{1}{2}\\ c,R=\left(x^2-2xy+y^2\right)+x^2+1=\left(x-y\right)^2+x^2+1\ge1\\ R_{min}=1\Leftrightarrow\left\{{}\begin{matrix}x-y=0\\x=0\end{matrix}\right.\Leftrightarrow x=y=0\)



\(\Leftrightarrow\dfrac{90}{x}-\dfrac{90}{x+5}=\dfrac{1}{5}\)
\(\Leftrightarrow x\left(x+5\right)=450x+2250-450x\)
\(\Leftrightarrow x^2+5x-2250=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=45\\x=-50\end{matrix}\right.\)
ĐKXĐ: \(x\ne0;x\ne-5\)
\(\dfrac{180}{x}-\dfrac{180}{x+5}=\dfrac{2}{5}\)
\(\Leftrightarrow\dfrac{180.5.\left(x+5\right)}{5x\left(x+5\right)}-\dfrac{180.5.x}{5x\left(x+5\right)}=\dfrac{2.x.\left(x+5\right)}{5x\left(x+5\right)}\)
\(\Leftrightarrow900x+4500-900x=2x^2+10x\)
\(\Leftrightarrow2x^2+10x-4500=0\)
\(\Leftrightarrow x^2+5x-2250=0\)
\(\Leftrightarrow x^2+50x-45x-2250=0\)
\(\Leftrightarrow x\left(x+50\right)-45\left(x+50\right)=0\)
\(\Leftrightarrow\left(x+50\right)\left(x-45\right)=0\)
=> x+50=0 hoặc x-45=0
x=-50 x=45
Vậy....

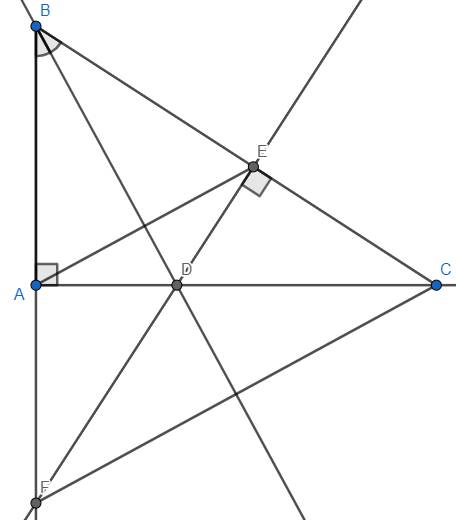
c) Ta đã biết DA = DE (chứng minh trên) (1)
Trong tam giác EDC vuông tại E có DC đối diện đỉnh E
Suy ra DC là cạnh lớn nhất trong tam giác EDC
Hay DC > DE (2)
Từ (1) và (2) suy ra DC > AD hay AD < DC

 \
\


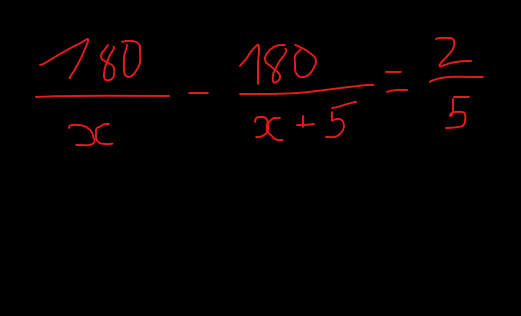

1. kitchen
2. opened
3. cook
4. good
5. sat
6. difficult
7. wasn't
8. mouth
9. ran
10. cake
1.kitchen
2.opened
3.cook
4.good
5.sat
6.difficult
7.wasn't
8.mouth
9.ran
10.cake