PTĐTTNT
\(a,x^4-4x^2+4x-1\)
\(b,4x^2-y^2+4x+1\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NT
1
16 tháng 2 2021
a) Ta có: \(8x^2+30x+7\)
\(=8x^2+28x+2x+7\)
\(=4x\left(2x+7\right)+\left(2x+7\right)\)
\(=\left(2x+7\right)\left(4x+1\right)\)
b) Ta có: \(4x^3-12x^2+9x\)
\(=x\left(4x^2-12x+9\right)\)
\(=x\left(2x-3\right)^2\)
c) Ta có: \(\left(2x+1\right)^2-\left(x-1\right)^2\)
\(=\left(2x+1-x+1\right)\left(2x+1+x-1\right)\)
\(=\left(x+2\right)\cdot3x\)
d) Ta có: \(ab+c^2-ac-bc\)
\(=\left(ab-bc\right)+\left(c^2-ac\right)\)
\(=b\left(a-c\right)+c\left(c-a\right)\)
\(=b\left(a-c\right)-c\left(a-c\right)\)
\(=\left(a-c\right)\left(b-c\right)\)
e) Ta có: \(4x^2-y^2+1-4x\)
\(=\left(4x^2-4x+1\right)-y^2\)
\(=\left(2x-1\right)^2-y^2\)
\(=\left(2x-1-y\right)\left(2x-1+y\right)\)
f) Ta có: \(6x^2-7x-20\)
\(=6x^2-15x+8x-20\)
\(=3x\left(2x-5\right)+4\left(2x-5\right)\)
\(=\left(2x-5\right)\left(3x+4\right)\)
16 tháng 2 2021
\(4x^3-12x^2+9x=x\left(4x^2-12x+9\right)=x\left(2x-3\right)^2\), \(\left(2x+1\right)^2-\left(x-1\right)^2=\left(2x+1-x+1\right)\left(2x+1+x-1\right)=\left(x+2\right)3x\)
\(ab+c^2-ac-bc=ab-ac-bc+c^2=a\left(b-c\right)-c\left(b-c\right)=\left(b-c\right)\left(a-c\right)\)
\(4x^2-y^2+1-4x=4x^2-4x+1-y^2=\left(2x-1\right)^2-y^2=\left(2x-y-1\right)\left(2x+y-1\right)\)
\(6x^2-7x-20=6x^2-15x+8x-20=3x\left(2x-5\right)+4\left(2x-5\right)=\left(2x-5\right)\left(3x+4\right)\)
\(8x^2+30x+7=8x^2+2x+28x+7=2x\left(4x+1\right)+7\left(4x+1\right)=\left(4x+1\right)\left(2x+7\right)\)
KK
24 tháng 10 2017
a, 4x2 - 4x - 3
=4x2-2x+6x-3
=2x(2x-1)+3(2x-1)
=(2x+3)(2x-1)
b, x3 - x2 - 4
= x3-x2+0x-4
= x3-2x2+x2-2x+2x-4
= (x3-2x2)+(x2-2x)+(2x-4)
= x2(x-2)+x(x-2)+2(x-2)
=(x-2)(x2+x+2)
c, 64x4+y4
=64x4+16x2y2+y4-16x2y2
= (8x2+y2)2-16x2y2
= (8x2+y2-4xy)(8x2+y2+4xy)
TL
1
26 tháng 6 2021
a,sửa đề : \(\left(\frac{1}{x^2+4x+4}-\frac{1}{x^2-4x+4}\right):\left(\frac{1}{x+2}+\frac{1}{x^2-4}\right)\)
\(=\left(\frac{1}{\left(x+2\right)^2}-\frac{1}{\left(x-2\right)^2}\right):\left(\frac{x-2+1}{\left(x+2\right)\left(x-2\right)}\right)\)
\(=\left(\frac{x^2-4x+4-x^2-4x-4}{\left(x+2\right)^2\left(x-2\right)^2}\right):\left(\frac{x-1}{\left(x+2\right)\left(x-2\right)}\right)\)
\(=\frac{-8x\left(x+2\right)\left(x-2\right)}{\left(x+2\right)^2\left(x-2\right)^2\left(x-1\right)}=\frac{-8x}{\left(x-1\right)\left(x^2-4\right)}\)
26 tháng 6 2021
b, \(\left(\frac{2x}{2x-y}-\frac{4x^2}{4x^2+4xy+y^2}\right):\left(\frac{2x}{4x^2-y^2}+\frac{1}{y-2x}\right)\)
\(=\left(\frac{2x}{2x-y}-\frac{4x^2}{\left(2x+y\right)^2}\right):\left(\frac{2x}{\left(2x-y\right)\left(2x+y\right)}-\frac{1}{2x-y}\right)\)
\(=\left(\frac{2x\left(2x+y\right)^2-4x^2\left(2x-y\right)}{\left(2x-y\right)\left(2x+y\right)^2}\right):\left(\frac{2x-\left(2x+y\right)}{\left(2x-y\right)\left(2x+y\right)}\right)\)
\(=\left(\frac{8x^3+8x^2y+2xy^2-8x^3+4x^2y}{\left(2x-y\right)\left(2x+y\right)^2}\right):\left(\frac{-y}{\left(2x-y\right)\left(2x+y\right)}\right)\)
\(=-\left(\frac{12x^2y+xy^2}{2x+y}\right)=\frac{-12x^2y-xy^2}{2x+y}\)
NV
Nguyễn Việt Lâm
Giáo viên
21 tháng 7 2021
\(y=\sqrt{\left(2x-1\right)^2}+\sqrt{\left(x-2\right)^2}=\left|2x-1\right|+\left|x-2\right|\)
\(y=\left[{}\begin{matrix}3x-3\left(\text{với }x\ge2\right)\\3-3x\left(\text{với }x\le\dfrac{1}{2}\right)\\x+1\left(\text{với }\dfrac{1}{2}\le x\le2\right)\end{matrix}\right.\)
Từ đó ta có đồ thị hàm số như sau:
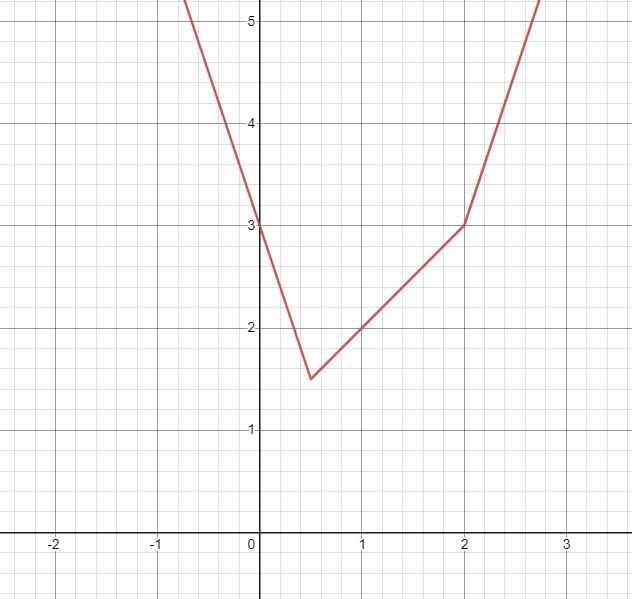
Từ đồ thị ta thấy phương trình \(\sqrt{4x^2-4x+1}+\sqrt{x^2-4x+4}=m\):
- Có đúng 1 nghiệm khi \(m=\dfrac{3}{2}\)
- Có 2 nghiệm phân biệt khi \(m>\dfrac{3}{2}\)
- Vô nghiệm khi \(m< \dfrac{3}{2}\)
8 tháng 4 2017
Ta có tổng quát: \(\left(ax^2+bx+c\right)\)\(\left(mx^2+nx+p\right)\)\(\circledast\)
-Nhân ra ta được: \(amx^4+\left(an+bm\right)x^3+\left(ap+bn+cm\right)x^2+\left(bp+cn\right)x+cp\)
-Áp dụng phương pháp hệ số bất định, ta có:
am=1
an+bm=4 (1)
ap+bn+cm=6 (2)
bp+cn=4 (3)
cp=5
-Xét a=m=1 và c=1, p=5
thay vào (1), ta được: n+b=4 (4)
thay vào (3), ta được: n+5b=4 (5)
từ (4),(5)\(\Rightarrow\)n=4 và b=0
giờ thay tất cả vào phương trình (3), ta được: 5+0+1=6 (T/M)
\(\Rightarrow\)Thay vào\(\circledast\), ta được: \(\left(x^2+1\right)\left(x^2+4x+5\right)\)
8 tháng 4 2017
Cách 2: Ta tách \(6x^2\) thành \(5x^2+x^2\)
ta được: \(x^4+4x^3+5x^2+x^2+4x+5\)
\(\Leftrightarrow x^2\left(x^2+4x+5\right)+\left(x^2+4x+5\right)\)
\(\Leftrightarrow\left(x^2+1\right)\left(x^2+4x+5\right)\)
NT
2
10 tháng 10 2016
Câu b thì bạn kia làm đúng rồi nên mình chỉ giải câu a thôi nhé
\(3x^2+4x-7=\left(3x^2-3x\right)+\left(7x-7\right)\)
\(=\left(x-1\right)\left(3x+7\right)\)
10 tháng 10 2016
a) = pp tìm nghiệm
= (x -1)( x +7/3)
b) = (2x-1)2 - 22
=(2x-1-2)(2x-1+2)
=(2x-3)(2x+1)
( ai cũng k hiu chi 1 vài ng gioi hiu)
a) x4 - 4x2 + 4x - 1
= ( x2)2 - [ ( 2x)2 - 2.2x + 1]
= ( x2)2 - ( 2x - 1)2
= ( x2 - 2x +1)( x2 + 2x - 1)
= ( x -1)2( x2 + 2x - 1)
b) 4x2 - y2 + 4x + 1
= (2x)2 + 2.2x +1 - y2
= ( 2x +1)2 - y2
= ( 2x + 1 - y)( 2x + 1 + y)
\(\text{a) }x^4-4x^2+4x-1\\ \\=x^4-\left(4x^2-4x+1\right)\\ \\ =\left(x^2\right)^2-\left(2x-1\right)^2\\ \\=\left(x^2-2x+1\right)\left(x^2+2x-1\right)\\ \\=\left(x-1\right)^2\left(x^2+2x-1\right)\)
\(\text{b) }4x^2-y^2+4x+1\\ \\=\left(4x^2+4x+1\right)-y^2\\ \\=\left(2x+1\right)^2-y^2\\ \\=\left(2x+1+y\right)\left(2x+1-y\right)\)