 ai giúp tui với
ai giúp tui với
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


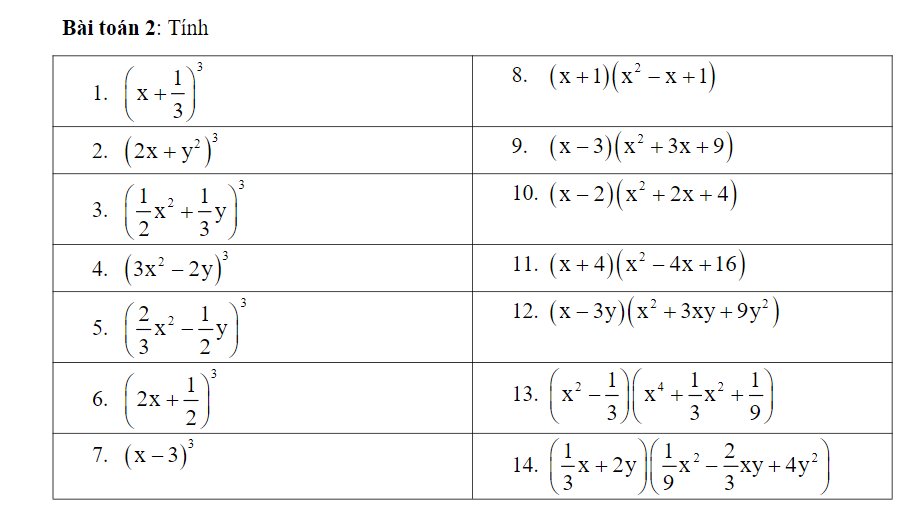
1) \(\left(x+\dfrac{1}{3}\right)^3=x^3+3.x^2.\dfrac{1}{3}+3.x.\left(\dfrac{1}{3}\right)^2+\left(\dfrac{1}{3}\right)^3\)
\(=x^3+x^2+\dfrac{x}{3}+\dfrac{1}{27}\)
2) \(\left(2x+y^2\right)^3=\left(2x\right)^3+3.\left(2x\right)^2.y^2+3.2x.\left(y^2\right)^2+\left(y^2\right)^3\)
\(=8x^3+12x^2y^2+6xy^4+y^6\)
3) \(\left(\dfrac{1}{2}x^2+\dfrac{1}{3}y\right)^3=\left(\dfrac{1}{2}x^2\right)^3+3.\left(\dfrac{1}{2}x^2\right)^2.\dfrac{1}{3}y+3.\dfrac{1}{2}x^2.\left(\dfrac{1}{3}y\right)^2+\left(\dfrac{1}{3}y\right)^3\)
\(=\dfrac{1}{8}x^6+\dfrac{1}{4}x^4y+\dfrac{1}{6}x^2y^2+\dfrac{1}{27}y^3\)
4) \(\left(3x^2-2y\right)^3=\left(3x^2\right)^3-3.\left(3x^2\right)^2.2y+3.3x^2.\left(2y\right)^2-\left(2y\right)^3\)
\(=27x^6-54x^4y+36x^2y^2-8y^3\)
5) \(\left(\dfrac{2}{3}x^2-\dfrac{1}{2}y\right)^3=\left(\dfrac{2}{3}x^2\right)^3-3.\left(\dfrac{2}{3}x^2\right)^2.\dfrac{1}{2}y+3.\dfrac{2}{3}x^2.\left(\dfrac{1}{2}y\right)^2-\left(\dfrac{1}{2}y\right)^3\)
\(=\dfrac{8}{27}x^6-\dfrac{1}{3}x^4y+\dfrac{1}{2}x^2y^2-\dfrac{1}{8}y^3\)
6) \(\left(2x+\dfrac{1}{2}\right)^3=\left(2x\right)^3+3.\left(2x\right)^2.\dfrac{1}{2}+3.2x.\left(\dfrac{1}{2}\right)^2+\left(\dfrac{1}{2}\right)^3\)
\(=8x^3+6x^2+\dfrac{3}{2}x+\dfrac{1}{8}\)
7) \(\left(x-3\right)^3=x^3-3.x^2.3+3.x.3^2-3^3\)
\(=x^3-9x^2+27x-27\)
8) \(\left(x+1\right)\left(x^2-x+1\right)\)
\(=\left(x+1\right)\left(x^2-x.1+1^2\right)\)
\(=x^3+1^3\)
\(=x+1\)
9) \(\left(x-3\right)\left(x^2+3x+9\right)\)
\(=\left(x-3\right)\left(x^2+x.3+3^2\right)\)
\(=x^3-3^3\)
\(=x^3-27\)
10) \(\left(x-2\right)\left(x^2+2x+4\right)\)
\(=\left(x-2\right)\left(x^2+x.2+2^2\right)\)
\(=x^3-2^3\)
\(=x^3-8\)
11) \(\left(x+4\right)\left(x^2-4x+16\right)\)
\(=\left(x+4\right)\left(x^2-x.4+4^2\right)\)
\(=x^3+4^3\)
\(=x^3+64\)
12) \(\left(x-3y\right)\left(x^2+3xy+9y^2\right)\)
\(=\left(x-3y\right)\left[x^2+x.3y+\left(3y\right)^2\right]\)
\(=x^3-\left(3y\right)^3\)
\(=x^3-27y^3\)
13) \(\left(x^2-\dfrac{1}{3}\right)\left(x^4+\dfrac{1}{3}x^2+\dfrac{1}{9}\right)\)
\(=\left(x^2-\dfrac{1}{3}\right)\left[\left(x^2\right)^2+x^2.\dfrac{1}{3}+\left(\dfrac{1}{3}\right)^2\right]\)
\(=\left(x^2\right)^3-\left(\dfrac{1}{3}\right)^3\)
\(=x^6-\dfrac{1}{27}\)
14) \(\left(\dfrac{1}{3}x+2y\right)\left(\dfrac{1}{9}x^2-\dfrac{2}{3}xy+4y^2\right)\)
\(=\left(\dfrac{1}{3}x+2y\right)\left[\left(\dfrac{1}{3}x\right)^2-\dfrac{1}{3}x.2y+\left(2y\right)^2\right]\)
\(=\left(\dfrac{1}{3}x\right)^3+\left(2y\right)^3\)
\(=\dfrac{1}{27}x^3+8y^3\)



His sister-in-law is a tall slim woman with long black hair and blue eyes
Playing football on the street is very dangerous for children but they always do that
The Greens get up early in the morning and do aerobics to keep fit
6 His sister - in - law is a tall slim woman with long black hair and blue eyes
7 Playing football on the street is very dangerous for chilren but they always do that
8 The Greens get up early in the morning and do aerobics to keep fit

Bạn cần hỗ trợ bài nào thì đăng bài đó lên để mọi người trợ giúp nhé.

Câu 3:
a: Xét ΔABC có AB<BC
nên \(\widehat{ACB}< \widehat{BAC}\)
b: Xét ΔABM có
AH là đường cao
AH là đường trung tuyến
Do đó: ΔABM cân tại A
mà \(\widehat{B}=60^0\)
nên ΔABM đều

Mảnh trăng non đầu tháng lơ lửng giữa trời như một cánh diều
 Tui đang cần gấp ai giúp tui với xogn tui tick
Tui đang cần gấp ai giúp tui với xogn tui tick 







dễ mà bn
bài 2
\(a,\sqrt{9x^2}=9\)
\(3x=9\)
\(x=3\left(TM\right)\)
\(b,\sqrt{x^2+3x+9}=3\)
\(x^2+3x+9=9\)
\(x^2+3x=0\)
\(x\left(x+3\right)=0\)
\(\orbr{\begin{cases}x=0\\x+3=0\end{cases}\orbr{\begin{cases}x=0\left(TM\right)\\x=-3\left(TM\right)\end{cases}}}\)
\(c,\sqrt{x^2+6x+9}+1=3x\)
\(\sqrt{\left(x+3\right)^2}+1=3x\)
\(\left|x+3\right|+1=3x\)
ta thấy \(VT=\left|x+3\right|+1>0\)
\(< =>VP=3x>0\Rightarrow x>0\)
\(\left|x+3\right|+1=3x\)
\(x+3+1=3x\)
\(x=2\left(TM\right)\)
\(d,\frac{3\sqrt{x}}{\sqrt{x}+2}=2\)
\(ĐKXĐ:x\ge0\)
\(3\sqrt{x}=2\sqrt{x}+4\)
\(x=16\left(TM\right)\)
\(e,\frac{\sqrt{x}-5}{\sqrt{x}+5}< \frac{1}{3}\)
\(ĐKXĐ:x\ge0\)
\(\frac{\sqrt{x}-5}{\sqrt{x}+5}-\frac{1}{3}< 0\)
\(\frac{\sqrt{x}-5-\sqrt{x}-5}{3\sqrt{x}+15}< 0\)
\(\frac{-10}{3\sqrt{x}+15}< 0\)luôn đúng \(\forall\)với mọi x