Cho \(y=e^{2x}\sin5x.\). Chứng minh hệ thức \(y"-4y'+29y=0\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
TN
0
TH
1
5 tháng 5 2016
ta có y'=\(e^{sinx}.\cos sx;y"=e^{sinx}.cos^2x-sinx.e^{sinx}\)
vậy y'cosx-ysinx-y"=\(e^{sinx}.cos^2x-e^{sinx}.sinx-é^{sinx}.sinx-e^{sinx}.cos^2x+e^{sinx}.sinx=0\)
2 tháng 7 2023
A chia hết cho 13
A+B=11x+29y+2x-3y=13x-26y chia hết cho 13
=>B chia hết cho 13
B chia hết cho 13
A+B chia hết cho 13
=>A chia hết cho 13
TV
1
7 tháng 1 2024
a: \(H=6x^3y^4-2x^4y^2+3x^2y^2+5x^4y^2-A\cdot x^3y^4\)
\(=x^3y^4\left(6-A\right)+x^4y^2\left(5-2\right)+3x^2y^2\)
\(=\left(6-A\right)\cdot x^3y^4+x^4y^2\cdot3+3x^2y^2\)
Để H có bậc là 6 thì 6-A=0
=>A=6
b: Khi A=6 thì \(H=\left(6-6\right)\cdot x^3y^4+3x^4y^2+3x^2y^2\)
\(=3x^4y^2+3x^2y^2\)
\(=3x^2y^2\left(x^2+1\right)\)
\(x^2+1>1>0\forall x\ne0\)
\(x^2>0\forall x\ne0\)
\(y^2>0\forall y\ne0\)
Do đó: \(x^2y^2\left(x^2+1\right)>0\forall x,y\ne0\)
=>\(H=3x^2y^2\left(x^2+1\right)>0\forall x,y\ne0\)
=>H luôn dương khi x,y khác 0
HQ
2
25 tháng 7 2021
Ta có: \(\left(x-y\right)^3+4y\left(2x^2+y^2\right)\)
\(=x^3-3x^2y+3xy^2-y^3+8x^2y+4y^3\)
\(=x^3+5x^2y+3xy^2+3y^3\)
\(=x^3+3x^2y+3xy^2+y^3+2x^2y+2y^3\)
\(=\left(x+y\right)^3+2y\left(x^2+y^2\right)\)
BT
6 tháng 12 2017
\(\left\{{}\begin{matrix}mx-y=4\\x+my=-2\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}mx=y+4\\my=-2-x\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}mxy=y^2+4y\left(y\ne0\right)\\mxy=-2x-x^2\left(x\ne0\right)\end{matrix}\right.\).
Suy ra \(y^2+4y=-2x-x^2\Leftrightarrow x^2+y^2+4y+2x=0\).
G
9 tháng 8 2023
a)\(2x^2+3x+5=0\)
\(\Leftrightarrow4x^2+6x+10=0\)
\(\Leftrightarrow\left(2x\right)^2+2.2x.\dfrac{3}{2}+\dfrac{9}{4}+\dfrac{31}{4}=0\)
\(\Leftrightarrow\left(2x+\dfrac{3}{2}\right)^2=-\dfrac{31}{4}\left(vn\right)\)
b) PT \(\Leftrightarrow\left(x^2-2x+1\right)+\left(y^2-4y+4\right)+1=0\)
\(\Leftrightarrow\left(x-1\right)^2+\left(y-2\right)^2=-1\left(vn\right)\) ( do \(VT\ge0\forall x,y\) )
c) PT \(\Leftrightarrow\left(x^2-2xy+y^2\right)+y^2+2x-6y+10=0\)
\(\Leftrightarrow\left(x-y\right)^2+2\left(x-y\right)+1+y^2-4y+4+5=0\)
\(\Leftrightarrow\left(x-y+1\right)^2+\left(y-2\right)^2=-5\left(vn\right)\)
Vậy PT vô nghiệm
9 tháng 8 2023
a: 2x^2+3x+5=0
=>x^2+3/2x+5/2=0
=>x^2+2*x*3/4+9/16+31/16=0
=>(x+3/4)^2+31/16=0(vô lý)
b: x^2-2x+y^2-4y+6=0
=>x^2-2x+1+y^2-4y+4+1=0
=>(x-1)^2+(y-2)^2+1=0(vô lý)
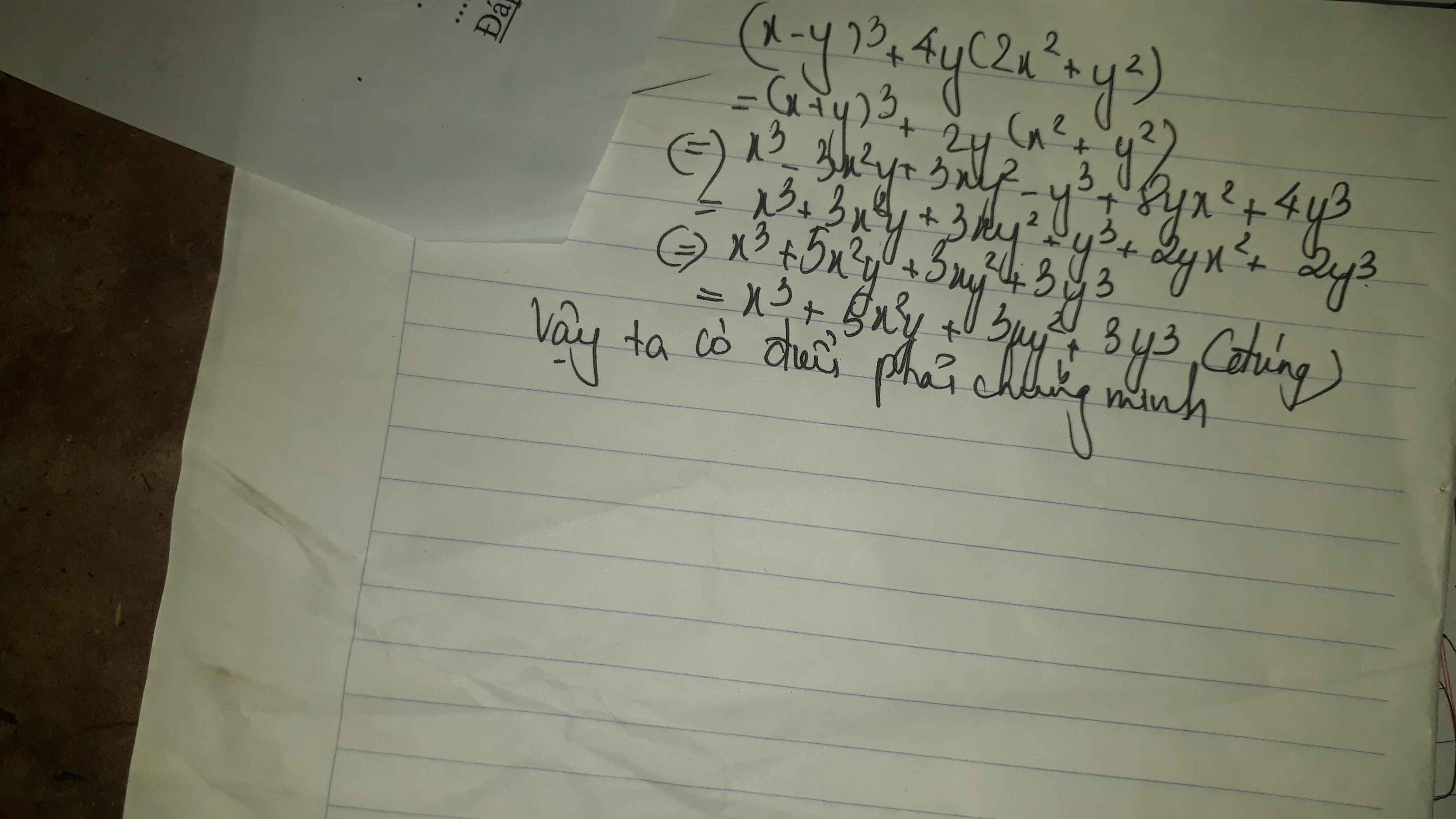
Ta có \(y'=2e^{2x}\sin5x+5e^{2x}\cos5x\)
\(y"=4e^{2x}\sin5x+10e^{2x}\cos5x+10e^{2x}\cos5x-25e^{2x}\sin5x\)
\(=-21e^{2x}\sin5x+20e^{2x}\cos5x\)
Vậy \(y"-4y'+29=-21e^{2x}\sin5x+20e^{2x}\cos5x-8e^{2x}\cos5x+29e^{2x}\sin5x=0\)