Tìm GTLNcủa biểu thức: 4x - x^2 + 3
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NH
15 tháng 10 2019
Vì x2 ≥ 0 => 2x2 ≥ 0 ; |y - 2| ≥ 0 => 3|y - 2| ≥ 0
=> (2x2 + 3|y - 2|) ≥ 0
=> (2x2 + 3|y - 2|) - 2016 ≤ 2016
Dấu " = " xảy ra <=> 2x2 = 0 và 3|y - 2| = 0
<=> x2 = 0 |y - 2| = 0
<=> x = 0 y - 2 = 0
<=> x = 0 y = 2
Vậy GTLN C = 2016 khi x = 0; y = 2
b, Ta có: \(D=\frac{x^2+15}{x^2+3}=\frac{\left(x^2+3\right)+12}{x^2+3}=1+\frac{12}{x^2+3}\)
Vì x2 ≥ 0 => x2 + 3 ≥ 3
=> \(\frac{12}{x^2+3}\le\frac{12}{3}=4\)
=> \(1+\frac{12}{x^2+3}\le1+4=5\)
Dấu " = " xảy ra <=> x2 = 0 <=> x = 0
Vậy GTNN của D = 5 khi x = 0
Đề ngược??
DD
1
17 tháng 4 2021
\(\dfrac{x^2-4x+4}{x^3-2x^2-\left(4x-8\right)}=\dfrac{\left(x-2\right)^2}{x^3-2x^2-4x+8}\)
Để biểu thức trên nhận giá trị âm khi \(\dfrac{\left(x-2\right)^2}{x^3-2x^2-4x+8}< 0\)
\(\Rightarrow x^3-2x^2-4x+8< 0\)do \(\left(x-2\right)^2\ge0\)
\(\Leftrightarrow\left(x+2\right)\left(x^2-2x+4\right)-2x\left(x+2\right)< 0\)
\(\Leftrightarrow\left(x+2\right)\left(x-2\right)^2< 0\Leftrightarrow x< -2\)
6 tháng 5 2021
>_ là lớn hơn hoặc bằng nha do bị lỗi chính tả
_< là bé hơn hoặc bằng
A,
2-5x >_ 3(2-x)
⇔ 2-5x >_ 6-3x
⇔ -5x+3x >_ 6-2
⇔ -2x >_ 3
⇔ x _< \(\dfrac{-3}{2}\)
Tập nghiệm { x / x _< \(\dfrac{-3}{2}\)}
B,
-4x + 3 _< 5x - 7
⇔ -4x - 5x _< -7 - 3
⇔ -9x _< -10
⇔ x >_ \(\dfrac{10}{9}\)
Tập nghiệm { x / x >_ \(\dfrac{10}{9}\) }
28 tháng 7 2023
1:
a: =x^2-7x+49/4-5/4
=(x-7/2)^2-5/4>=-5/4
Dấu = xảy ra khi x=7/2
b: =x^2+x+1/4-13/4
=(x+1/2)^2-13/4>=-13/4
Dấu = xảy ra khi x=-1/2
e: =x^2-x+1/4+3/4=(x-1/2)^2+3/4>=3/4
Dấu = xảy ra khi x=1/2
f: x^2-4x+7
=x^2-4x+4+3
=(x-2)^2+3>=3
Dấu = xảy ra khi x=2
2:
a: A=2x^2+4x+9
=2x^2+4x+2+7
=2(x^2+2x+1)+7
=2(x+1)^2+7>=7
Dấu = xảy ra khi x=-1
b: x^2+2x+4
=x^2+2x+1+3
=(x+1)^2+3>=3
Dấu = xảy ra khi x=-1
17 tháng 3 2021
TXĐ: \(\left\{{}\begin{matrix}x\in R\\x\notin\left\{0;-1\right\}\end{matrix}\right.\)
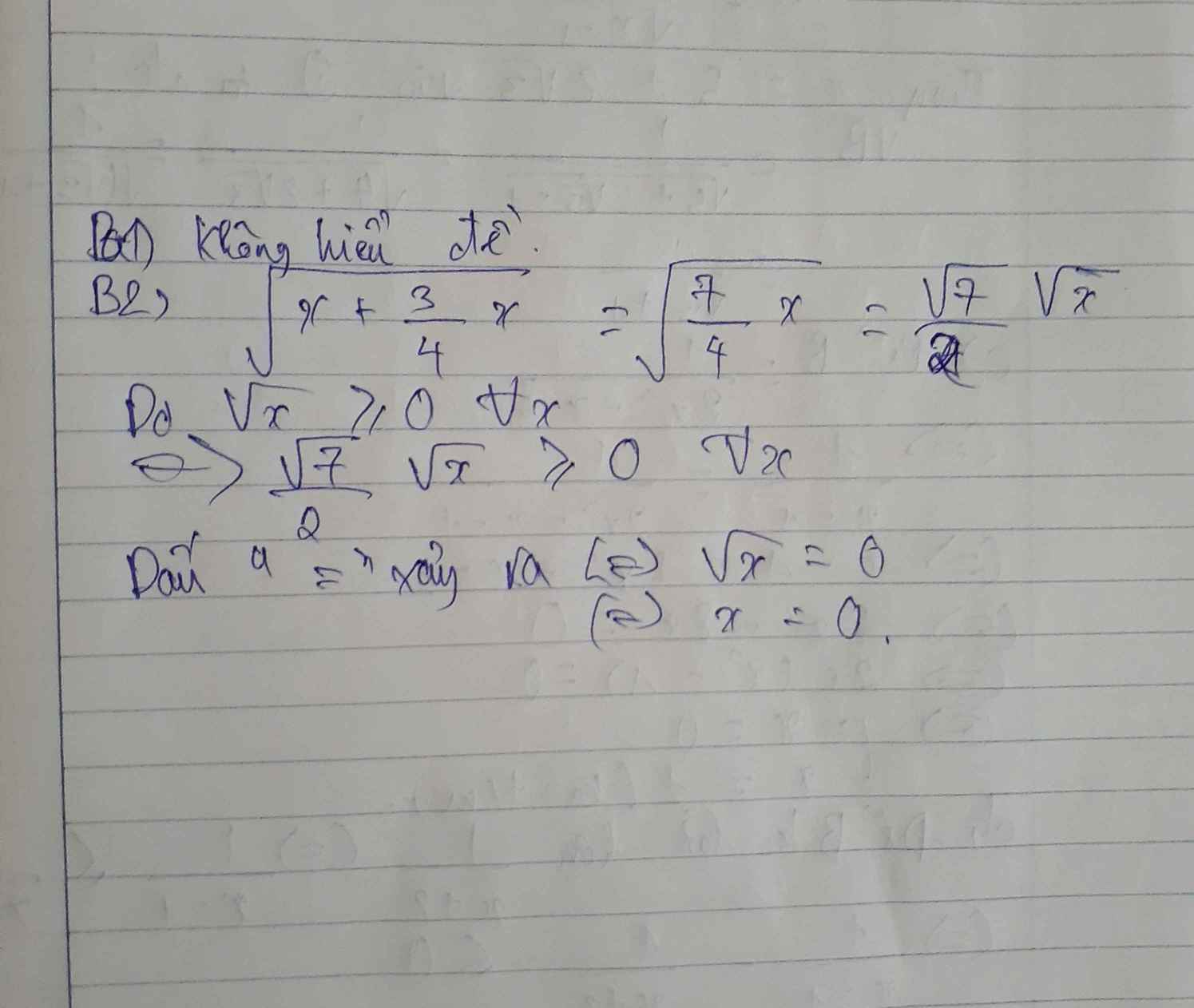
4x - x^2 đạt GTLN tại x = 2
Khi x = 2 thì 4x - x^2 = 4
=> 4x - x^2 + 3 = 4 + 3 = 7
Vậy GTLN của biểu thức trên là 7
Đặt \(A=4x-x^2+3\)
\(A=-\left(x^2-2.2x+2^2\right)+7\)
\(A=-\left(x-2\right)^2+7\)
Ta có: \(-\left(x-2\right)^2\ge0\forall x\)
\(\Rightarrow-\left(x-2\right)^2+7\le7\forall x\)
\(A=7\Leftrightarrow-\left(x-2\right)^2=0\Leftrightarrow x-2=0\Leftrightarrow x=2\)
Vậy \(A_{min}=7\Leftrightarrow x=2\)