- Tìm x, biết
.
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


1.
| x + 2 | = | 2 - 3x |
xét 2 trường hợp :
+) TH1 :
2 - 3x = x + 2
-3x + x = 2 + 2
2x = 4
x = 4 : 2 = 2
+) TH2 :
2 - 3x = - ( x + 2 )
2 - 3x = -x - 2
-3x - x = 2 - 2
-4x = 0
x = 0 : ( -4 )
x = 0
bài còn lại tương tự


a) Gọi 3 số tự nhiên lẻ liên tiếp theo thứ tự tăng dần lần lượt là: a,a+2,a+4
Theo đề bài ta có: \(\left(a+2\right)\left(a+4\right)-a\left(a+2\right)=132\)
\(\Leftrightarrow a^2+6a+8-a^2-2a=132\)
\(\Leftrightarrow4a=124\Leftrightarrow a=31\)
Vậy 3 số tự nhiên liên tiếp đó lần lượt là: 31,33,35
b) \(x-3\sqrt{x}+2=0\left(đk:x\ge0\right)\)
\(\Leftrightarrow\left(\sqrt{x}-1\right)\left(\sqrt{x}-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{x}=1\\\sqrt{x}=2\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x=1\left(tm\right)\\x=4\left(tm\right)\end{matrix}\right.\)

Vì vế trái |x(x − 4)| ≥ 0 với mọi x nên vế phải x ≥ 0.
Ta có: x|x − 4| = x (vì x ≥ 0).
Nếu x = 0 thì 0|0 − 4| = 0 (đúng)
Nếu x ≠ 0 từ x. | x - 4| = x suy ra:
|x − 4| = 1 (chia cả hai vế cho)
⇔ x – 4 = 1 hoặc x – 4 = -1
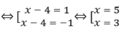
Vậy x = 0, x = 5, x = 3.

Sửa đề : a) Tìm GTNN A
a) \(A=\left|x-5\right|+3\)có : \(\left|x-5\right|\ge0\Rightarrow\left|x-5\right|+3\ge0\)
\(\Leftrightarrow A\ge3\)dấu "=" xảy ra khi : \(\left|x-5\right|=0\Leftrightarrow x-5=0\Leftrightarrow x=5\)
Vậy GTNN A = 3 khi x = 5.
b) \(C=-\left|x+1\right|+5\)có : \(-\left|x+1\right|\le0\Rightarrow-\left|x+1\right|+5\le5\)
\(\Leftrightarrow C\le5\)dấu "=" xảy ra khi : \(-\left|x+1\right|=0\Leftrightarrow x+1=0\Leftrightarrow x=-1\)
Vậy GTLN C = 5 khi x = -1.
\(D=5-\left|2x+3\right|\)có : \(-\left|2x+3\right|\le0\Rightarrow5-\left|2x+3\right|\le5\)
\(\Leftrightarrow D\le5\)dấu "=" xảy ra khi : \(-\left|2x+3\right|=0\Leftrightarrow2x+3=0\Leftrightarrow x=-\frac{3}{2}\)
Vậy GTLN D = 5 khi x = -3/2.
c) \(\left|x-3\right|+\left|y+1\right|=0\)có \(\left|x-3\right|\ge0;\left|y+1\right|\ge0\Rightarrow\left|x-3\right|+\left|y+1\right|\ge0\)
\(\Rightarrow\hept{\begin{cases}\left|x-3\right|=0\\\left|y+1\right|=0\end{cases}}\Leftrightarrow\hept{\begin{cases}x=3\\y=-1\end{cases}}.\)

Ta có: \(\dfrac{2x-4.36}{0.125}=0.25\cdot42.9-11.7\cdot0.25+0.25\cdot0.8\)
\(\Leftrightarrow\dfrac{2x-4.36}{\dfrac{1}{8}}=0.25\left(42.9-11.7+0.8\right)\)
\(\Leftrightarrow2x-4.36=\dfrac{1}{4}\cdot32\cdot\dfrac{1}{8}=1\)
\(\Leftrightarrow2x=5.36\)
hay x=2,68
\(0,25\times42,9-11,7\times0,25+0,25\times0,8=0,25\times\left(42,9-11,7+0,8\right)\)
\(=0,25\times32=8\)
Phương trình ban đầu tương đương với:
\(\frac{2\times x-4,36}{0,125}=8\)
\(\Leftrightarrow2\times x-4,36=8\times0,125\)
\(\Leftrightarrow2\times x-4,36=1\)
\(\Leftrightarrow2\times x=1+4,36\)
\(\Leftrightarrow2\times x=5,36\)
\(\Leftrightarrow x=5,36\div2=2,68\)