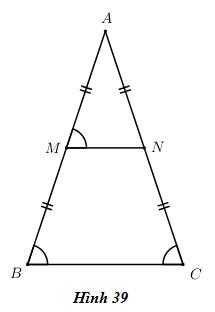
Cho tam giác ABC cân tại A (h.39). Gọi M, N lần lượt là trung điểm của AB, AC. Chứng minh rằng tứ giác MNCB là hình thang cân.
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(\dfrac{3x^2+9x-3}{x^2+x-2}-\dfrac{x+1}{x+2}+\dfrac{x-2}{1-x}\left(ĐK:x\ne\left\{1;-2\right\}\right)\\ =\dfrac{3x^2+9x-3}{\left(x+2\right)\left(x-1\right)}-\dfrac{x+1}{x+2}-\dfrac{x-2}{x-1}\\ =\dfrac{3x^2+9x-3-\left(x+1\right)\left(x-1\right)-\left(x-2\right)\left(x+2\right)}{\left(x+2\right)\left(x-1\right)}\\ =\dfrac{3x^2+9x-3-\left(x^2-1\right)-\left(x^2-4\right)}{\left(x+2\right)\left(x-1\right)}\)
\(=\dfrac{3x^2+9x-3-x^2+1-x^2+4}{\left(x+2\right)\left(x-1\right)}\\ =\dfrac{x^2+9x+2}{\left(x+2\right)\left(x-1\right)}\)

(a):
\(P=\dfrac{3x-12}{3x^2-3x-36}=\dfrac{3\left(x-4\right)}{3\left(x^2-x-12\right)}\\ =\dfrac{3\left(x-4\right)}{3\left(x-4\right)\left(x+3\right)}\\ =\dfrac{1}{x+3}\left(ĐK:x\ne\left\{4;-3\right\}\right)\)
(b):
\(x=\dfrac{1}{2}\left(TMDK\right)=>P=1:\left(\dfrac{1}{2}+3\right)=1:\dfrac{7}{2}=\dfrac{2}{7}\)
(c):
\(P=\dfrac{1}{x+3}\in Z=>1⋮\left(x+3\right)\\ =>x+3\inƯ\left(1\right)=\left\{\pm1\right\}\\ =>x\in\left\{-4;-2\right\}\left(TMDK\right)\)

\(P=\left(x+2\right)\left(2x^2-3x+4\right)-\left(x^2-1\right)\left(2x+1\right)\\ =2x^3-3x^2+4x+4x^2-6x+8-\left(2x^3+x^2-2x-1\right)\\ =2x^3+x^2-2x+8-2x^3-x^2+2x+1\\ =9\)

A = (1-3m)(9m2 +3m +1) - (6-26m3)
A = 9m2 + 3m + 1 - 27m3 - 9m2 - 3m - 6 + 26m3
A = -m3 - 5
với m = 5 ⇔A = -53 - 5 = -130
b, B = ( 2x - 3)2 + ( 2x + 1)2 - 2 (4x2 - 9)
B = ( 2x - 3 - 2x - 1)2 + 2(2x-3)(2x+1) - 2(4x2 - 9)
B = 42 + 8x2 + 4x - 12x - 6 - 8x2 + 18
B =- 8x + 16 + 18 - 6
B = -8x + 28
với x = 3 ⇔ B = -8. 3 + 28 = 4

A B C D I
a/
\(\widehat{B}+\widehat{C}=200^o\) (1)
\(\widehat{B}+\widehat{D}=180^o\) (2)
\(\widehat{C}+\widehat{D}=120^o\) (3)
Cộng 2 vế của (1) (2) (3)
\(\Rightarrow2\left(\widehat{B}+\widehat{C}+\widehat{D}\right)=500^o\Rightarrow\widehat{B}+\widehat{C}+\widehat{D}=250^o\)
\(\Rightarrow\widehat{A}=360^o-250^o=110^o\) (tổng góc trong 1 tứ giác bằng \(360^o\) )
Cộng 2 vế của (1) với (2)
\(\Rightarrow\widehat{B}+\widehat{B}+\widehat{C}+\widehat{D}=380^o\Rightarrow\widehat{B}=380^o-250^o=130^o\)
Cộng 2 vế của (2) với (3)
\(\Rightarrow\widehat{B}+\widehat{C}+\widehat{D}+\widehat{D}=300^o\Rightarrow\widehat{D}=300^o-250^o=50^o\)
\(\Rightarrow\widehat{C}=360^o-\widehat{A}-\widehat{B}-\widehat{D}=360^o-110^o-130^o-50^o=70^o\)
b/
Xét tg AIB có
\(\widehat{AIB}=180^o-\widehat{IAB}-\widehat{IBA}=180^o-\dfrac{\widehat{A}}{2}-\dfrac{\widehat{B}}{2}=\)
\(=180^o-\dfrac{110^o}{2}-\dfrac{130^o}{2}=60^o\)
\(\dfrac{\widehat{C}+\widehat{D}}{2}=\dfrac{70^o+50^o}{2}=60^o\)
\(\Rightarrow\widehat{AIB}=\dfrac{\widehat{C}+\widehat{D}}{2}=60^o\)

Các điểm em cần phải viết hoa nhé. Cô đã sửa lại, em lưu ý lần sau nếu ko thì câu hỏi sẽ bị xóa