Tìm GTNN của biểu thức:
a) C= x+60/√x + 2 ( x≥0, x khác 4)
b) B = x+8/√x + 1 ( x≥0)
c) D = x+27/√x + 3 ( x≥0)
Giúp mình với ạ! Mình cần gấp
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a, Thay x = vào A ta được : \(A=\frac{3}{3-2}=3\)
b, Với \(x\ge0;x\ne4\)
\(B=\frac{3}{\sqrt{x}+2}+\frac{\sqrt{x}}{\sqrt{x}-2}-\frac{\sqrt{x}-10}{x-4}\)
\(=\frac{3\sqrt{x}-6+x+2\sqrt{x}-\sqrt{x}+10}{x-4}=\frac{4\sqrt{x}+4+x}{x-4}\)
\(=\frac{\left(\sqrt{x}+2\right)^2}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}=\frac{\sqrt{x}+2}{\sqrt{x}-2}\)(đpcm)

Với \(x\ge0;x\ne\pm16\)
\(B=\left(\frac{\sqrt{x}}{\sqrt{x}+4}+\frac{4}{\sqrt{x}-4}\right):\frac{x+16}{\sqrt{x}+2}\)
\(=\left(\frac{x-4\sqrt{x}+4\sqrt{x}+16}{x-16}\right):\frac{x+16}{\sqrt{x}-2}=\frac{\sqrt{x}-2}{x-16}\)


này thì Cauchy cái gì bạn :v
Với x ≥ 0 thì √x + 5 ≥ 5 => 10/(√x + 5) ≤ 2 => -10/(√x + 5) ≥ -2
Dấu "=" xảy ra <=> x = 0 . Vậy MinA = -2

ĐK : x >= 0
Vì \(\sqrt{x}+5\ge5\)
\(\Rightarrow A=-\frac{10}{\sqrt{x}+5}\ge-\frac{10}{5}=-2\)
Dấu ''='' xảy ra khi x = 0
Vậy GTNN của A bằng - 2 tại x = 0


f, \(ĐK:x\ge0;x\ne1\)
\(F=\left(\frac{x+2}{x\sqrt{x}-1}+\frac{\sqrt{x}}{x+\sqrt{x}+1}+\frac{1}{1-\sqrt{x}}\right):\frac{\sqrt{x}-1}{2}\)
\(F=\left(\frac{x+2}{\sqrt{x}^3-1}+\frac{\sqrt{x}\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}-\frac{x+\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\right)\cdot\frac{2}{\sqrt{x}-1}\)
\(F=\left(\frac{x+2+x-\sqrt{x}-x-\sqrt{x}-1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\right)\cdot\frac{2}{\sqrt{x}-1}\)
\(F=\frac{x-2\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\cdot\frac{2}{\sqrt{x}-1}\)
\(F=\frac{\left(\sqrt{x}-1\right)^2}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\cdot\frac{2}{\sqrt{x}-1}=\frac{2}{x+\sqrt{x}+1}\)


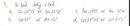
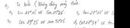


Viết đề bài khó hiểu quá!
lớp 9 mà lị