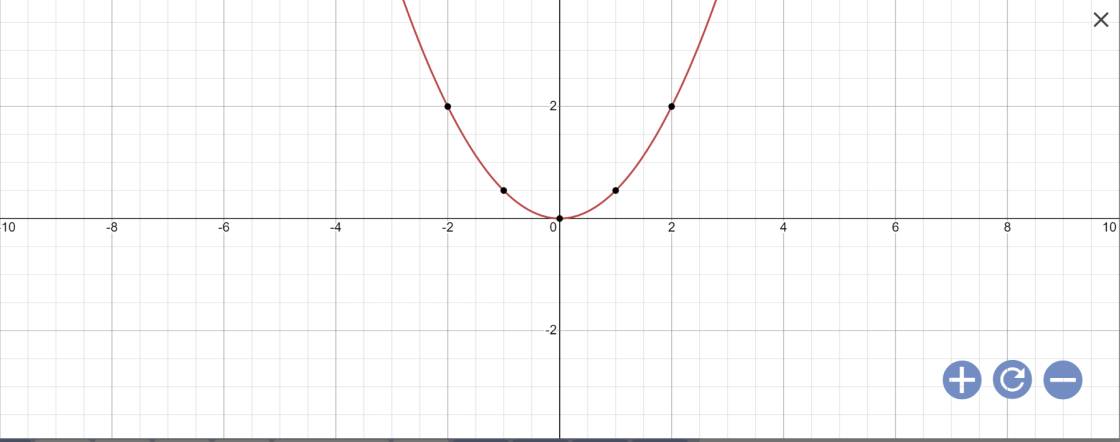
Bạn hãy điền số thích hợp vào chỗ trống.
Hùng và Quân chơi rất thân với nhau. Hùng đếm trong túi của mình có nhiều hơn 4 viên bị.
Để tham gia trò chơi bắn bi của lớp, mỗi bạn cần chuẩn bị cho mình ít nhất 18 viên bị, nhưng Hùng lại không đủ.
Thấy vậy, Quân liền lấy 1 tá bi của mình đưa cho Hùng để hai bạn được tham gia cùng nhau, nhưng Hùng vẫn không đủ số viên bị để
tham gia trò chơi. Biết 1 tá bi = 12 viên bị.
Vậy số viên bi mà Hùng có ban đầu là .........viên.