1. Yes, I have contributed to charity by donating money
2. To help people in my community, I can engage in volunteer work, support local initiatives, and participate in community projects
3. Some benefits of volunteering activities include personal fulfillment, skill development, building a sense of community, and making a positive impact on others' lives
4. The most useful invention in my house is the refrigerator, as it helps preserve food and keeps it fresh for a longer time
5. I use my mobile phone for various purposes, including communication, browsing the internet, taking photos, and managing my schedule
6. Yes, the Internet is important in my daily life for tasks such as information gathering, communication, work, and entertainment

Volunteering activities help many teenagers find their sense of purpose in life

The internet enables people to connect globally since its invention

I haven't gone kayaking for 3 years

The doctor advised me to spend more time in nature

The modern art collection will be exhibited at the museum 

A = {x|xϵ N và x ≤ 7}

A = {0, 1, 2, 3, 4, 5, 6, 7}

B = {x|xϵƯ(21) và x > 0}

B = {1, 3, 7, 21}

=>  A ∩ B = {1, 3, 7}

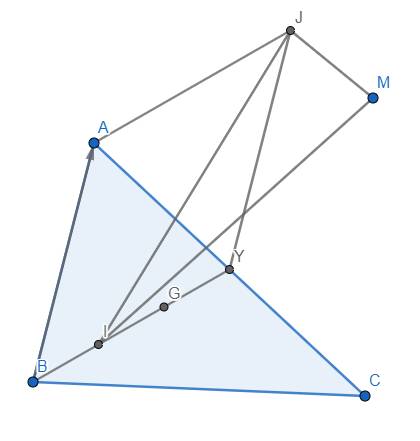
Gọi \(I\) là tâm tỉ cự của 3 điểm A, B, C ứng với bộ \(\left(1,4,1\right)\).

Khi đó: \(\overrightarrow{IA}+4\overrightarrow{IB}+\overrightarrow{IC}=\overrightarrow{0}\). Gọi Y là trung điểm AC thì \(4\overrightarrow{IB}+2\overrightarrow{IY}=\overrightarrow{0}\)  

\(\Leftrightarrow\overrightarrow{IY}=-2\overrightarrow{IB}\)

Từ đó dễ dàng xác định được vị trí của I là điểm nằm trên cạnh BY sao cho \(IY=2IB\)

 Gọi \(J\) là tâm tỉ cự của 3 điểm A, B, C ứng với bộ \(\left(9,-6,3\right)\). Khi đó \(9\overrightarrow{JA}-6\overrightarrow{JB}+3\overrightarrow{JC}=\overrightarrow{0}\)

\(\Leftrightarrow3\left(\overrightarrow{JA}+\overrightarrow{JC}\right)+6\left(\overrightarrow{JA}-\overrightarrow{JB}\right)=\overrightarrow{0}\)

\(\Leftrightarrow6\overrightarrow{JY}+6\overrightarrow{BA}=\overrightarrow{0}\)

\(\Leftrightarrow\overrightarrow{JY}=\overrightarrow{AB}\)

Vậy ta thấy J là điểm sao cho tứ giác ABYJ là hình hình hành.

Ta có \(\left|\overrightarrow{MA}+4\overrightarrow{MB}+\overrightarrow{MC}\right|+3\left|3\overrightarrow{MA}-2\overrightarrow{MB}+\overrightarrow{MC}\right|\)

\(=\left|\overrightarrow{MI}+\overrightarrow{IA}+4\left(\overrightarrow{MI}+\overrightarrow{IB}\right)+\overrightarrow{MI}+\overrightarrow{IC}\right|+\left|9\left(\overrightarrow{MJ}+\overrightarrow{JA}\right)-6\left(\overrightarrow{MJ}+\overrightarrow{JB}\right)+3\left(\overrightarrow{MJ}+\overrightarrow{JC}\right)\right|\)

\(=\left|6\overrightarrow{MI}\right|+\left|6\overrightarrow{MJ}\right|\)

\(=6\left(MI+MJ\right)\)

 Vậy ta cần tìm M để \(MI+MJ\) đạt GTNN. Ta thấy \(MI+MJ\ge IJ=const\). Dấu "=" xảy ra \(\Leftrightarrow\) M nằm trên đoạn thẳng IJ.