các đồng chí giải hộ với 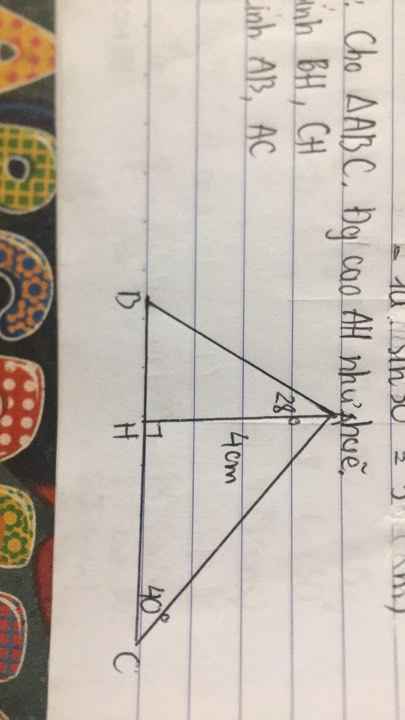
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Gọi n là hóa trị cao nhất của kim loại Z
\(Z+\dfrac{n}{2}Cl_2\underrightarrow{t^o}ZCl_n\)
\(n_Z=\dfrac{2,275}{Z}\)
\(m_{ZCl_n}=\dfrac{2,275}{Z}.\left(Z+35,5n\right)=\dfrac{2,275Z+80,7625n}{Z}=4,76\)
Với n = 2 => Z = 65
Vậy kim loại cần tìm là Zn (kẽm)

ĐKXĐ: \(\left\{{}\begin{matrix}x>=0\\x\ne4\end{matrix}\right.\)
\(P=\left(\dfrac{2}{x-4}+\dfrac{1}{\sqrt{x}+2}\right):\dfrac{1}{\sqrt{x}+2}\)
\(=\dfrac{2+\sqrt{x}-2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\cdot\dfrac{\sqrt{x}+2}{1}=\dfrac{\sqrt{x}}{\sqrt{x}-2}\)
Để P=3/2 thì \(\dfrac{\sqrt{x}}{\sqrt{x}-2}=\dfrac{3}{2}\)
=>\(3\left(\sqrt{x}-2\right)=2\sqrt{x}\)
=>\(3\sqrt{x}-2\sqrt{x}=6\)
=>\(\sqrt{x}=6\)
=>x=36(nhận)

a: 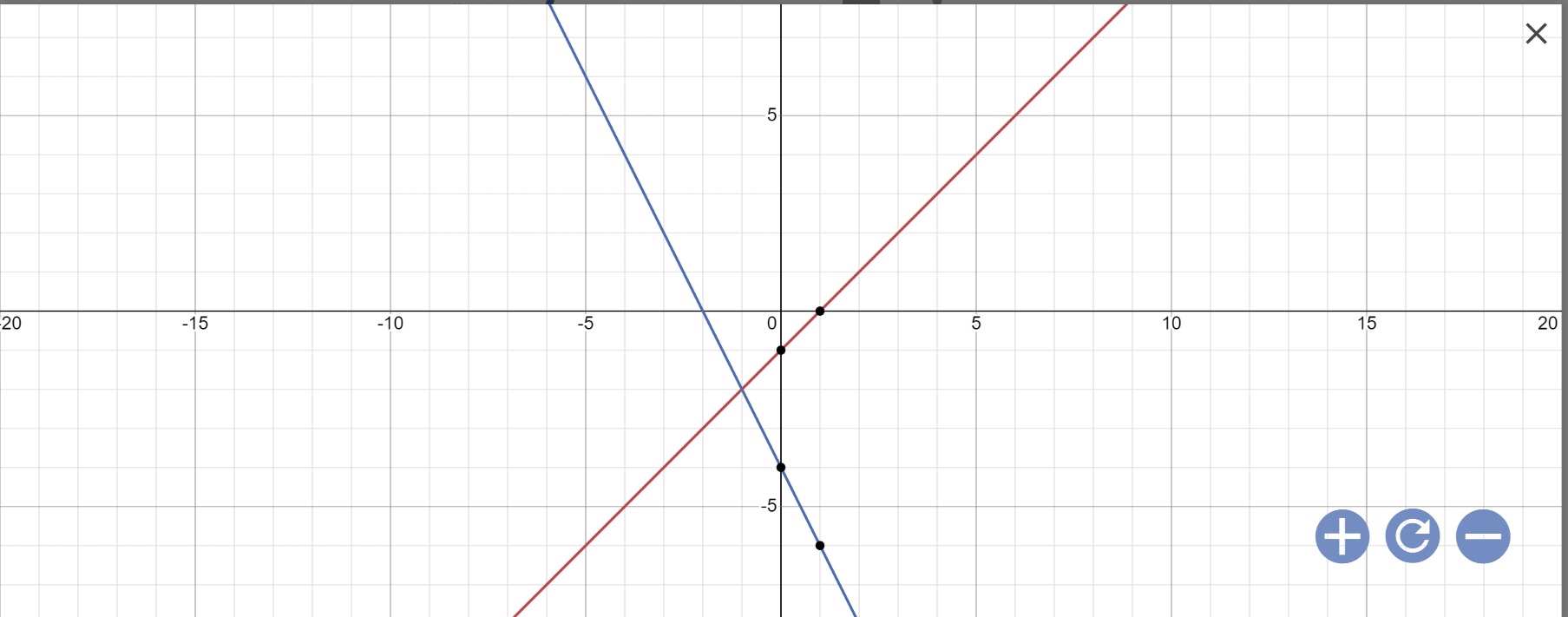
b: Phương trình hoành độ giao điểm là:
-2x-4=x-1
=>-2x-x=-1+4
=>-3x=3
=>x=-1
Thay x=-1 vào y=x-1, ta được:
y=-1-1=-2
Vậy: Tọa độ giao điểm là A(-1;-2)

a.
\(\Leftrightarrow\left\{{}\begin{matrix}\left(2x-3y\right)\left(2x-y\right)=0\\6x^2+7xy-5y^2=0\end{matrix}\right.\)
TH1: \(2x-3y=0\Rightarrow y=\dfrac{2}{3}x\) thay vào pt dưới
\(\Rightarrow6x^2+7x.\left(\dfrac{2}{3}x\right)-5\left(\dfrac{2}{3}x\right)^2=0\)
\(\Leftrightarrow\dfrac{76}{9}x^2=0\Rightarrow x=0\Rightarrow y=0\)
TH2: \(2x-y=0\Rightarrow y=2x\)
Tương tự ta cũng được \(x=0;y=0\)
Vậy hệ có nghiệm duy nhất \(\left(x;y\right)=\left(0;0\right)\)
b.
\(\Leftrightarrow\left\{{}\begin{matrix}13x^2-39xy+13y^2=-13\\2x^2+xy+3y^2=13\end{matrix}\right.\)
Cộng vế với vế
\(\Rightarrow15x^2-38xy+16y^2=0\)
\(\Leftrightarrow\left(x-2y\right)\left(15x-8y\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=2y\\x=\dfrac{8}{15}y\end{matrix}\right.\)
Thay vào pt đầu:
- Với \(x=2y\Rightarrow4y^2-6y^2+y^2=-1\)
\(\Rightarrow y^2=1\Rightarrow\left[{}\begin{matrix}y=1\Rightarrow x=2\\y=-1\Rightarrow x=-2\end{matrix}\right.\)
- Với \(x=\dfrac{8}{15}y\)
\(\Rightarrow\left(\dfrac{8}{15}y\right)^2-3\left(\dfrac{8}{15}y\right).y+y^2=-1\)
\(\Leftrightarrow-\dfrac{71}{225}y^2=-1\Rightarrow y^2=\dfrac{225}{71}\)
\(\Rightarrow\left[{}\begin{matrix}y=\dfrac{15}{\sqrt{71}}\Rightarrow x=\dfrac{8}{\sqrt{71}}\\y=-\dfrac{15}{\sqrt{71}}\Rightarrow x=-\dfrac{8}{\sqrt{71}}\end{matrix}\right.\)

- Kiều Nguyệt Nga là một cô gái kiêu sa, dịu dàng, hiểu biết, và lòng trọng ân nghĩa. - Nhân vật này thể hiện lòng biết ơn, lòng chân thành, và tư tưởng đền ơn báo nghĩa. - Kiều Nguyệt Nga là hình mẫu của người con gái truyền thống, nết na, hiền thục, và có hiểu biết.

Xét ΔABC vuông tại A có \(sinB=\dfrac{AC}{BC}\)
=>\(\dfrac{6}{BC}=sin30=\dfrac{1}{2}\)
=>\(BC=6\cdot2=12\left(cm\right)\)

\(\left|A+B\right|< =\left|A\right|+\left|B\right|\)
=>\(\left(\left|A+B\right|\right)^2< =\left(\left|A\right|+\left|B\right|\right)^2\)
=>\(A^2+B^2+2AB< =A^2+B^2+2\left|AB\right|\)
=>2AB<=2|AB|
=>AB<=|AB|(luôn đúng)
Dấu '=' xảy ra khi AB>=0

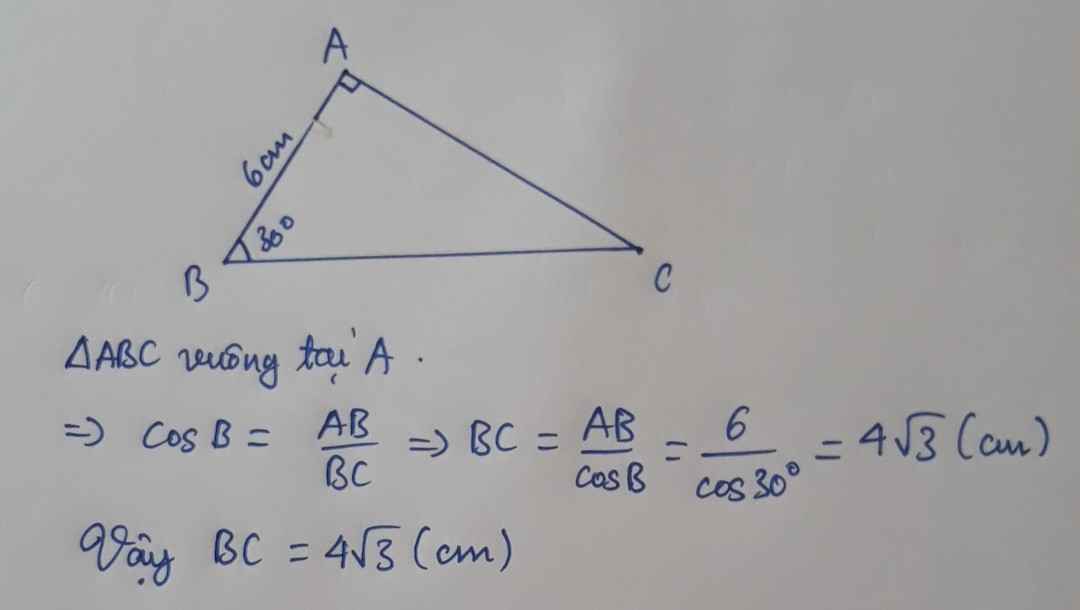
xét ΔABC vuông tại A có \(cosB=\dfrac{AB}{BC}\)
=>\(\dfrac{6}{BC}=cos30=\dfrac{\sqrt{3}}{2}\)
=>\(BC=6\cdot\dfrac{2}{\sqrt{3}}=4\sqrt{3}\left(cm\right)\)

1 It is difficult for her to go on a trip abroad now
2 I wish I had more time to take my friends to beauty sports in my hometown
3 At school, English is compulsory while French is optional
4 Although we are away from each other, we still keep in touch
5 Lan wishes she would spend her vacation in Singapore someday
6 Malaysia is divided into two regions, known as West Malaysia and East Malaysia
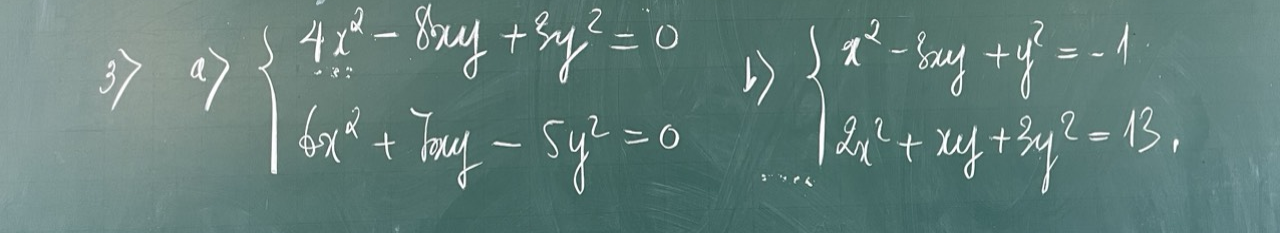
Xét ΔAHB vuông tại H có \(tanBAH=\dfrac{BH}{AH}\)
=>\(BH=AH\cdot tanBAH=4\cdot tan28\simeq2,13\left(cm\right)\)
Xét ΔAHC vuông tại H có
\(tanC=\dfrac{AH}{HC}\)
=>\(HC=\dfrac{AH}{tanC}=\dfrac{4}{tan40}\simeq4,77\left(cm\right)\)
ΔAHB vuông tại H
=>\(AH^2+HB^2=AB^2\)
=>\(AB=\sqrt{AH^2+HB^2}\simeq4,53\left(cm\right)\)
ΔAHC vuông tại H
=>\(AH^2+HC^2+AC^2\)
=>\(AC=\sqrt{AH^2+HC^2}\simeq6,23\left(cm\right)\)