TÍNH a=?
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Trả lời:
a) √(9a) + √(25a) - √(49a)
=3√a +5√a -7√a
=√a.(3+5-7)
=√a.1=√a
b) √75 + √48 - √300
=√25.√3 +√16.√3 -√100.√3
=√3.(√25 +√16-√100)
=√3.(5 +4 -10)
=√3.(-1) =-√3
c) (2√3 + √5) √3 - √60
=2.√3.√3 +√5.√3 -√4.√15
=2.3 +√15 -2.√15
=6 +√15.(1-2)
=6 +√15.(-1)
=6 -√15
HT
a) √(9a) + √(25a) - √(49a) với a ≥ 0
\(a*căn bậc hai(9*a) + căn bậc hai(25*a) - căn bậc hai(49*a)\)
b) √75 + √48 - √300
căn bậc hai(75) + căn bậc hai(48) - căn bậc hai(300)

cp cắt (o) tại e tức e thuộc (O) rồi xong lại be cắt (o) tại m ??

Áp dụng định lí Pytago tam giác DHF vuông tại H
\(DF=\sqrt{FH^2+DF^2}=\sqrt{9+9}=\sqrt{18}=3\sqrt{2}\)cm
Xét tam giác ABC vuông tại A, đường cao AH
* Áp dụng hệ thức : \(DH^2=FH.HE\Rightarrow HF=\frac{DH^2}{HE}=\frac{9}{3}=3\)cm
-> FE = 2FH = 6 cm
Theo định lí Pytago tam giác DÈF vuông tại D
\(DE=\sqrt{FE^2-DF^2}=\sqrt{36-18}=\sqrt{18}=3\sqrt{2}\)cm


\(\frac{1-2sin^2x}{cosx-sinx}=\frac{sin^2x+cos^2x-2sin^2x}{cosx-sinx}\)
\(=\frac{cos^2x-sin^2x}{cosx-sinx}=\frac{\left(cosx+sinx\right)\left(cosx-sinx\right)}{cosx-sinx}=cosx+sinx\)
\(\frac{1-\sin^2x-\sin^2x}{\cos x-\sin x}=\frac{\cos^2x-\sin^2x}{\cos x-\sin x}=\frac{\left(\cos x-\sin x\right)\left(\cos x+\sin x\right)}{\cos x-\sin x}\cos=\cos x+\sin x\)
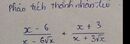
\(\sqrt{16+6\sqrt{7}}=a+\sqrt{7}\)
\(\Leftrightarrow\sqrt{9+2.3.\sqrt{7}+7}=a+\sqrt{7}\)
\(\Leftrightarrow\sqrt{\left(3+\sqrt{7}\right)^2}=a+\sqrt{7}\)
\(\Leftrightarrow3+\sqrt{7}=a+\sqrt{7}\)
\(\Leftrightarrow a=3\)