1 người cao 1,5m. mắt cách đỉnh đầu 15cm, đứng cách gương 2m. Vẽ hình và xác định chiều cao tối thiểu của gương và gương cách mặt đất bao nhiêu để người đó nhìn thấy toàn bộ ảnh của mình
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
NV
Nguyễn Việt Lâm
Giáo viên
9 tháng 1 2024
\(H=\left(3x-6\right)^2-3\left|2x-4\right|+2023\)
\(=\left(3x-6\right)^2-2\left|3x-6\right|+2023\)
\(=\left(3x-6\right)^2-2\left|3x-6\right|+1+2022\)
\(=\left(\left|3x-6\right|-1\right)^2+2022\)
Do \(\left(\left|3x-6\right|-1\right)^2\ge0;\forall x\)
\(\Rightarrow H\ge2022\)
\(\Rightarrow H_{min}=2022\) khi \(\left|3x-6\right|-1=0\Rightarrow x=\left\{\dfrac{7}{3};\dfrac{5}{3}\right\}\)
NV
1
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
8 tháng 1 2024
A = \(\dfrac{2}{3.5}\) + \(\dfrac{2}{5.7}\) + \(\dfrac{2}{7.9}\) + ... + \(\dfrac{2}{19.21}\)
A = \(\dfrac{1}{3}\) - \(\dfrac{1}{5}\) + \(\dfrac{1}{5}\) - \(\dfrac{1}{7}\) + \(\dfrac{1}{7}\) - \(\dfrac{1}{9}\) + ... + \(\dfrac{1}{19}\) - \(\dfrac{1}{21}\)
A = \(\dfrac{1}{3}\) - \(\dfrac{1}{21}\)
A = \(\dfrac{2}{7}\)
AH
Akai Haruma
Giáo viên
9 tháng 1 2024
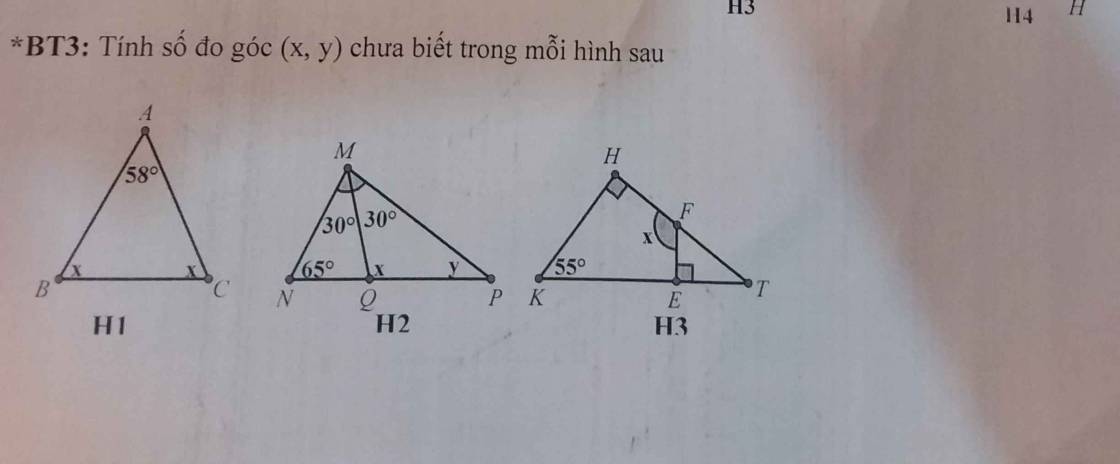
Lời giải:
$x+x+58^0=180^0$ (tổng 3 góc trong tam giác)
$\Rightarrow 2x=180^0-58^0=122^0$
$\Rightarrow x=61^0$
--------------------
$x=\widehat{NMQ}+\widehat{MNQ}=30^0+65^0=95^0$
$y=180^0-30^0-x=150^0-95^0=55^0$
--------------------
$x=360^0-55^0-90^0-90^0=125^0$
