
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Bài 1:
a) Để căn thức có nghĩa thì:
\(x+1\ge0\Leftrightarrow x\ge-1\)
b) Để căn thức có nghĩa thì:
\(3x-8\ge0\Leftrightarrow3x\ge8\Leftrightarrow x\ge\dfrac{8}{3}\)
c) Để căn thức có nghĩa thì:
\(2x^2+3>0\)
Mà điều này luôn đúng nên căn thức có nghĩa khi x ∈ R
d) Để căn thức có nghĩa thì:
\(16-x^2\ge0\Leftrightarrow\left(4-x\right)\left(4+x\right)\ge0\Leftrightarrow-4\le x\le4\)
Câu 1: B
Câu 2: D
Câu 3: ĐKXĐ: 2x+5>=0
=>2x>=-5
=>\(x>=-\dfrac{5}{2}\)
=>Chọn C
Câu 4: ĐKXĐ: 3-4x>=0
=>-4x>=-3
=>4x<=3
=>\(x< =\dfrac{3}{4}\)
=>Chọn D
Câu 5: \(\sqrt{7}-\sqrt{\left(1-\sqrt{7}\right)^2}\)
\(=\sqrt{7}-\left|1-\sqrt{7}\right|\)
\(=\sqrt{7}-\sqrt{7}+1=1\)
=>Chọn B
Câu 6: \(\sqrt{x^2-6x+9}=\sqrt{\left(x-3\right)^2}=\left|x-3\right|\)
x>=3 nên x-3>=0
=>\(\sqrt{x^2-6x+9}=\left|x-3\right|=x-3\)
=>Chọn B
Câu 7: \(\sqrt{25+x^2+10x}=\sqrt{x^2+10x+25}=\sqrt{\left(x+5\right)^2}=\left|x+5\right|\)
x<-6
=>x+6<0
mà x+5<x+6
nên x+5<0
=>\(\sqrt{25+x^2+10x}=-\left(x+5\right)\)
=>Chọn D
Câu 8: \(M=2x-\sqrt{x^4+2x^2+1}\)
\(=2x-\sqrt{\left(x^2+1\right)^2}\)
\(=2x-\left(x^2+1\right)=-\left(x^2-2x+1\right)=-\left(x-1\right)^2\)
Thay x=11 vào M, ta được:
\(M=-\left(11-1\right)^2=-10^2=-100\)
=>Chọn A
Câu 9: \(\sqrt{x-7}=2\)
=>\(x-7=2^2=4\)
=>x=4+7=11
=>Chọn C
Câu 10: \(\sqrt{a^4b^2}=\sqrt{a^4}\cdot\sqrt{b^2}=a^2\cdot\left|b\right|\)
=>Chọn D
Câu 11: \(\dfrac{1}{2+\sqrt{3}}+\dfrac{1}{2-\sqrt{3}}\)
\(=\dfrac{2-\sqrt{3}+2+\sqrt{3}}{\left(2+\sqrt{3}\right)\left(2-\sqrt{3}\right)}\)
\(=\dfrac{4}{4-3}=4\)
=>Chọn C
Câu 12: A

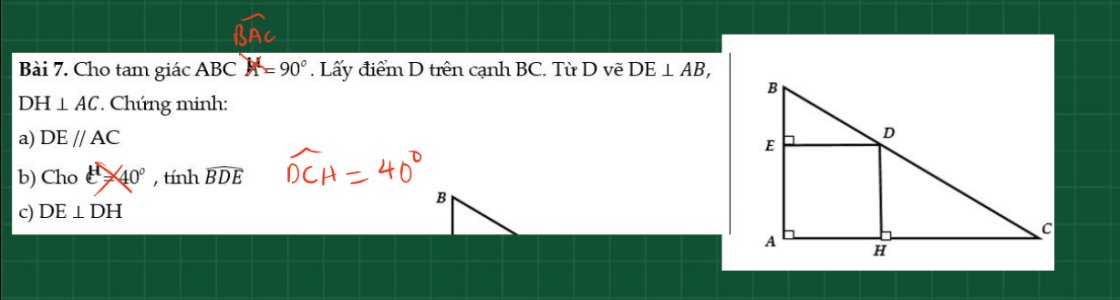
a, Ta có DE vuông AB
AH vuông AB
=> DE // AH
b, Ta có DE // AH => ^BDE = ^ACB ( 2 góc đồng vị )
=> ^BDE = ^DCH = 400
c, Ta có DH vuông AC
AB vuông AC
=> DH // AB
Ta có DH // AB; ED//AH ; ^EAH = ^AED = ^AHD = 900
Vậy tứ giác AEDH là hình vuông
=> DE vuông DH

Số xe cửa hàng nhập về là:
\(80:32\%=250\) (chiếc)
Số xe cửa hàng còn lại là:
`250 - 80 =170` (chiếc)
ĐS: ...

\(a)2^3-50:25+13\cdot7=8-2+91\\ =6+91\\ =97\\ b)60-\left[120-\left(42-33\right)\cdot2\right]\\ =60-\left(120-9\cdot2\right)\\ =60-\left(120-18\right)\\ =60-102\\ =-42\\ c)3^{17}:3^{15}+8\cdot3\\ =3^{17-15}+24\\ =3^2+24\\ =9+24\\ =33\\ d)12:\left\{390:\left[500-\left(125+35\cdot7\right)\right]\right\}\\ =12:\left\{390:\left[500-\left(125+245\right)\right]\right\}\\ =12:\left[390:\left(500-370\right)\right]\\ =12:\left(390:130\right)\\ =12:3=4\)
e: \(72^3\cdot49-72^2\cdot9\)
\(=72^2\left(72\cdot49-9\right)\)
\(=5184\cdot3519=18242496\)
f: \(\dfrac{2^3+2^4+2^5}{7^2}=\dfrac{2^3\left(1+2+2^2\right)}{7^2}=\dfrac{8\cdot7}{49}=\dfrac{8}{7}\)
g: \(\dfrac{15^{22}\cdot7^{18}}{7^{20}\cdot15^{21}}=\dfrac{15^{22}}{15^{21}}\cdot\dfrac{7^{18}}{7^{20}}=\dfrac{15}{7^2}=\dfrac{15}{49}\)

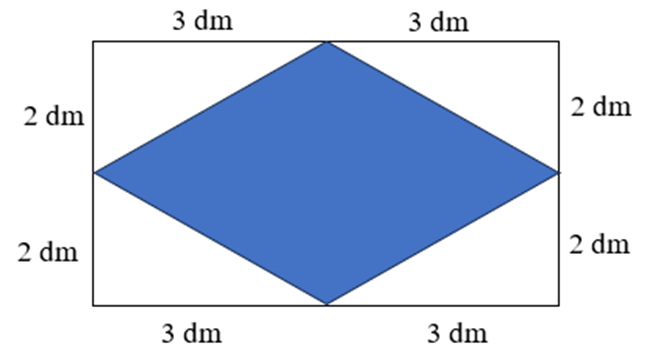
Diện tích của các hình là:
\(\left(2+2\right)\times\left(3+3\right)=24\left(dm^2\right)\)
Diện tích phần màu trắng là:
\(\dfrac{1}{2}\times2\times3+\dfrac{1}{2}\times2\times3+\dfrac{1}{2}\times2\times3+\dfrac{1}{2}\times2\times3=12\left(dm^2\right)\)
Diện tích phần tô màu là:
\(24-12=12\left(dm^2\right)\)
ĐS: ...

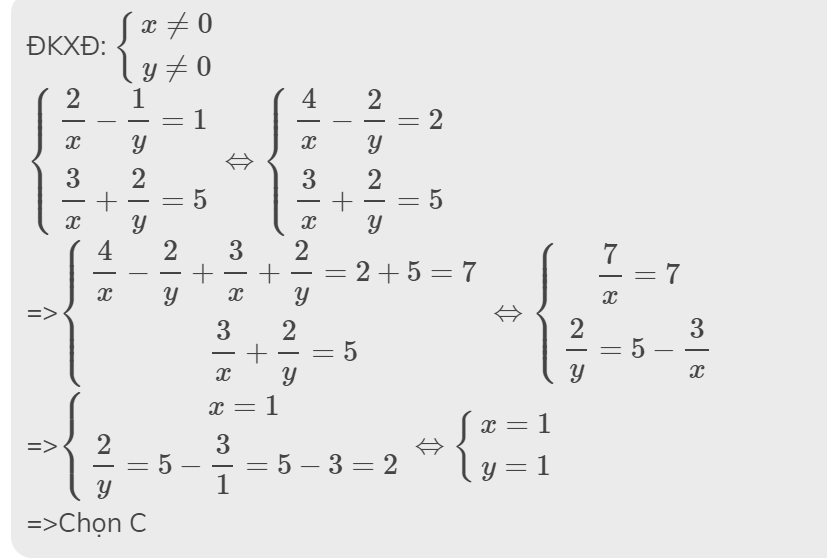
ĐKXĐ: \(\left\{{}\begin{matrix}x\ne0\\y\ne0\end{matrix}\right.\)
\(\left\{{}\begin{matrix}\dfrac{2}{x}-\dfrac{1}{y}=1\\\dfrac{3}{x}+\dfrac{2}{y}=5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\dfrac{4}{x}-\dfrac{2}{y}=2\\\dfrac{3}{x}+\dfrac{2}{y}=5\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\dfrac{4}{x}-\dfrac{2}{y}+\dfrac{3}{x}+\dfrac{2}{y}=2+5=7\\\dfrac{3}{x}+\dfrac{2}{y}=5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\dfrac{7}{x}=7\\\dfrac{2}{y}=5-\dfrac{3}{x}\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=1\\\dfrac{2}{y}=5-\dfrac{3}{1}=5-3=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=1\end{matrix}\right.\)
=>Chọn C

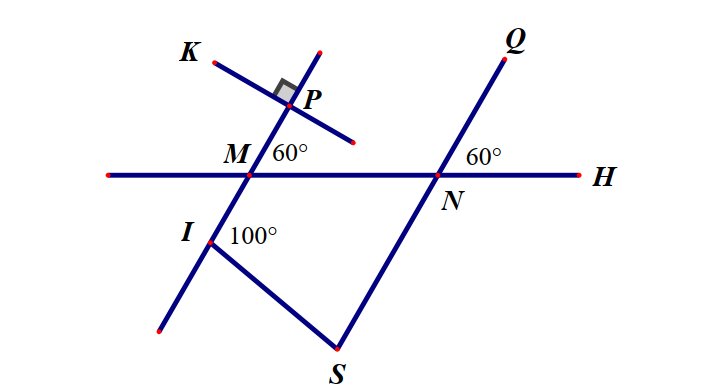
a: ta có: \(\widehat{MNS}=\widehat{HNQ}\)(hai góc đối đỉnh)
mà \(\widehat{HNQ}=60^0\)
nên \(\widehat{MNS}=60^0\)
b: Ta có: \(\widehat{QNH}=\widehat{PMN}\left(=60^0\right)\)
mà hai góc này là hai góc ở vị trí đồng vị
nên PI//QS
=>MP//NQ
c: ta có: MP//NQ
KP\(\perp\)MP
Do đó: KP\(\perp\)QN
d: ta có: MI//SN
=>\(\widehat{MIS}+\widehat{S}=180^0\)(hai góc trong cùng phía)
=>\(\widehat{S}+100^0=180^0\)
=>\(\widehat{S}=80^0\)

ĐKXĐ: \(\left\{{}\begin{matrix}x>=0\\y>=0\end{matrix}\right.\)
\(\left\{{}\begin{matrix}2\sqrt{x}+\sqrt{y}=5\\3\sqrt{x}-\sqrt{y}=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}2\sqrt{x}+\sqrt{y}+3\sqrt{x}-\sqrt{y}=5+1\\2\sqrt{x}+\sqrt{y}=5\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}5\sqrt{x}=6\\\sqrt{y}=5-2\sqrt{x}\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\sqrt{x}=\dfrac{6}{5}\\\sqrt{y}=5-2\cdot\dfrac{6}{5}=5-\dfrac{12}{5}=\dfrac{13}{5}\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=\dfrac{36}{25}\\y=\dfrac{169}{25}\end{matrix}\right.\)
=>Chọn B
\(\left\{{}\begin{matrix}2\sqrt{x}+\sqrt{y}=5\\3\sqrt{x}-\sqrt{y}=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}5\sqrt{x}=6\\2\sqrt{x}+\sqrt{y}=5\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}\sqrt{x}=\dfrac{6}{5}\\\dfrac{12}{5}+\sqrt{y}=5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{36}{25}\\\sqrt{y}=5-\dfrac{12}{5}=\dfrac{13}{5}\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{36}{25}\\y=\left(\dfrac{13}{5}\right)^2=\dfrac{169}{25}\end{matrix}\right.\)
=> Chọn B


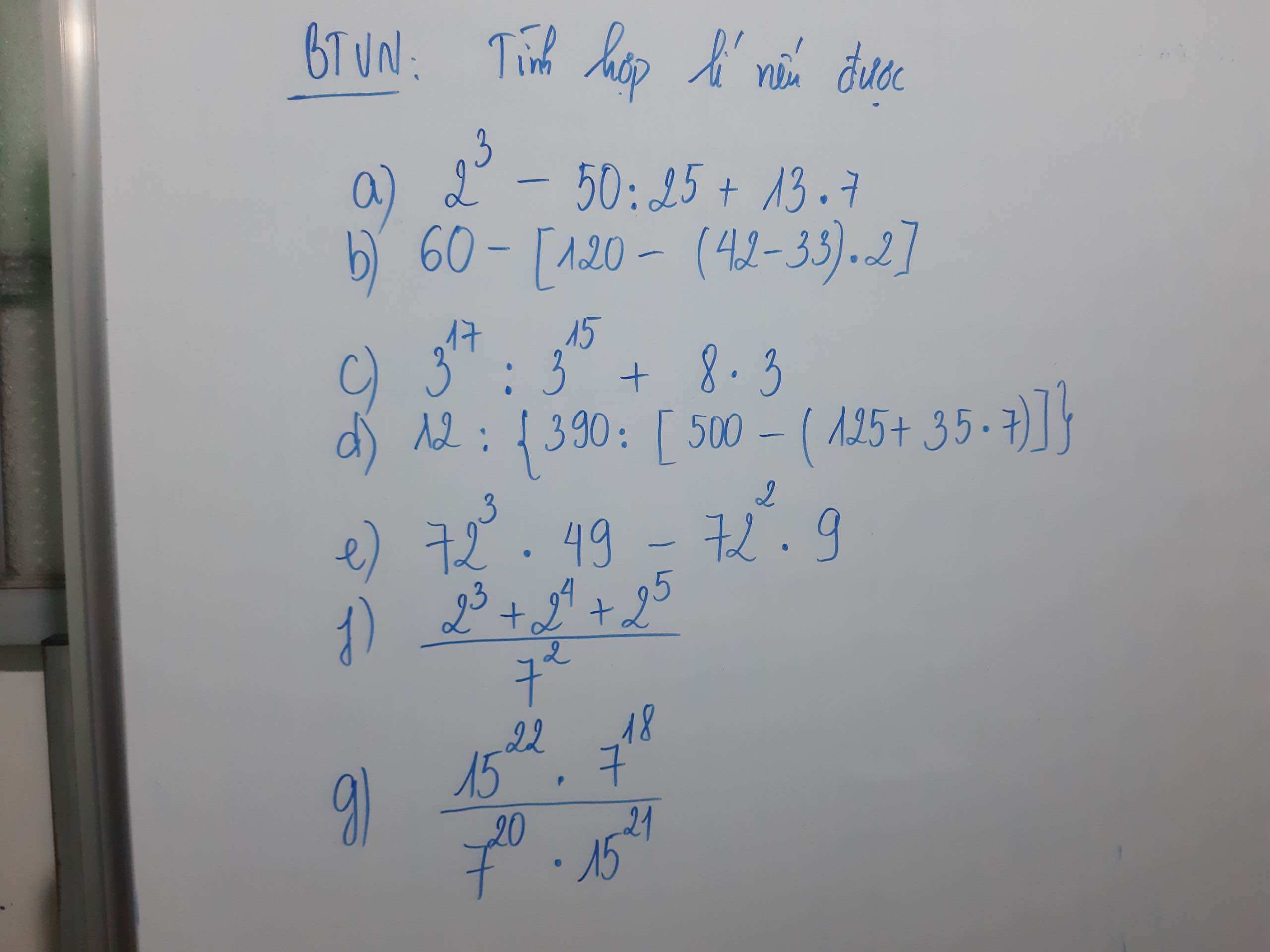
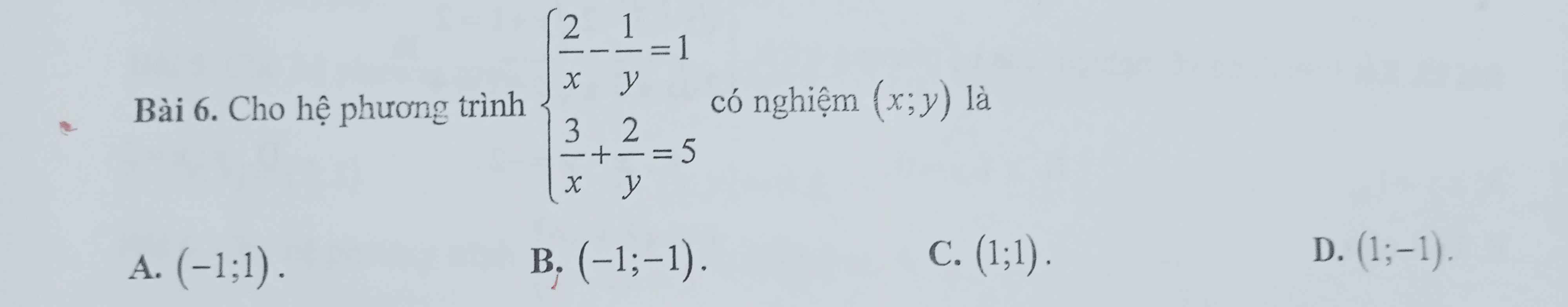
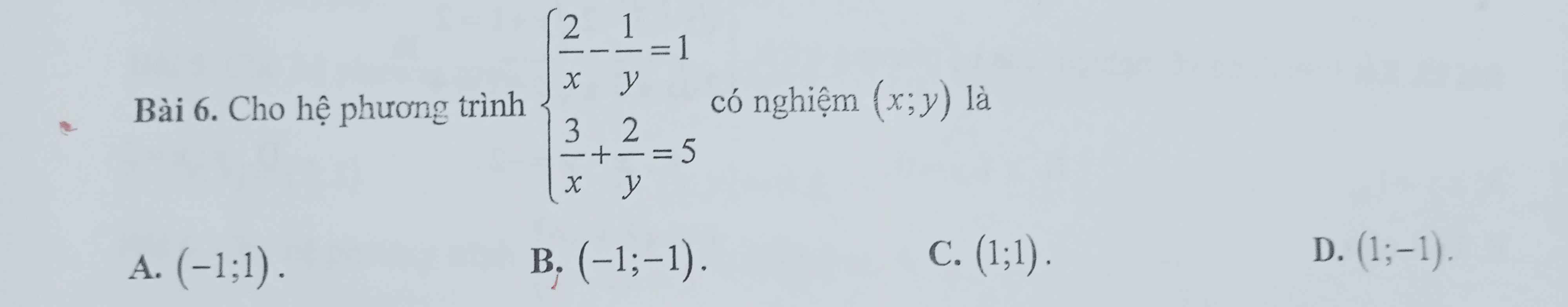
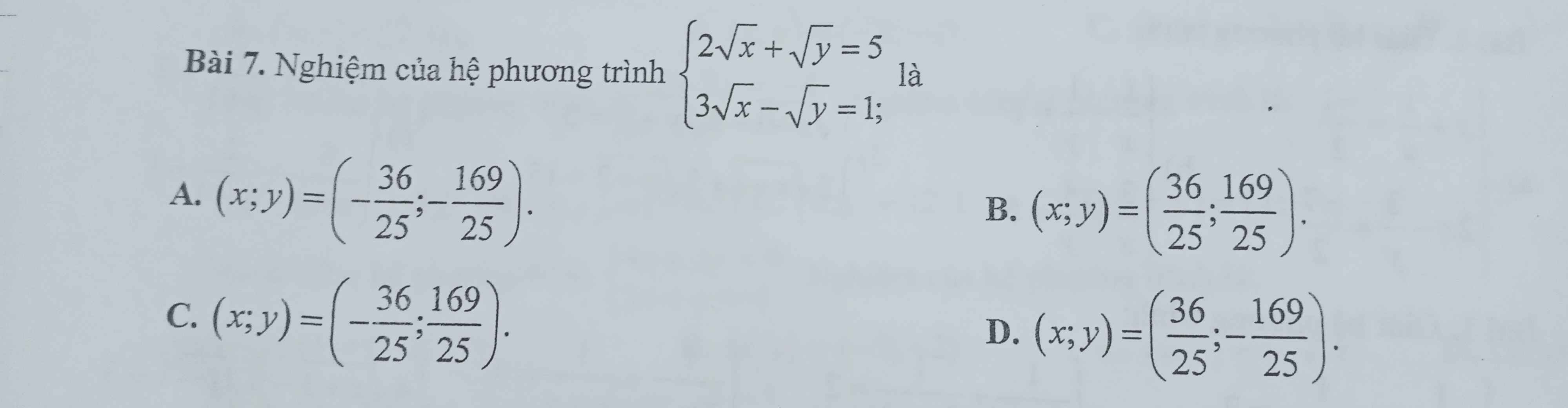
Bài 3:
\(a)\left(x+2\right)\left(x+3\right)-\left(x-2\right)\left(x+5\right)=0\\ \Leftrightarrow x^2+3x+2x+6-\left(x^2+5x-2x-10\right)=0\\ \Leftrightarrow x^2+5x+6-x^2-3x+10=0\\ \Leftrightarrow2x+16=0\\ \Leftrightarrow2x=-16\\ \Leftrightarrow x=-\dfrac{16}{2}=-8\\ b)\left(x-3\right)\left(x-2\right)-\left(x+1\right)\left(x-5\right)=0\\ \Leftrightarrow\left(x^2-2x-3x+6\right)-\left(x^2-5x+x-5\right)=0\\ \Leftrightarrow x^2-5x+6-x^2+4x+5=0\\ \Leftrightarrow-x+11=0\\ \Leftrightarrow x=11\\ c)x\left(2x-5\right)-2x\left(x-6\right)=42\\ \Leftrightarrow2x^2-5x-2x^2+12x=42\\ \Leftrightarrow7x=42\\ \Leftrightarrow x=\dfrac{42}{7}\\ \Leftrightarrow x=6\\ d)\left(x-1\right)\left(2x+3\right)-2x\left(x-1\right)=0\\ \Leftrightarrow\left(x-1\right)\left(2x+3-2x\right)=0\\ \Leftrightarrow3\left(x-1\right)=0\\ \Leftrightarrow x-1=0\\ \Leftrightarrow x=1\)
Bài 2:
b: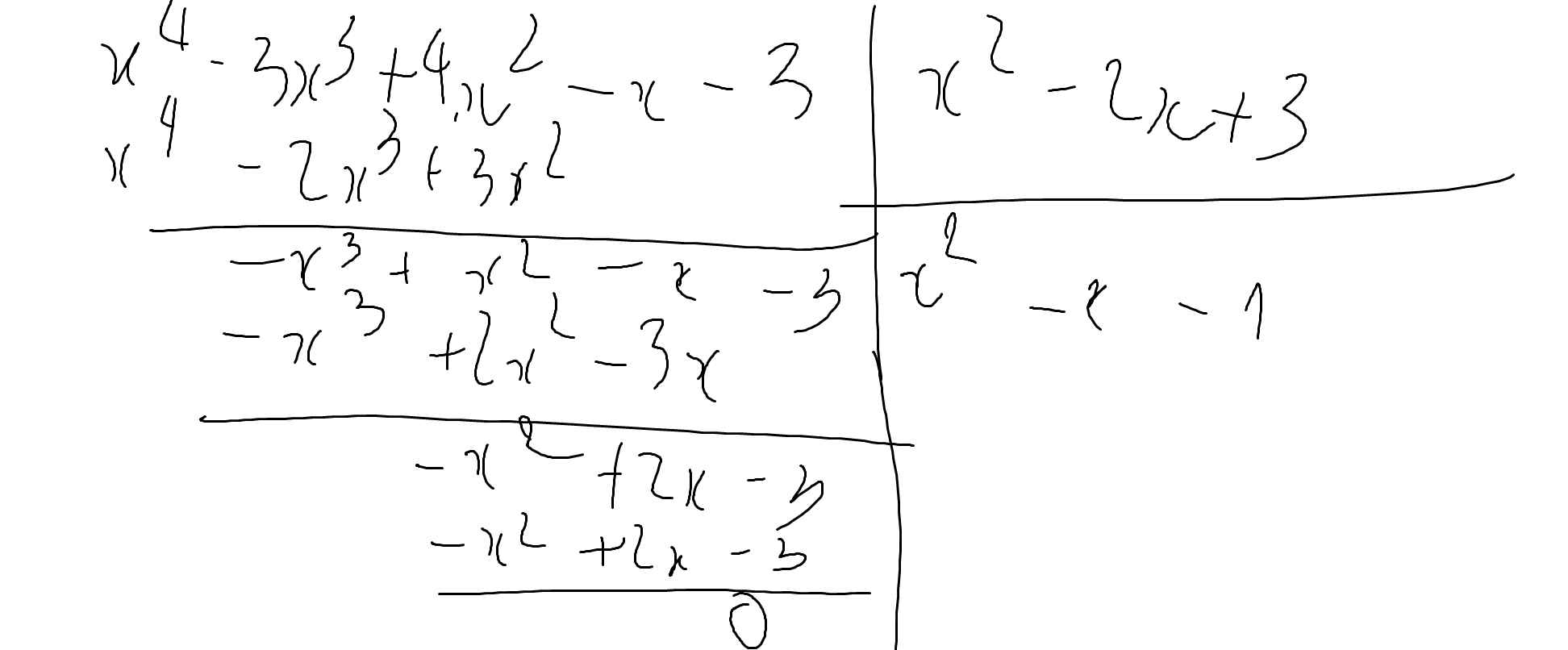
c:
d:
Bài 4:
a: \(A\left(x\right)=x^7-3x^2-x^5+x^4-x^2+2x-7\)
\(=x^7-x^5+x^4+\left(-3x^2-x^2\right)+2x-7\)
\(=x^7-x^5+x^4-4x^2+2x-7\)
\(B\left(x\right)=x-2x^2+x^4-x^5-x^7-4x^2-1\)
\(=-x^7-x^5+x^4+\left(-2x^2-4x^2\right)+x-1\)
\(=-x^7-x^5+x^4-6x^2+x-1\)
b: A(x)+B(x)
\(=x^7-x^5+x^4-4x^2+2x-7-x^7-x^5+x^4-6x^2+x-1\)
\(=-2x^5-10x^2+3x-8\)
A(x)-B(x)
\(=x^7-x^5+x^4-4x^2+2x-7+x^7+x^5-x^4+6x^2-x+1\)
\(=2x^7+2x^2+x-6\)
c: C(x)=A(x)+B(x
=>\(C\left(x\right)=-2x^5-10x^2+3x-8\)
Thay x=-1 vào C(x), ta được:
\(C\left(-1\right)=-2\cdot\left(-1\right)^5-10\cdot\left(-1\right)^2+3\cdot\left(-1\right)-8\)
=2-10-3-8
=-1-10-8=-19