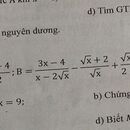Tính A=\(x^3+y^3-3\left(x+y\right)\) biết rằng:
\(x=\sqrt[3]{3+2\sqrt{2}}+\sqrt[3]{3-2\sqrt{2}}\); \(y=\sqrt[3]{17+12\sqrt{2}}+\sqrt[3]{17-12\sqrt{2}}\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
LM
1

KN
3 tháng 12 2020
ĐK : x\(\ge\)- 5
\(x^2-7x=6\sqrt{x+5}-30\)
<=> \(x^2-7x+30-6\sqrt{x+5}=0\)
<=> \(\left(x^2-8x+16\right)+\left(x+5-6\sqrt{x+5}+9\right)=0\)
<=> \(\left(x-4\right)^2+\left(\sqrt{x+5}-3\right)^2=0\)
<=> \(\orbr{\begin{cases}x-4=0\\\sqrt{x+5}-3=0\end{cases}}\)<=> x = 4

28 tháng 12 2019
\(\left(a-\sqrt{2011}\right)\left(b+\sqrt{2011}\right)=14\)
\(\Leftrightarrow ab+\sqrt{2011}\left(a-b\right)=2025\)
Có: a,b nguyên => a-b nguyên
=> VP=VT <=> \(\sqrt{2011}\left(a-b\right)\)nguyên
=> a-b=0 <=> a=b
=> pt <=> a^2=2025
Làm nốt nhé.

MP
0